Difference between revisions of "Kustaanheimo-Stiefel transformation"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Kustaanheimo–Stiefel transformation to Kustaanheimo-Stiefel transformation: ascii title) |
(No difference)
| |
Revision as of 18:53, 24 March 2012
A particular case of the surjective application  defined by
defined by
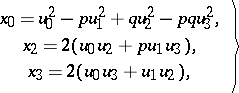 | (a1) |
subject to the constraint  with
with
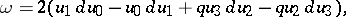 | (a2) |
where the parameters  and
and  can take the values
can take the values  . The transformation introduced by P. Kustaanheimo and E. Stiefel [a1] corresponds to
. The transformation introduced by P. Kustaanheimo and E. Stiefel [a1] corresponds to  , while the cases
, while the cases  [a2] and
[a2] and 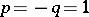 (or
(or  ) [a3] correspond to two other (inequivalent) transformations.
) [a3] correspond to two other (inequivalent) transformations.
There are several ways of introducing these three transformations. For instance, the Kustaanheimo–Stiefel mapping is inherent to the Cartan spinor theory, since (a1), with 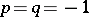 , can be rewritten as
, can be rewritten as  ,
,  and
and  , where the
, where the  (with
(with  ) are the Pauli matrices and
) are the Pauli matrices and 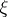 is a spinor of components
is a spinor of components  and
and  (
( stands for the adjoint of
stands for the adjoint of 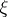 ). Furthermore, the Kustaanheimo–Stiefel transformation, along with the two other transformations, can be introduced as a particular Hurwitz transformation (see also Hurwitz transformation) in the framework of the Cayley–Dickson algebra
). Furthermore, the Kustaanheimo–Stiefel transformation, along with the two other transformations, can be introduced as a particular Hurwitz transformation (see also Hurwitz transformation) in the framework of the Cayley–Dickson algebra  . (The algebra
. (The algebra  turns out to be the algebra of ordinary (or elliptic) quaternions when
turns out to be the algebra of ordinary (or elliptic) quaternions when 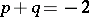 and the algebra of hyperbolic quaternions when
and the algebra of hyperbolic quaternions when 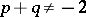 .) The main properties of the transformations (a1)–(a2) are as follows.
.) The main properties of the transformations (a1)–(a2) are as follows.
1) These transformations satisfy
 |
and
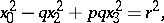 | (a3) |
where the metric  is
is  , so that
, so that
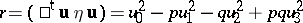 | (a4) |
( denotes a column vector, the transposed of which is the row vector
denotes a column vector, the transposed of which is the row vector 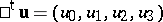 ).
).
2) Equations (a3)–(a4) show that the various mappings (a1) correspond to three types of fibrations (cf. Fibration): the celebrated Hopf fibration (on spheres)  of compact fibre
of compact fibre  for
for 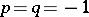 and two other fibrations (on hyperboloids), namely,
and two other fibrations (on hyperboloids), namely,  of compact fibre
of compact fibre  for
for 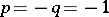 and
and  of non-compact fibre
of non-compact fibre  for
for  and
and  .
.
3) The preceding geometrical property can be made precise by transformation properties of (first- and) second-order elliptic and hyperbolic differential operators. For example, this yields (for  )
)
 |
where
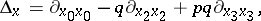 |
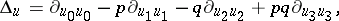 |
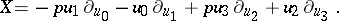 |
4) The vector field 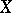 associated to the
associated to the  -form
-form 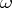 (with an action satisfying
(with an action satisfying  ) is an element of the symplectic Lie algebra
) is an element of the symplectic Lie algebra  which generates either the subalgebra
which generates either the subalgebra  or
or  , according to whether the fibre is compact or non-compact. The introduction of the (Dirac primary) constraint
, according to whether the fibre is compact or non-compact. The introduction of the (Dirac primary) constraint  in
in  produces a Lie algebra under constraint (i.e., the centralizer of
produces a Lie algebra under constraint (i.e., the centralizer of  in
in  ), which is isomorphic to
), which is isomorphic to  or
or  , depending on the compactness or non-compactness of the fibre, respectively (see [a3] and the references therein).
, depending on the compactness or non-compactness of the fibre, respectively (see [a3] and the references therein).
The applications of the transformations (a1)–(a2) range from number theory to physics (classical and quantum mechanics, gauge theories). By way of illustration, the (generalized) Hurwitz matrix corresponding to (a1)–(a2) is connected to the (generalized) problem of factoring the sum of four squared numbers, addressed by A. Hurwitz (1898) in the specific case  . In addition, for
. In addition, for 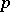 and
and 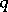 fixed, the solutions of the generalized Pythagorean equation (a3) with
fixed, the solutions of the generalized Pythagorean equation (a3) with 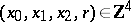 are parametrized by (a1) and (a4) with
are parametrized by (a1) and (a4) with 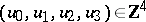 . In classical mechanics, the Kustaanheimo–Stiefel transformation is used for the regularization of the Kepler problem. In quantum mechanics (cf. also MIC-Kepler problem), the latter transformation makes it possible to transform the Schrödinger equation for the three-dimensional hydrogen atom (in an electromagnetic field) into a Schrödinger equation for a four-dimensional isotropic harmonic oscillator (with quartic and sextic anharmonic terms) subject to a constraint [a4], [a5]. Conversely, the mappings (a1)–(a2) may be used in some dimensional reduction process for converting a dynamical system in
. In classical mechanics, the Kustaanheimo–Stiefel transformation is used for the regularization of the Kepler problem. In quantum mechanics (cf. also MIC-Kepler problem), the latter transformation makes it possible to transform the Schrödinger equation for the three-dimensional hydrogen atom (in an electromagnetic field) into a Schrödinger equation for a four-dimensional isotropic harmonic oscillator (with quartic and sextic anharmonic terms) subject to a constraint [a4], [a5]. Conversely, the mappings (a1)–(a2) may be used in some dimensional reduction process for converting a dynamical system in  or
or  into a dynamical system in
into a dynamical system in  .
.
References
| [a1] | P. Kustaanheimo, E. Stiefel, "Perturbation theory of Kepler motion based on spinor regularization" J. Reine Angew. Math. , 218 (1965) pp. 204 |
| [a2] | T. Iwai, "On reduction of two degrees of freedom Hamiltonian system by an 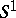 action, and action, and 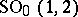 as a dynamical group" J. Math. Phys. , 26 (1985) pp. 885 as a dynamical group" J. Math. Phys. , 26 (1985) pp. 885 |
| [a3] | D. Lambert, M. Kibler, "An algebraic and geometric approach to non-bijective quadratic transformations" J. Phys. A: Math. Gen. , 21 (1988) pp. 307 |
| [a4] | M. Boiteux, "Theory of nonbijective canonical transformations in mechanics: Application to the Coulomb problem" J. Math. Phys. , 23 (1982) pp. 1311 |
| [a5] | M. Kibler, T. Négadi, "Hydrogen atom in a uniform electromagnetic field as an anharmonic oscillator" Lett. Nuovo Cim. , 39 (1984) pp. 319 |
Kustaanheimo-Stiefel transformation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kustaanheimo-Stiefel_transformation&oldid=17651