Difference between revisions of "Kuhn-Tucker conditions"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Kuhn–Tucker conditions to Kuhn-Tucker conditions: ascii title) |
(No difference)
| |
Revision as of 18:53, 24 March 2012
Let 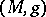 be a complete finite-dimensional Riemannian manifold, let
be a complete finite-dimensional Riemannian manifold, let  be a convex objective function and let
be a convex objective function and let  be a totally convex subset described by a system of inequalities
be a totally convex subset described by a system of inequalities 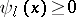 ,
, 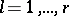 , where
, where 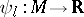 are concave functions (cf. Concave function). The solution of the convex programming problem
are concave functions (cf. Concave function). The solution of the convex programming problem  is completely characterized by a saddle-point theorem initially stated on
is completely characterized by a saddle-point theorem initially stated on  [a2] by H.W. Kuhn and A.W. Tucker.
[a2] by H.W. Kuhn and A.W. Tucker.
Suppose 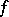 and
and 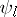 are of class
are of class 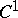 , and let
, and let  . Introduce the Lagrange function
. Introduce the Lagrange function  ,
, 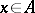 ,
, 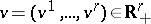 . A point
. A point 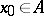 is the optimal solution of the convex programming problem
is the optimal solution of the convex programming problem  if and only if there exists a
if and only if there exists a 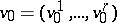 such that the so-called Kuhn–Tucker conditions hold:
such that the so-called Kuhn–Tucker conditions hold:
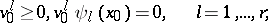 |
 |
These equations provide a criterion for testing whether a solution which has been found by other methods is in fact an optimal solution.
The Kuhn–Tucker theorem has been generalized in various directions:
to necessary or sufficient conditions, or both types, for an extremum of a function subject to equality or inequality constraints [a1];
to changing the geometrical structure of the underlying manifold [a1], [a3].
References
| [a1] | K.J. Arrow, L. Hurwicz, H. Uzawa, "Studies in linear and nonlinear programming" , Stanford Univ. Press (1958) |
| [a2] | H.W. Kuhn, A.W. Tucker, "Nonlinear programming" , Proc. Second Berkeley Symp. Math. Stat. Probab. , California Univ. Press (1951) pp. 481–492 |
| [a3] | C. Udrişte, "Convex functions and optimization methods on Riemannian manifolds" , Kluwer Acad. Publ. (1994) |
Kuhn-Tucker conditions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kuhn-Tucker_conditions&oldid=12541