Kontorovich-Lebedev transform

Lebedev–Kontorovich transform

The integral transform

where 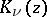is the Macdonald function.

This transform was introduced in [a1] and later investigated in [a2]. If 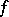is an integrable function with the weight 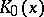, i.e. 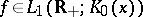, then is a bounded continuous function, which tends to zero at infinity (an analogue of the Riemann–Lebesgue lemma, cf. Fourier series, for the Fourier integral). If is a function of bounded variation in a neighbourhood of a point 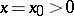and if

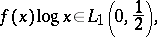then the following inversion formula holds:

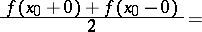If the Mellin transform of 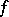, denoted by 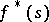, belongs to the space 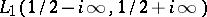, then 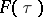can be represented by an integral (see [a6]):

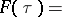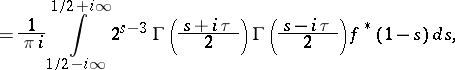where is the Euler gamma-function.

Let 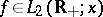. Then the integral 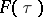converges in mean square and isomorphically maps the space 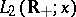onto the space 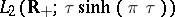. The inverse operator has the form [a10]

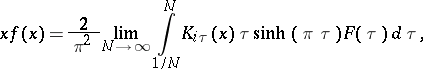and the Parseval equality holds (see also [a3], [a5]):

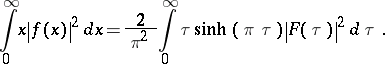The Kontorovich–Lebedev transform of distributions was considered in [a7], [a8]. A transform table for the Kontorovich–Lebedev transform can be found in [a4]. Special properties in -spaces are given in [a10].

For two functions 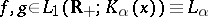, , 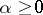define the operator of convolution for the Kontorovich–Lebedev transform as ([a9], [a10])

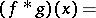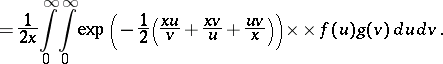The following norm estimate is true:

and the space 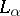forms a normed ring with the convolution 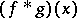as operation of multiplication.

If 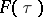, 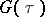are the Kontorovich–Lebedev transforms of two functions 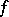, , 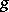then the factorization property is true:

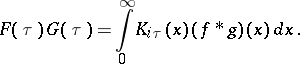If 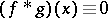in the ring 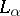, then at least one of the functions 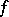, 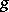is equal to zero almost-everywhere on 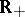(an analogue of the Titchmarsh theorem).

The Kontorovich–Lebedev transform is the simplest and most basic in the class of integral transforms of non-convolution type, which forms a special class of so-called index transforms (cf. also Index transform), depending upon parameters, subscripts (indices) of the hypergeometric functions (cf. Hypergeometric function) as kernels.

References

[a1]	M.I. Kontorovich, N.N. Lebedev, "A method for the solution of problems in diffraction theory and related topics" Zh. Eksper. Teor. Fiz. , 8 : 10–11 (1938) pp. 1192–1206 (In Russian)
[a2]	N.N. Lebedev, "Sur une formule d'inversion" Dokl. Akad. Sci. USSR , 52 (1946) pp. 655–658
[a3]	N.N. Lebedev, "Analog of the Parseval theorem for the one integral transform" Dokl. Akad. Nauk SSSR , 68 : 4 (1949) pp. 653–656 (In Russian)
[a4]	A. Erdélyi, W. Magnus, F. Oberhettinger, "Tables of integral transforms 1-2" , McGraw-Hill (1954) pp. Chap. XII
[a5]	I.N. Sneddon, "The use of integral transforms" , McGraw-Hill (1972) pp. Chap. 6
[a6]	Vu Kim Tuan, S.B. Yakubovich, "The Kontorovich–Lebedev transform in a new class of functions" Amer. Math. Soc. Transl. , 137 (1987) pp. 61–65
[a7]	S.B. Yakubovich, B. Fisher, "On the Kontorovich–Lebedev transformation on distributions" Proc. Amer. Math. Soc. , 122 : 3 (1994) pp. 773–777
[a8]	A.H. Zemanian, "The Kontorovich–Lebedev transformation on distributions of compact support and its inversion" Math. Proc. Cambridge Philos. Soc. , 77 (1975) pp. 139–143
[a9]	S.B. Yakubovich, Yu.F. Luchko, "The hypergeometric approach to integral transforms and convolutions" , Kluwer Acad. Publ. (1994) pp. Chap. 6
[a10]	S.B. Yakubovich, "Index transforms" , World Sci. (1996) pp. Chaps. 2;4