Hilbert-Schmidt operator

An operator acting on a Hilbert space such that for any orthonormal basis in the following condition is met:

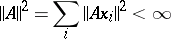(however, this need be true for some basis only). A Hilbert–Schmidt operator is a compact operator for which the condition

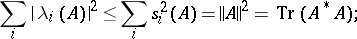applies to its -numbers and its eigen values ; here 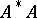is a trace-class operator ( is the adjoint of and 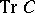is the trace of an operator 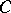). The set of all Hilbert–Schmidt operators on a fixed space 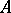forms a Hilbert space with scalar product

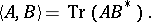If 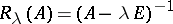is the resolvent of and

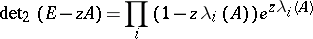is its regularized characteristic determinant, then the Carleman inequality

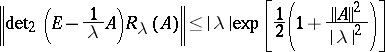holds.

A typical representative of a Hilbert–Schmidt operator is a Hilbert–Schmidt integral operator (which explains the origin of the name).

Comments

The -numbers or singular values of are the (positive) eigen values of the self-adjoint operator 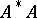. Instead of Hilbert–Schmidt operator one also says "of Hilbert–Schmidt class operatorHilbert–Schmidt class" . A bounded operator 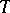on a Hilbert space is said to be of trace class if for arbitrary complete orthonormal systems 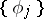, 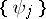. Equivalently, 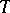is of trace class if 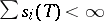. The trace of such an operator is defined as 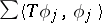, where 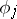is any orthonormal basis. The product of two Hilbert–Schmidt operators is of trace class and the converse is also true.

The norm 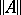in the above article is not the usual operator norm of 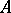but its Hilbert–Schmidt norm.

References

[a1]	M. Reed, B. Simon, "Methods of modern mathematical physics" , 1. Functional analysis , Acad. Press (1972)
[a2]	I.C. Gohberg, S. Goldberg, "Basic operator theory" , Birkhäuser (1977)
[a3]	N.I. Akhiezer, I.M. Glazman, "Theory of linear operators in Hilbert space" , 1–2 , Pitman (1981) (Translated from Russian)