Cohen-Macaulay ring

Macaulay ring

A commutative local Noetherian ring , the depth 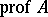of which is equal to its dimension 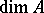. In homological terms, a Cohen–Macaulay ring 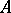is characterized as follows: The groups 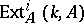, or the local cohomology groups 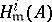, vanish for all , where is the maximal ideal in 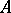and 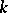is the residue field of 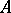. An alternative definition utilizes the concept of a regular sequence. A regular sequence is a sequence of elements of 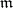such that, for all 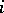, the element 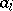is not a zero divisor in 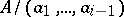. A local ring is a Cohen–Macaulay ring if there exists a regular sequence such that the quotient ring 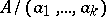is Artinian. In that case .

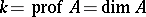If is a prime ideal in a Cohen–Macaulay ring 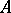, then its height 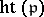(see Height of an ideal) satisfies the relation

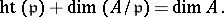In particular, a Cohen–Macaulay ring is equi-dimensional and it is a catenary ring. A fundamental result on Cohen–Macaulay rings is the following unmixedness theorem. Let 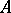be a 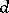-dimensional Cohen–Macaulay ring and a sequence of elements of such that 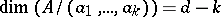. Then is a regular sequence and the ideal is unmixed, i.e. any prime ideal associated with 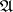has height 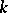and co-height 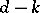. The unmixedness theorem was proved by F.S. Macaulay [1] for a polynomial ring and by I.S. Cohen [2] for a ring of formal power series.

Examples of Cohen–Macaulay rings. A regular local ring (and, in general, any Gorenstein ring) is a Cohen–Macaulay ring; any Artinian ring, any one-dimensional reduced ring, any two-dimensional normal ring — all these are Cohen–Macaulay rings. If 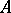is a local Cohen–Macaulay ring, then the same is true of its completion, of the ring of formal power series over 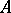and of any finite flat extension. A complete intersection of a Cohen–Macaulay ring 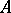, i.e. a quotient ring 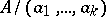, where is a regular sequence, is a Cohen–Macaulay ring. Finally, the localization of a Cohen–Macaulay ring in a prime ideal is again a Cohen–Macaulay ring. This makes it possible to extend the definition of a Cohen–Macaulay ring to arbitrary rings and schemes. Indeed, a Noetherian ring 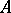(a scheme 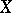) is called a Cohen–Macaulay ring (a Cohen–Macaulay scheme) if for any prime ideal 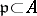(respectively, for any point 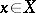) the local ring 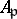(respectively, 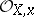) is a Cohen–Macaulay ring; for example, this is true of any semi-group ring 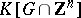, where 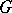is a convex polyhedral cone in (see [6]).

Cohen–Macaulay rings are also stable under passage to rings of invariants. If 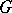is a finite group acting on a Cohen–Macaulay ring 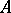, and if moreover its order is invertible in 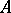, then the ring of invariants is also a Cohen–Macaulay ring.

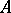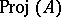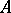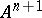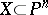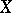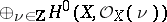If is a graded ring, the property of being a Cohen–Macaulay ring appears in the cohomology of the invertible sheaves over the projective scheme (see [4]). If the homogeneous ring of a cone in associated with a projective variety is a Cohen–Macaulay ring, then is called an arithmetical Cohen–Macaulay variety. In that case the ring is isomorphic to , and 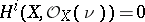for all 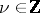and 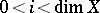, where 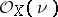is the -th tensor power of the polarized invertible sheaf 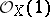on 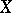. This property holds for projective spaces and their products, complete intersections, Grassmann manifolds and Schubert subvarieties [7], flag manifolds and generalized flag manifolds [8].

A module 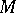over a local ring 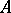is called a Cohen–Macaulay module if its depth equals its dimension. Many results for Cohen–Macaulay rings carry over to Cohen–Macaulay modules; for example, the support of such a module is equi-dimensional. It has been conjectured that any local complete ring 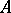has a Cohen–Macaulay module 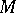such that .

References

[1]	F.S. Macaulay, "The algebraic theory of modular systems" , Cambridge Univ. Press (1916)
[2]	I.S. Cohen, "On the structure and ideal theory of complete local rings" Trans. Amer. Math. Soc. , 59 (1946) pp. 54–106
[3]	O. Zariski, P. Samuel, "Commutative algebra" , 2 , v. Nostrand (1960)
[4]	D. Mumford, "Lectures on curves on an algebraic surface" , Princeton Univ. Press (1966)
[5]	J.-P. Serre, "Algèbre locale. Multiplicités" , Lect. notes in math. , 11 , Springer (1965)
[6]	M. Hochster, "Rings of invariants of tori, Cohen–Macaulay rings generated by monomials, and polytopes" Ann. of Math. , 96 (1972) pp. 318–337
[7]	M. Hochster, "Grassmannians and their Schubert subvarieties are arithmetically Cohen–Macaulay" J. of Algebra , 25 (1973) pp. 40–57
[8]	G.R. Kempf, "Linear systems on homogeneous spaces" Ann. of Math. , 103 (1976) pp. 557–591

Comments

For the concepts of depth, dimension, regular local ring, normal ring, Gorenstein ring, cf., respectively, Depth of a module; Dimension; Local ring; Normal ring; Gorenstein ring. For a description of the invertible sheaf 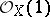cf. also Projective spectrum of a ring, and for a discussion of the local cohomology groups 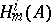cf. Local cohomology and Koszul complex.