Difference between revisions of "Compact group"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 12: | Line 12: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Weil, "l'Intégration dans les groupes topologiques et ses applications" , Hermann (1940)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> D.P. Zhelobenko, "Compact Lie groups and their representations" , Amer. Math. Soc. (1973) (Translated from Russian)</TD></TR></table> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) {{MR|0201557}} {{ZBL|0022.17104}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Weil, "l'Intégration dans les groupes topologiques et ses applications" , Hermann (1940) {{MR|0005741}} {{ZBL|}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian) {{MR|0793377}} {{ZBL|0484.22018}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> D.P. Zhelobenko, "Compact Lie groups and their representations" , Amer. Math. Soc. (1973) (Translated from Russian) {{MR|0473097}} {{MR|0473098}} {{ZBL|0228.22013}} </TD></TR></table> |
| Line 20: | Line 20: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , '''1–2''' , Springer (1970)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> D. Montgomery, L. Zippin, "Topological transformation groups" , Interscience (1955)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French)</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , '''1–2''' , Springer (1970) {{MR|0262773}} {{ZBL|0213.40103}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> D. Montgomery, L. Zippin, "Topological transformation groups" , Interscience (1955) {{MR|0073104}} {{ZBL|0068.01904}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) {{MR|0682756}} {{ZBL|0319.17002}} </TD></TR></table> |
Revision as of 10:02, 24 March 2012
A topological group that is compact as a topological space. For example, every finite group (in the discrete topology) is a compact group. An algebraic group, even though it is a compact topological space (with respect to the Zariski topology), is not a topological group with respect to this topology and therefore is not a compact group.
The following groups are two important classes of compact groups.
1) Locally connected compact groups. Examples of such compact groups are the group  of all unitary complex matrices of order
of all unitary complex matrices of order  , the group
, the group 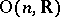 of all orthogonal real matrices of order
of all orthogonal real matrices of order  (with the topology induced by the topology determined by the ordinary norm of the fields
(with the topology induced by the topology determined by the ordinary norm of the fields 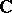 and
and  , respectively) and, more generally, any compact real Lie group.
, respectively) and, more generally, any compact real Lie group.
2) Totally-disconnected compact groups. Of this type is the group 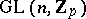 of invertible matrices of order
of invertible matrices of order  with coefficients in the ring
with coefficients in the ring  of
of 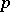 -adic integers (with the topology induced by that determined by the
-adic integers (with the topology induced by that determined by the  -adic norm of
-adic norm of 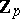 ; see Totally-disconnected space).
; see Totally-disconnected space).
Any totally-disconnected compact group is a profinite group, and conversely, every profinite group is a totally-disconnected compact group. The totally-disconnected compact Hausdorff groups can be characterized as the compact groups of topological dimension zero. If 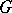 is locally connected and finite-dimensional, then
is locally connected and finite-dimensional, then 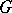 is a real Lie group [1]. The structure of a compact group of general type is to a certain degree determined by the structure of these two types of compact groups. In an arbitrary finite-dimensional compact group
is a real Lie group [1]. The structure of a compact group of general type is to a certain degree determined by the structure of these two types of compact groups. In an arbitrary finite-dimensional compact group 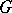 there is zero-dimensional subgroup
there is zero-dimensional subgroup 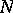 (lying in the centre of
(lying in the centre of 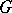 ) such that
) such that 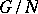 is a real Lie group and, furthermore, some neighbourhood of the identity in
is a real Lie group and, furthermore, some neighbourhood of the identity in  is the direct product of the group
is the direct product of the group 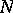 and a real local Lie group (cf. Lie group, local). Every connected finite-dimensional compact group has the form
and a real local Lie group (cf. Lie group, local). Every connected finite-dimensional compact group has the form 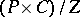 , where
, where 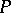 is a simply-connected compact semi-simple real Lie group,
is a simply-connected compact semi-simple real Lie group, 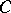 is a finite-dimensional connected commutative compact group and
is a finite-dimensional connected commutative compact group and 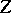 is a finite central normal subgroup for which only the identity lies in
is a finite central normal subgroup for which only the identity lies in 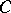 . The study of the structure of connected compact real Lie groups has led to a complete classification of them (see Lie group, compact); the structure of commutative compact groups is elucidated in the theory of Pontryagin duality. Any compact group (not necessarily finite-dimensional) is the projective limit of compact real Lie groups [2]. The topological structure of the above two types of compact groups is as follows: Every locally connected finite-dimensional compact group is a topological manifold, while every infinite zero-dimensional compact group with a countable base is homeomorphic to the perfect Cantor set.
. The study of the structure of connected compact real Lie groups has led to a complete classification of them (see Lie group, compact); the structure of commutative compact groups is elucidated in the theory of Pontryagin duality. Any compact group (not necessarily finite-dimensional) is the projective limit of compact real Lie groups [2]. The topological structure of the above two types of compact groups is as follows: Every locally connected finite-dimensional compact group is a topological manifold, while every infinite zero-dimensional compact group with a countable base is homeomorphic to the perfect Cantor set.
The study of structure of compact groups is based on the fact that every compact group 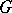 has a sufficient system of finite-dimensional linear representations, that is, for any element
has a sufficient system of finite-dimensional linear representations, that is, for any element 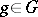 there exists a continuous finite-dimensional linear representation
there exists a continuous finite-dimensional linear representation  for which
for which 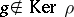 . This fact is one of the important results of the well-developed general theory of linear representations of compact groups. This theory makes essential use of the fact that every compact group has a two-sided invariant measure
. This fact is one of the important results of the well-developed general theory of linear representations of compact groups. This theory makes essential use of the fact that every compact group has a two-sided invariant measure  (a Haar measure), which enables one to define invariant integration on
(a Haar measure), which enables one to define invariant integration on 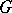 . The most important facts of this theory are as follows. Every continuous representation of a compact group
. The most important facts of this theory are as follows. Every continuous representation of a compact group 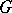 in a pre-Hilbert space is equivalent to a unitary representation. Let
in a pre-Hilbert space is equivalent to a unitary representation. Let 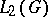 be the Hilbert space of square-integrable complex-valued functions on
be the Hilbert space of square-integrable complex-valued functions on 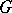 with respect to the invariant measure
with respect to the invariant measure  . The action of the group
. The action of the group  on the functions by left and right translations determines on
on the functions by left and right translations determines on 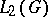 the structure of a left and a right
the structure of a left and a right  -module. The corresponding representations are respectively called the left and right regular representation of
-module. The corresponding representations are respectively called the left and right regular representation of 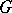 ; they are unitary and unitarily equivalent. Let
; they are unitary and unitarily equivalent. Let  be the family of all possible pairwise inequivalent finite-dimensional irreducible unitary representations of the compact group
be the family of all possible pairwise inequivalent finite-dimensional irreducible unitary representations of the compact group 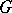 and let
and let 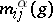 ,
,  , be the set of matrix elements of the representation
, be the set of matrix elements of the representation 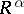 in some orthonormal basis. Then the functions
in some orthonormal basis. Then the functions 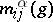 lie in
lie in  and form in it a complete orthogonal system, the norm of the function
and form in it a complete orthogonal system, the norm of the function  being
being 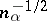 . Any continuous complex-valued function on
. Any continuous complex-valued function on 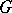 can, to any desired degree of accuracy, be uniformly approximated by finite linear combinations of the functions
can, to any desired degree of accuracy, be uniformly approximated by finite linear combinations of the functions  (the Peter–Weyl theorem). The characters of the irreducible unitary finite-dimensional representations are pairwise orthogonal and have norm 1. Continuous finite-dimensional unitary representations are equivalent if and only if their characters are equal. A continuous finite-dimensional unitary representation is irreducible if and only if the norm of its character (which lies in
(the Peter–Weyl theorem). The characters of the irreducible unitary finite-dimensional representations are pairwise orthogonal and have norm 1. Continuous finite-dimensional unitary representations are equivalent if and only if their characters are equal. A continuous finite-dimensional unitary representation is irreducible if and only if the norm of its character (which lies in 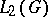 ) is equal to 1. Every irreducible continuous unitary representation of the group
) is equal to 1. Every irreducible continuous unitary representation of the group 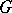 in a Hilbert space is finite-dimensional. Every continuous unitary representation of the group in a Hilbert space is an orthogonal direct sum of unitary representations that are multiples of finite-dimensional irreducible representations. In particular, the multiplicity of the imbedding of the representation
in a Hilbert space is finite-dimensional. Every continuous unitary representation of the group in a Hilbert space is an orthogonal direct sum of unitary representations that are multiples of finite-dimensional irreducible representations. In particular, the multiplicity of the imbedding of the representation 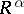 in the right regular representation is equal to
in the right regular representation is equal to 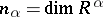 ; furthermore, the sum of all
; furthermore, the sum of all 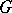 -submodules in the
-submodules in the 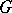 -module
-module  that are isomorphic to
that are isomorphic to 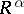 is precisely the linear span of all the
is precisely the linear span of all the 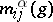 .
.
References
| [1] | L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian) MR0201557 Zbl 0022.17104 |
| [2] | A. Weil, "l'Intégration dans les groupes topologiques et ses applications" , Hermann (1940) MR0005741 |
| [3] | M.A. Naimark, "Theory of group representations" , Springer (1982) (Translated from Russian) MR0793377 Zbl 0484.22018 |
| [4] | D.P. Zhelobenko, "Compact Lie groups and their representations" , Amer. Math. Soc. (1973) (Translated from Russian) MR0473097 MR0473098 Zbl 0228.22013 |
Comments
References
| [a1] | E. Hewitt, K.A. Ross, "Abstract harmonic analysis" , 1–2 , Springer (1970) MR0262773 Zbl 0213.40103 |
| [a2] | D. Montgomery, L. Zippin, "Topological transformation groups" , Interscience (1955) MR0073104 Zbl 0068.01904 |
| [a3] | N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) MR0682756 Zbl 0319.17002 |
Compact group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Compact_group&oldid=15731