Spearman rho metric
Spearman rho
The non-parametric correlation coefficient (or measure of association) known as Spearman's rho was first discussed by the psychologist C. Spearman in 1904 [a4] as a coefficient of correlation on ranks (cf. also Correlation coefficient; Rank statistic). In modern use, the term "correlation" refers to a measure of a linear relationship between variates (such as the Pearson product-moment correlation coefficient), while "measure of association" refers to a measure of a monotone relationship between variates (such as the Kendall tau metric and Spearman's rho). For an historical review of Spearman's rho and related coefficients, see [a2].
Spearman's rho, denoted 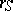 , is computed by applying the Pearson product-moment correlation coefficient procedure to the ranks associated with a sample
, is computed by applying the Pearson product-moment correlation coefficient procedure to the ranks associated with a sample 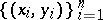 . Let
. Let 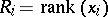 and
and  ; then computing the sample (Pearson) correlation coefficient
; then computing the sample (Pearson) correlation coefficient  for
for 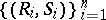 yields
yields
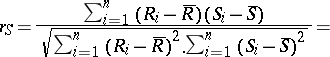 |
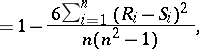 |
where  . When ties exist in the data, the following adjusted formula for
. When ties exist in the data, the following adjusted formula for 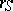 is used:
is used:
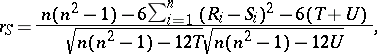 |
where 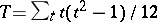 for
for 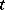 the number of
the number of 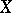 observations that are tied at a given rank, and
observations that are tied at a given rank, and 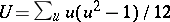 for
for  the number of
the number of 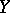 observations that are tied at a given rank. For details on the use of
observations that are tied at a given rank. For details on the use of 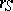 in hypothesis testing, and for large-sample theory, see [a1].
in hypothesis testing, and for large-sample theory, see [a1].
If 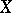 and
and  are random variables (cf. Random variable) with respective distribution functions
are random variables (cf. Random variable) with respective distribution functions 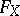 and
and  , then the population parameter estimated by
, then the population parameter estimated by  , usually denoted
, usually denoted 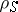 , is defined to be the Pearson product-moment correlation coefficient of the random variables
, is defined to be the Pearson product-moment correlation coefficient of the random variables  and
and  :
:
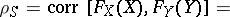 |
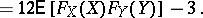 |
Spearman's  is occasionally referred to as the grade correlation coefficient, since
is occasionally referred to as the grade correlation coefficient, since 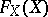 and
and  are sometimes called the "grades" of
are sometimes called the "grades" of 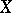 and
and 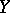 .
.
Like Kendall's tau, 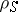 is a measure of association based on the notion of concordance. One says that two pairs
is a measure of association based on the notion of concordance. One says that two pairs 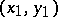 and
and  of real numbers are concordant if
of real numbers are concordant if 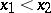 and
and 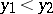 or if
or if 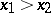 and
and 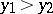 (i.e., if
(i.e., if 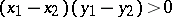 ); and discordant if
); and discordant if 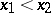 and
and 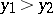 or if
or if 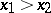 and
and 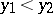 (i.e., if
(i.e., if 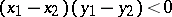 ). Now, let
). Now, let  ,
, 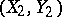 and
and  be independent random vectors with the same distribution as
be independent random vectors with the same distribution as 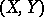 . Then
. Then
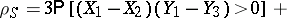 |
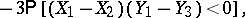 |
that is, 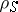 is proportional to the difference between the probabilities of concordance and discordance between the random vectors
is proportional to the difference between the probabilities of concordance and discordance between the random vectors 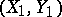 and
and  (clearly,
(clearly,  can be replaced by
can be replaced by  ).
).
When 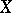 and
and  are continuous,
are continuous,
 |
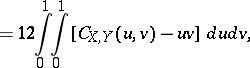 |
where  is the copula of
is the copula of 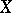 and
and 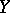 . Consequently,
. Consequently, 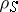 is invariant under strictly increasing transformations of
is invariant under strictly increasing transformations of 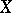 and
and  , a property
, a property 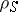 shares with Kendall's tau but not with the Pearson product-moment correlation coefficient. Note that
shares with Kendall's tau but not with the Pearson product-moment correlation coefficient. Note that 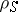 is proportional to the signed volume between the graphs of the copula
is proportional to the signed volume between the graphs of the copula 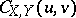 and the "product" copula
and the "product" copula 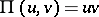 , the copula of independent random variables. For a survey of copulas and their relationship with measures of association, see [a3].
, the copula of independent random variables. For a survey of copulas and their relationship with measures of association, see [a3].
Spearman [a5] also proposed an  version of
version of 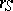 , known as Spearman's footrule, based on absolute differences
, known as Spearman's footrule, based on absolute differences  in ranks rather than squared differences:
in ranks rather than squared differences:
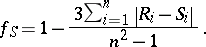 |
The population parameter 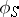 estimated by
estimated by 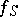 is given by
is given by
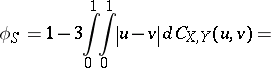 |
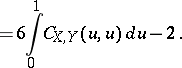 |
References
| [a1] | J.D. Gibbons, "Nonparametric methods for quantitative analysis" , Holt, Rinehart & Winston (1976) |
| [a2] | W.H. Kruskal, "Ordinal measures of association" J. Amer. Statist. Assoc. , 53 (1958) pp. 814–861 |
| [a3] | R.B. Nelsen, "An introduction to copulas" , Springer (1999) |
| [a4] | C. Spearman, "The proof and measurement of association between two things" Amer. J. Psychol. , 15 (1904) pp. 72–101 |
| [a5] | C. Spearman, "A footrule for measuring correlation" Brit. J. Psychol. , 2 (1906) pp. 89–108 |
Spearman rho metric. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Spearman_rho_metric&oldid=15466