Poisson formula
The same as Poisson integral.
A formula giving an integral representation for the solution of the Cauchy problem for the wave equation in  :
:
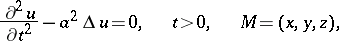 |
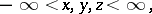 |
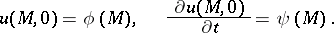 |
This solution has the form
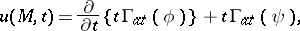 | (1) |
where
 |
is the mean value of the function 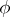 on the sphere
on the sphere 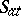 in the
in the 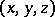 -space of radius
-space of radius 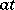 and centre at the point
and centre at the point 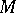 , and
, and 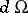 is the area element on the unit sphere. In the case of the inhomogeneous wave equation a third term is added to formula (1) (see ).
is the area element on the unit sphere. In the case of the inhomogeneous wave equation a third term is added to formula (1) (see ).
From formula (1), by the method of descent (cf. Descent, method of) formulas are obtained for solving the Cauchy problem in two- (Poisson's formula) and one- (d'Alembert formula) dimensional space (see ). See also Kirchhoff formula.
Sometimes the phrase "Poisson formula" is used for the integral representation of the solution to the Cauchy problem for the heat equation in the space  :
:
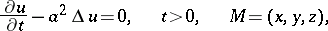 |
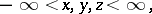 |
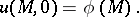 |
This solution has the form
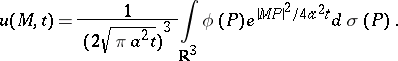 | (2) |
Formula (2) immediately generalizes to an  -dimensional space,
-dimensional space, 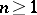 .
.
References
| [1] | S.D. Poisson, Mém. Acad. Sci. Paris , 3 (1818) pp. 121–176 |
| [2] | A.N. Tikhonov, A.A. Samarskii, "Equations of mathematical physics" , Pergamon (1963) (Translated from Russian) |
| [3] | V.S. Vladimirov, "Equations of mathematical physics" , MIR (1984) (Translated from Russian) |
Comments
References
| [a1] | G.B. Whitham, "Linear and non-linear waves" , Wiley (Interscience) (1974) pp. 229ff |
| [a2] | A.V. Bitsadze, "Equations of mathematical physics" , MIR (1980) pp. 179 (Translated from Russian) |
Poisson formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poisson_formula&oldid=14431