Markov spectrum problem
A problem in number theory which arises in connection with the distribution of the normalized values of arithmetic minima of indefinite binary quadratic forms (cf. Binary quadratic form). Let
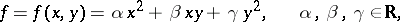 |
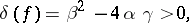 |
and let
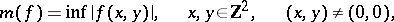 |
be the uniform arithmetic minimum of the form 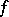 . The number
. The number
 |
is called the Markov constant of 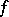 . The set
. The set 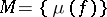 , where
, where  runs through all real indefinite quadratic forms, is called the Markov spectrum. The Markov constant and the Markov spectrum have been defined in various ways; in particular, A.A. Markov in
runs through all real indefinite quadratic forms, is called the Markov spectrum. The Markov constant and the Markov spectrum have been defined in various ways; in particular, A.A. Markov in
considered the set 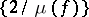 . It is known that
. It is known that 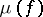 is an invariant of a ray
is an invariant of a ray  of classes of forms, that is, of a set
of classes of forms, that is, of a set
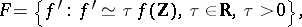 | (1) |
since 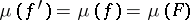 . Each ray of classes
. Each ray of classes 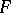 is in one-to-one correspondence with a doubly-infinite (infinite in both directions) sequence
is in one-to-one correspondence with a doubly-infinite (infinite in both directions) sequence
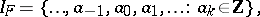 |
such that if one puts
 |
( is the notation for a continued fraction), then
is the notation for a continued fraction), then
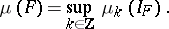 |
The Markov problem can be stated as follows: 1) describe the Markov spectrum 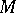 ; and 2) for each
; and 2) for each  , describe the set of forms
, describe the set of forms 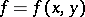 (or the rays
(or the rays 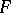 ) for which
) for which  . The problem was solved by Markov for the initial part of the spectrum
. The problem was solved by Markov for the initial part of the spectrum  defined by the condition
defined by the condition 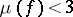 . This part of the spectrum is a discrete set:
. This part of the spectrum is a discrete set:
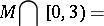 |
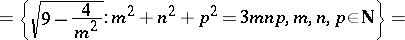 |
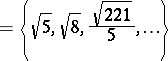 |
with the unique limit point 3 (a condensation point of  );
);  ,
,  and
and  run through all positive integer solutions of Markov's Diophantine equation
run through all positive integer solutions of Markov's Diophantine equation
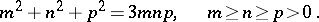 | (2) |
In this case there corresponds to each point of this part of the spectrum precisely one ray 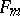 , given by a Markov form
, given by a Markov form  , with
, with
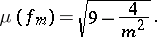 |
A solution 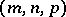 of (2) is called a Markov triple; the number
of (2) is called a Markov triple; the number 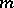 is called a Markov number. The Markov form
is called a Markov number. The Markov form 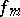 is associated to the Markov number
is associated to the Markov number  as follows. Let
as follows. Let  be defined by the conditions
be defined by the conditions
 |
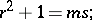 |
then, by definition,
 |
The set  is closed and there is a smallest number
is closed and there is a smallest number 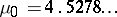 such that
such that 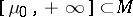 and
and  borders the interval of contiguity of
borders the interval of contiguity of  .
.
The Markov problem is closely related to the Lagrange–Hurwitz problem on rational approximation of a real number 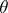 . The quantity
. The quantity
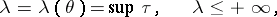 |
where the least upper bound is taken over all  ,
, 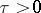 , for which
, for which
 |
has an infinite set of solutions  ,
, 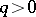 , is called a Lagrange constant. The set
, is called a Lagrange constant. The set 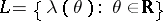 is called the Lagrange spectrum. It is natural to regard Lagrange's theorem as the first result in the theory of the Lagrange spectrum: All convergents of the continued fraction expansion of
is called the Lagrange spectrum. It is natural to regard Lagrange's theorem as the first result in the theory of the Lagrange spectrum: All convergents of the continued fraction expansion of 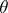 satisfy
satisfy
 |
If  , that is, if
, that is, if
 |
then 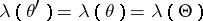 , where
, where 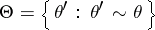 is an equivalence class of numbers. If
is an equivalence class of numbers. If 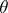 is expanded as a continued fraction
is expanded as a continued fraction 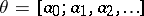 , then
, then
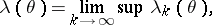 |
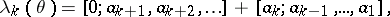 |
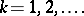 |
Thus, the Lagrange–Hurwitz problem can be stated as: a) describe the Lagrange spectrum 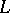 ; and b) for each
; and b) for each 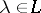 , describe the set of numbers
, describe the set of numbers  (or classes
(or classes 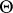 ) for which
) for which 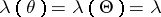 .
.
For  this problem reduces to the Markov problem; moreover,
this problem reduces to the Markov problem; moreover,
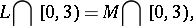 |
and to each  ,
, 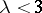 , corresponds precisely one class
, corresponds precisely one class 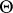 , described by the Markov form
, described by the Markov form 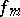 , provided the unicity conjecture is true. It has been proved that
, provided the unicity conjecture is true. It has been proved that 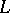 , like
, like 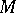 , is a closed set; that
, is a closed set; that 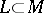 but
but  ; that
; that
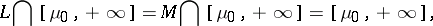 |
where  borders the interval of contiguity of
borders the interval of contiguity of 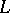 . Research into the structure of
. Research into the structure of 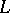 and the connection between
and the connection between 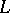 and
and 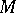 is described in [6]. For generalizations and analogues of the Markov spectrum problem and "isolation phenomena" see [2], [3], [7].
is described in [6]. For generalizations and analogues of the Markov spectrum problem and "isolation phenomena" see [2], [3], [7].
References
| [1a] | A. [A.A. Markov] Markoff, "Sur les formes quadratiques binaires indéfinies" Math. Ann. , 15 (1879) pp. 381–406 |
| [1b] | A. [A.A. Markov] Markoff, "Sur les formes quadratiques binaires indéfinies" Math. Ann. , 17 (1880) pp. 379–400 |
| [2] | J.W.S. Cassels, "An introduction to diophantine approximation" , Cambridge Univ. Press (1957) |
| [3] | B.N. Delone, "The Peterburg school of number theory" , Moscow-Leningrad (1947) (In Russian) |
| [4] | D.S. Gorshkov, "Lobachevskii geometry in connection with some problems of arithmetic" Zap. Nauchn. Sem. Leningrad. Otdel. Mat. Inst. Steklov. , 67 (1977) pp. 39–85 (In Russian) |
| [5] | G.A. Freiman, "Diophantine approximation and the geometry of numbers. (The Markov problem)" , Kalinin (1975) (In Russian) |
| [6] | A.V. Malyshev, "Markov and Lagrange spectra (a survey of the literature)" Zap. Nauchn. Sem. Leningrad. Otdel. Mat. Inst. Steklov. , 67 (1977) pp. 5–38 (In Russian) |
| [7] | B.A. Venkov, "On an extremum problem of Markov for indefinite ternaire quadratic forms" Izv. Akad. Nauk SSSR Ser. Mat. , 9 (1945) pp. 429–494 (In Russian) (French summary) |
Comments
In equation (1) in the article above, the notation 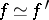 (
( ) refers to equivalence of binary forms over
) refers to equivalence of binary forms over 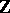 . More precisely,
. More precisely,  (
(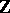 ) if and only if there are integers
) if and only if there are integers  ,
,  such that
such that 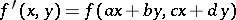 .
.
The "interval of contiguity of a Markov spectruminterval of contiguity" of 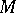 is simply the maximal interval
is simply the maximal interval 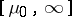 completely belonging to
completely belonging to  . The intersections
. The intersections 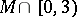 and
and 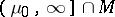 have been well-described. The structure of the portion between, i.e.
have been well-described. The structure of the portion between, i.e. 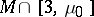 , is still (1989) unclear.
, is still (1989) unclear.
The unicity conjecture claims that the Markov number 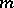 uniquely determines the triplet
uniquely determines the triplet  (and thus the Markov form
(and thus the Markov form  ). It is still (1989) a conjecture.
). It is still (1989) a conjecture.
References
| [a1] | D. Zagier, "On the number of Markoff numbers below a given bound" Math. Comp. , 39 (1982) pp. 709–723 |
| [a2] | T.W. Cusick, M.E. Flahive, "The Markoff and Lagrange spectra" , Amer. Math. Soc. (1989) |
Markov spectrum problem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Markov_spectrum_problem&oldid=13629