Manifold of figures (lines, surfaces, spheres)

A manifold whose constituent elements are various figures in a certain homogeneous space. From an analytic point of view the simplest figures are algebraic curves and surfaces. So the manifolds that have been investigated (as a rule, in Euclidean, affine and projective spaces) mainly have as constituent elements points, straight lines, planes, circles, spheres, conics, quadrics, and multi-dimensional analogues of these.

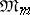An -dimensional manifold of figures 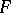of rank is defined by a closed system of differential equations

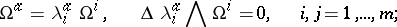(1)

where 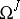(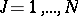) are the left-hand sides of the isotropy equations of a figure 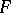and 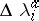are the Pfaffian forms arising upon closing the Pfaffian equations of the system (1), with 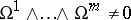(cf. Pfaffian form; Pfaffian equation). After carrying out a sequence of prolongations of the system (1), one obtains a sequence of fundamental objects of 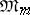, selects from it a basic object of the manifold, and carries out an invariant construction of the differential geometry of the manifold.

The differential geometry of ruled manifolds has been developed in depth. The simplest manifolds with non-linear constituent elements are manifolds of conics. With every one-dimensional manifold 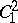of conics in a three-dimensional space (Euclidean, affine or projective) there is associated a torse 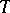, which is the envelope of the planes of the conics. A manifold 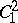is called focal or non-focal, depending on whether a generator of the torse is tangent to the conics or not. A two-dimensional manifold (a congruence) of conics in a three-dimensional space has, in general, six focal surfaces and six focal families. All the conics in the congruence are tangent to these surfaces. Congruences of conics with indefinite focal families (every two adjacent conics of which intersect up to second order) are characterized by all the conics in the congruence belonging to one quadric. Congruences of conics whose planes form a one-parameter family have one quadruplicate focal family to which correspond four focal points of intersection of two adjacent conics belonging to one plane. The two other focal points are points of intersection of a conic with a characteristic of its plane.

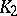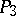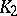A congruence of quadrics in has, in general, eight focal surfaces, to which all the quadrics in the congruence are tangent. A point of a quadric of the congruence of defined along any direction by the system of equations 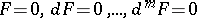, is called a focal point of order 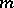of this quadric. A focal point of the second order is a fourfold point of the first order; a focal point of third order is a focal point of arbitrary order 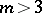. On each conic 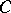of a three-dimensional manifold (a complex) of conics there are, in general, six invariant points (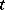-focal points of the conic). For each conic 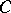of a complex whose planes form a two-parameter family, there is a unique conic 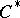passing through the characteristic point of the plane of 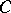and the four points of intersection of the conic with an adjacent conic in the same plane. The geometric properties of multi-parameter families of conics depend essentially on the number of parameters that characterize the planes of the conics of these families.

An immediate generalization of a conic in is a quadratic element — an 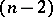-dimensional non-degenerate quadric i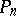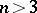n (). An 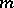-parameter family of quadratic elements whose hyperplanes form an 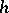-parameter family is called an 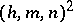-manifold in 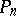. 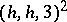-manifolds, 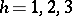, are the most general one-parameter families, congruences and complexes of conics in 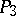. With each local quadratic elements of an 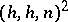-manifold for 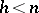is associated an 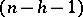-dimensional characteristic subspace and an 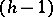-dimensional polar subspace. The rank of an 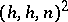-manifold is the number equal to 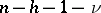, where is the dimension of the subspace in which the characteristic subspace intersects its polar subspace.

The differential geometry of an 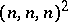-manifold can be regarded as the geometry of some regular hypersurface of an 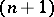-dimensional centro-projective space 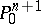in which the original -dimensional space plays the role of the fixed point.

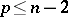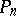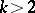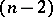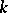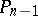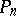A quadric of dimension in always lies in a -dimensional subspace. Algebraic surfaces of order do not have this property, in general. In investigating manifolds of algebraic surfaces it turns out to be advisable to select families of surfaces that lie in planes of dimension higher by one. A non-degenerate -dimensional algebraic surface of order belonging to a hyperplane of is called a plane algebraic element of order 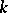. 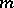An -dimensional manifold of plane algebraic elements of order 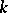whose hyperplanes form an -parameter family is called an 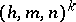-manifold. A fundamental object of the first order is the basic object of an 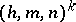-manifold.

In applications of geometry to hydromechanics, field theory and differential equations one uses manifolds of lines and surfaces that are not algebraic, in general. The study of such manifolds is of interest for geometry in its own right. A curvilinear congruence in a three-dimensional space is a two-dimensional family 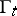of curves 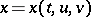such that through each point of the space passes, in general, a unique curve of the family. For fixed and a curve 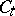of the family 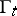is distinguished; for fixed and variable and a surface 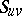is distinguished which is called a transversal surface of the congruence 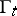. Points of 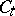at which are called focal points. 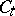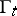The set of focal points of the curves of is called the focal surface of the congruence. A surface 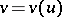of 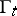on which the curves have an envelope is called a principal surface of .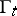

References

[1]	V.S. Malakhovaskii, "Differential geometry of manifolds of figures" J. Soviet Math. , 21 : 2 (1983) pp. 127–150 Itogi Nauk. i Tekhn. Probl. Geom. , 12 (1981) pp. 31–60

References

[a1]	P.A. Griffiths, J.E. Harris, "Principles of algebraic geometry" , Wiley (Interscience) (1978)