Jenkins theorem
general coefficient theorem
A theorem in the theory of univalent conformal mappings of families of domains on a Riemann surface, containing an inequality for the coefficients of the mapping functions, as well as conditions to be satisfied by the function so that the inequality becomes an equality. Jenkins' theorem is an exact expression and generalization of Teichmüller's principle (stated without proof, [1]), according to which the solution of a certain class of extremal problems for univalent functions is determined by the quadratic differentials of the respective forms. Obtained by J.A. Jenkins in 1954 [1]–[4].
The conditions of Jenkins' theorem. Let 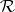 be a finite oriented Riemann surface, let
be a finite oriented Riemann surface, let 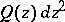 be a positive quadratic differential on
be a positive quadratic differential on 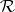 with at least one pole of order
with at least one pole of order 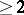 , and let
, and let 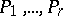 be all the poles of order 2, while
be all the poles of order 2, while 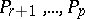 are all the poles of orders higher than 2. Let an open and everywhere-dense set
are all the poles of orders higher than 2. Let an open and everywhere-dense set 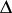 on
on 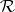 be the complement of the union of a finite number of closures of trajectories and closures of trajectory arcs, and let
be the complement of the union of a finite number of closures of trajectories and closures of trajectory arcs, and let 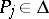 ,
, 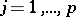 . Suppose the function
. Suppose the function  maps
maps 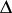 conformally and univalently (cf. Conformal mapping) into
conformally and univalently (cf. Conformal mapping) into 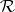 , and suppose there exists a homotopy
, and suppose there exists a homotopy
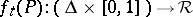 |
of the mapping 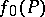 into the identity mapping
into the identity mapping 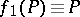 which leaves all poles from
which leaves all poles from 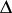 fixed and satisfies the condition
fixed and satisfies the condition 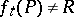 for each pole
for each pole 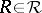 ,
, 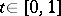 , and each point
, and each point 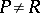 . Let
. Let 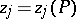 be a local parameter for the pole
be a local parameter for the pole 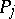 such that
such that 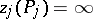 ,
,  . Let, for
. Let, for 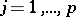 , in a neighbourhood of the pole
, in a neighbourhood of the pole 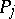 ,
,
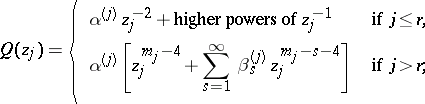 |
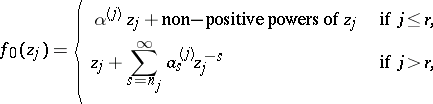 |
where 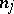 is the integer part of the number
is the integer part of the number 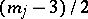 . Let
. Let
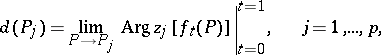 |
and let 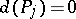 for all
for all 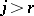 for which
for which 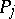 lies on the boundary of a strip-like domain with respect to
lies on the boundary of a strip-like domain with respect to 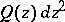 . Finally, let
. Finally, let
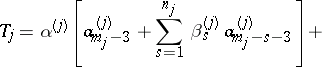 |
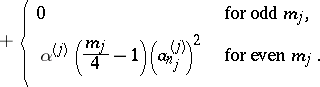 |
The statement of Jenkins' theorem. Under the conditions mentioned above,
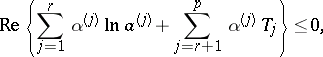 | (*) |
where 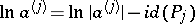 ,
, 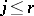 .
.
Jenkins' theorem in the case of equality. If in (*) the equality sign holds, then: a) in each domain 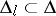 the mapping
the mapping  is an isometry in the
is an isometry in the 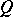 -metric:
-metric: 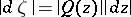 , each trajectory
, each trajectory 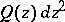 in
in 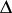 is mapped to a trajectory, and the set
is mapped to a trajectory, and the set  is everywhere-dense in
is everywhere-dense in 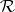 ; b) for
; b) for 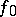 to be the identity mapping in a certain domain
to be the identity mapping in a certain domain 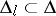 it is sufficient that one of the following additional conditions holds:
it is sufficient that one of the following additional conditions holds:
1) 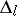 contains a pole
contains a pole 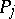 ,
, 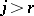 , of order
, of order  such that
such that 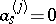 for
for 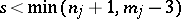 ;
;
2) 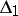 contains a pole
contains a pole 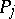 for which
for which  and
and 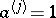 ;
;
3) 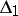 contains a point of a trajectory ending in a simple pole.
contains a point of a trajectory ending in a simple pole.
If (*) is an equality and if 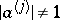 for a certain
for a certain 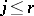 , then
, then  is conformally equivalent to the sphere,
is conformally equivalent to the sphere, 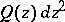 has no zeros or simple poles and
has no zeros or simple poles and 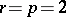 . If, in addition,
. If, in addition, 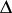 is a domain, the mapping
is a domain, the mapping 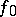 is conformally equivalent to a linear mapping all fixed points of which are images of the poles.
is conformally equivalent to a linear mapping all fixed points of which are images of the poles.
The method of the extremal metric (cf. Extremal metric, method of the), on which the proof of Jenkins' theorem is based, was employed by Jenkins, with suitable modifications, to obtain several other results, in particular the so-called special coefficient theorem [4]. For additions to and the development of Jenkins' theorem, see [5].
References
| [1] | J.A. Jenkins, "Univalent functions and conformal mapping" , Springer (1958) |
| [2] | J.A. Jenkins, "An extension of the general coefficient theorem" Trans. Amer. Math. Soc. , 95 : 3 (1960) pp. 387–407 |
| [3] | J.A. Jenkins, "The general coefficient theorem and certain applications" Bull. Amer. Math. Soc. , 68 : 1 (1962) pp. 1–9 |
| [4] | J.A. Jenkins, "Some area theorems and a special coefficient theorem" Illinois J. Math. , 8 : 1 (1964) pp. 80–99 |
| [5] | P.M. Tamrazov, "On the general coefficient theorem" Math. USSR Sb. , 1 (1967) pp. 49–59 Mat. Sb. , 72 : 1 (1967) pp. 59–71 |
Jenkins theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Jenkins_theorem&oldid=13018