Fundamental forms of a surface
The general name for the quadratic differential forms of the surface given in coordinates on the surface and satisfying the usual transformation laws under transformations of these coordinates. The fundamental forms of a surface characterize the basic intrinsic properties of the surface and the way it is located in space in a neighbourhood of a given point; one usually singles out the so-called first, second and third fundamental forms.
The first fundamental form characterizes the interior geometry of the surface in a neighbourhood of a given point. This means that measurements on the surface can be carried out by means of it. Suppose that the surface is given by the equation
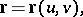 |
where  and
and  are coordinates on the surface; and
are coordinates on the surface; and
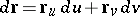 |
is the differential of the radius vector  along a chosen direction from a point
along a chosen direction from a point 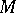 to an infinitesimally close point
to an infinitesimally close point  (see Fig. a).
(see Fig. a).
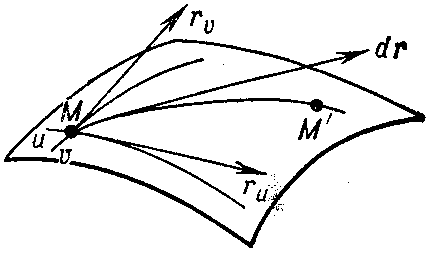
Figure: f042200a
The principal linear part of growth of the arc length 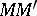 is expressed by the square of
is expressed by the square of 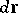 :
:
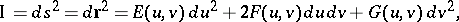 |
where
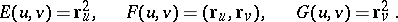 |
The form 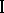 is the first fundamental form of the surface. See also first fundamental form of a surface.
is the first fundamental form of the surface. See also first fundamental form of a surface.
The second fundamental form characterizes the local structure of the surface in a neighbourhood of a regular point. Thus, choose
 |
a unit normal vector to the surface at  , where
, where 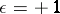 if the triple of vectors
if the triple of vectors 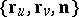 has a right-hand orientation and
has a right-hand orientation and 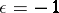 in the opposite case. The doubled principal linear part
in the opposite case. The doubled principal linear part 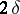 of the deviation of the point
of the deviation of the point 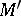 on the surface (see Fig. b) from the tangent plane at the point
on the surface (see Fig. b) from the tangent plane at the point  is given by
is given by
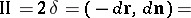 |
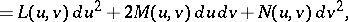 |
where
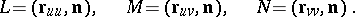 |
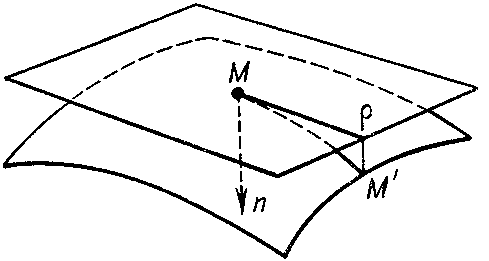
Figure: f042200b
The form  is called the second fundamental form of the surface. See also Second fundamental form.
is called the second fundamental form of the surface. See also Second fundamental form.
The first and second fundamental forms define two important common scalar quantities which are invariant under a transformation of the coordinates on the surface. Namely, the determinant of the ratio of the second with respect to the first one is the Gaussian curvature of the surface at the point:
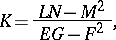 |
while the trace of this ratio,
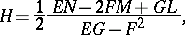 |
defines the mean curvature of the surface at the point.
Specifying the first (positive definite) and second fundamental forms defines the surface up to a motion (the Bonnet theorem).
The third fundamental form of the surface is the square of the differential of the unit normal vector  to the surface at the point
to the surface at the point  (see Fig. c):
(see Fig. c):
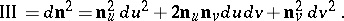 |
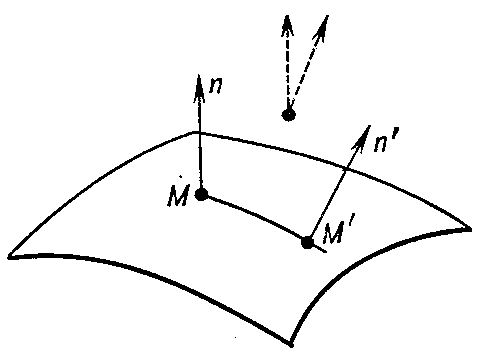
Figure: f042200c
The third fundamental form of a surface is equal to the principal linear part of growth of the angle between the vectors 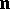 and
and  under displacement along the surface from
under displacement along the surface from 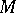 to
to 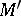 ; it is the first fundamental form of the spherical image of the surface (cf. Spherical map).
; it is the first fundamental form of the spherical image of the surface (cf. Spherical map).
The three fundamental forms are related by the linear dependence
 |
In addition to the fundamental forms listed above, other fundamental forms are sometimes encountered (see, for example, [3]).
References
| [1] | V.F. Kagan, "Foundations of the theory of surfaces in a tensor setting" , 1 , Moscow-Leningrad (1947) (In Russian) |
| [2] | P.K. Rashevskii, "A course of differential geometry" , Moscow (1956) (In Russian) |
| [3] | V.I. Shulikovskii, "Classical differential geometry in a tensor setting" , Moscow (1963) (In Russian) |
Comments
See also Quadratic surface forms; 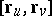 denotes the vector product of the vectors
denotes the vector product of the vectors 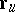 and
and 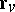 .
.
References
| [a1] | W. Klingenberg, "A course in differential geometry" , Springer (1978) (Translated from German) |
| [a2] | M. Spivak, "A comprehensive introduction to differential geometry" , 1979 , Publish or Perish pp. 1–5 |
| [a3] | W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1975) |
| [a4] | N.J. Hicks, "Notes on differential geometry" , v. Nostrand (1965) |
Fundamental forms of a surface. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fundamental_forms_of_a_surface&oldid=13236