Fractional power

of a linear operator on a complex Banach space

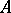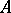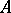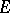A function of this operator such that . If the operator is bounded and its spectrum does not contain zero and does not surround it, is defined by a Cauchy integral along a contour around the spectrum of not containing zero. If is unbounded, the contour has to be taken infinite, and problems on the convergence of the integral arise. If has a domain of definition which is dense in , and has for 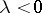a resolvent

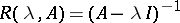satisfying the estimate

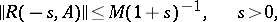(1)

then

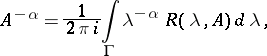where consists of the sides of an angle depending on 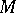. The operators 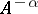are bounded and 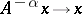for any as . Positive powers are defined as follows: 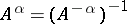; they are unbounded. For any real 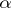and 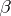the following fundamental property of powers holds:

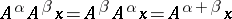for and . If 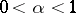, 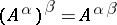. For any and ,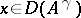

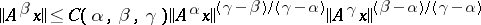(inequality of moments). The power semi-group 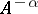permits extension to the semi-group 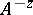which is analytic in the right half-plane.

The above properties are extended to include the case when has no bounded inverse and when the estimate 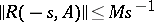, , 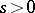holds. If condition (1) is met and if 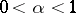, then

If is the infinitesimal operator of a contraction semi-group 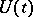, then

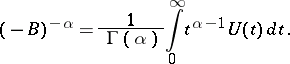It does not follow from condition (1) that 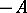is the infinitesimal operator of a strongly-continuous semi-group, but the operator 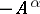is the infinitesimal operator of an analytic semi-group if .

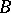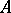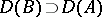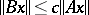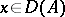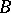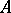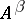An operator is dominated by an operator if and if , . If is dominated by and if the resolvents of both operators have the property (1), then is dominated by if .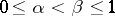

If 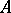is a positive self-adjoint operator on a Hilbert space, its fractional power is defined by the spectral decomposition (cf. Spectral decomposition of a linear operator):

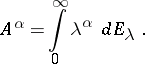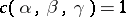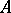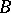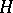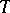In the inequality of moments, for such an operator. Let and be two positive self-adjoint operators, acting on Hilbert spaces and , respectively. If is a bounded linear operator from to 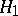with norm such that 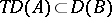and 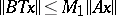, , 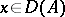then and

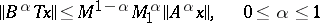(Heinz's inequality). In particular, if 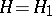and , the fact that 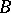is dominated by 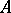implies that 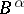is dominated by if . Fractional powers of operators are employed in the study of non-linear equations. They have been studied in detail for operators generated by elliptic boundary value problems.

References

[1]	S.G. Krein (ed.) , Functional analysis , Wolters-Noordhoff (1972) (Translated from Russian)
[2]	S.G. Krein, "Linear differential equations in Banach space" , Transl. Math. Monogr. , 29 , Amer. Math. Soc. (1971) (Translated from Russian)
[3]	R.T. Seeley, "Complex powers of elliptic operators" , Proc. Symp. Pure Math. , 10 , Amer. Math. Soc. (1967) pp. 288–307

References

[a1]	E. Hille, R.S. Phillips, "Functional analysis and semi-groups" , Amer. Math. Soc. (1957)