Fractional-linear mapping
fractional-linear transformation
A mapping of the complex space 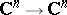 realized by fractional-linear functions (cf. Fractional-linear function).
realized by fractional-linear functions (cf. Fractional-linear function).
In the case of the complex plane 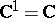 , this is a non-constant mapping of the form
, this is a non-constant mapping of the form
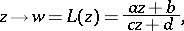 | (1) |
where  ; the unimodular normalization
; the unimodular normalization 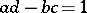 is often employed. Any fractional-linear mapping can be additionally defined by the correspondence
is often employed. Any fractional-linear mapping can be additionally defined by the correspondence 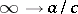 and
and 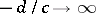 to yield a one-to-one mapping of the extended plane
to yield a one-to-one mapping of the extended plane 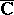 onto itself. The simplest fractional-linear mappings are the linear ones,
onto itself. The simplest fractional-linear mappings are the linear ones, 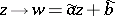 , which are obtained if
, which are obtained if 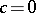 . All non-linear fractional-linear mappings can be represented as compositions of two linear mappings and of the mapping
. All non-linear fractional-linear mappings can be represented as compositions of two linear mappings and of the mapping 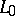 :
:  . The properties of the fractional-linear mapping
. The properties of the fractional-linear mapping 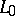 can be illustrated on the Riemann sphere, since if the stereographic projection is employed, it corresponds to the rotation of the sphere through 180° around the diameter passing through the images of the points
can be illustrated on the Riemann sphere, since if the stereographic projection is employed, it corresponds to the rotation of the sphere through 180° around the diameter passing through the images of the points 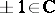 .
.
Special properties. A fractional-linear mapping maps  onto itself, conformally and bijectively. The circle property: Under a fractional-linear mapping any circle in
onto itself, conformally and bijectively. The circle property: Under a fractional-linear mapping any circle in 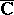 (i.e. a circle in
(i.e. a circle in  or a straight line supplemented by the point
or a straight line supplemented by the point 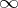 ) transforms into a circle in
) transforms into a circle in 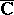 . The invariance of the ratio of two symmetrically-located points: A pair of points
. The invariance of the ratio of two symmetrically-located points: A pair of points 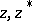 which is symmetric with respect to any circle in
which is symmetric with respect to any circle in 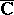 becomes, as a result of a fractional-linear mapping, a pair of points
becomes, as a result of a fractional-linear mapping, a pair of points 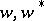 which is symmetric with respect to the image of this circle. The cross ratio between four points in
which is symmetric with respect to the image of this circle. The cross ratio between four points in 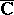 is invariant with respect to a fractional-linear mapping, i.e. if that mapping transforms the points
is invariant with respect to a fractional-linear mapping, i.e. if that mapping transforms the points 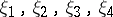 into the points
into the points 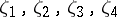 , respectively, then
, respectively, then
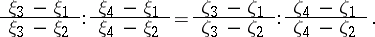 | (2) |
For any given triplets 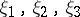 and
and 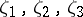 of pairwise distinct points in
of pairwise distinct points in 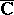 there exists one and only one fractional-linear mapping which transforms
there exists one and only one fractional-linear mapping which transforms 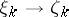 ,
, 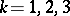 , respectively. This fractional-linear mapping can be found from equation (2) by substituting in it
, respectively. This fractional-linear mapping can be found from equation (2) by substituting in it  and
and  for
for 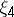 and
and 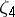 , respectively. The group property: The set of all fractional-linear mappings forms a non-commutative group with respect to composition
, respectively. The group property: The set of all fractional-linear mappings forms a non-commutative group with respect to composition 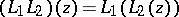 , with unit element
, with unit element 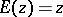 . The universality property: Any conformal automorphism of
. The universality property: Any conformal automorphism of 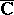 is a fractional-linear mapping and therefore the group of all fractional-linear mappings coincides with the group
is a fractional-linear mapping and therefore the group of all fractional-linear mappings coincides with the group 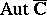 of all conformal automorphisms of
of all conformal automorphisms of  .
.
All conformal automorphisms of the unit disc 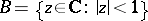 form a subgroup
form a subgroup 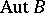 of the group
of the group 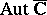 , consisting of fractional-linear mappings of the type
, consisting of fractional-linear mappings of the type
 |
The same applies to the conformal automorphisms of the upper half-plane 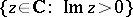 ; they have the form
; they have the form
 |
All conformal homeomorphisms of the upper half-plane onto the unit disc have the form
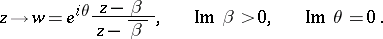 |
Except for the identity fractional-linear mapping 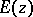 , fractional-linear mappings have at most two distinct fixed points
, fractional-linear mappings have at most two distinct fixed points  ,
, 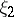 in
in  . If there are two fixed points
. If there are two fixed points 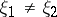 , the family
, the family 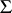 of circles passing through
of circles passing through  and
and 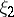 is transformed by the fractional-linear transformation (1) into itself. The family
is transformed by the fractional-linear transformation (1) into itself. The family 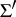 of all circles orthogonal to the circles of
of all circles orthogonal to the circles of  is also transformed into itself. Three cases are possible in this connection.
is also transformed into itself. Three cases are possible in this connection.
1) Each circle of 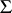 is transformed into itself; such a fractional-linear mapping is said to be hyperbolic and is representable in normal form
is transformed into itself; such a fractional-linear mapping is said to be hyperbolic and is representable in normal form
 | (3) |
where the multiplier of the mapping is 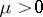 ,
, 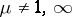 . A unimodular fractional-linear mapping (1) is hyperbolic if and only if
. A unimodular fractional-linear mapping (1) is hyperbolic if and only if 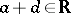 and
and 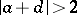 .
.
2) Each circle of  is transformed into itself; such a fractional-linear mapping is said to be elliptic and, in normal form (3), is characterized by a multiplier
is transformed into itself; such a fractional-linear mapping is said to be elliptic and, in normal form (3), is characterized by a multiplier  such that
such that 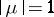 ,
, 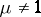 . A unimodular fractional-linear mapping (1) is elliptic if and only if
. A unimodular fractional-linear mapping (1) is elliptic if and only if  ,
, 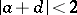 .
.
3) None of the circles of the families 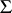 and
and 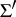 is transformed into itself; such a fractional-linear mapping is said to be loxodromic and, in normal form (3), is characterized by a multiplier
is transformed into itself; such a fractional-linear mapping is said to be loxodromic and, in normal form (3), is characterized by a multiplier 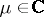 ,
, 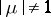 , such that either
, such that either 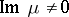 or
or 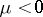 . A unimodular fractional-linear mapping (1) is loxodromic if and only if
. A unimodular fractional-linear mapping (1) is loxodromic if and only if 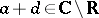 .
.
If two fixed points merge into one point 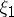 , the fractional-linear mapping is said to be parabolic. The family
, the fractional-linear mapping is said to be parabolic. The family 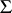 in such a case consists of all the circles having a common tangent at
in such a case consists of all the circles having a common tangent at  ; each circle is transformed into itself. The normal form of a parabolic fractional-linear mapping is
; each circle is transformed into itself. The normal form of a parabolic fractional-linear mapping is
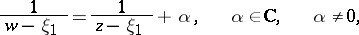 |
if 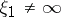 , or
, or
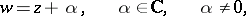 |
if 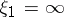 . A unimodular fractional-linear mapping (1) is parabolic if and only if
. A unimodular fractional-linear mapping (1) is parabolic if and only if 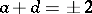 .
.
Owing to the many elementary properties listed above, fractional-linear mappings find extensive use in all branches of the theory of functions of a complex variable and in various applied disciplines. In particular, a model of Lobachevskii geometry can be constructed with the aid of fractional-linear mappings.
Among the subgroups of the complete group of fractional-linear mappings discrete groups 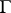 of fractional-linear mappings are the most important as regards applications to the analytic theory of differential equations, the theory of automorphic functions and other problems in analysis. Elementary discrete groups of fractional-linear mappings are the finite groups; they are isomorphic either to the cyclic rotation groups of the Riemann sphere or to the rotation groups of regular polyhedra. A discrete group
of fractional-linear mappings are the most important as regards applications to the analytic theory of differential equations, the theory of automorphic functions and other problems in analysis. Elementary discrete groups of fractional-linear mappings are the finite groups; they are isomorphic either to the cyclic rotation groups of the Riemann sphere or to the rotation groups of regular polyhedra. A discrete group  of fractional-linear mappings with an invariant circle
of fractional-linear mappings with an invariant circle  in
in 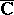 which is common for all transformations of
which is common for all transformations of 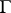 and for which the interior of
and for which the interior of 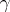 is transformed into itself under all transformations of
is transformed into itself under all transformations of  , is known as a Fuchsian group. A Fuchsian group cannot contain a loxodromic fractional-linear mapping. Historically, the first example of a Fuchsian group was the modular group appearing in the theory of elliptic functions (see also Modular function). The modular group consists of all unimodular fractional-linear mappings (1) in which the coefficients
, is known as a Fuchsian group. A Fuchsian group cannot contain a loxodromic fractional-linear mapping. Historically, the first example of a Fuchsian group was the modular group appearing in the theory of elliptic functions (see also Modular function). The modular group consists of all unimodular fractional-linear mappings (1) in which the coefficients  ,
, 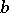 ,
,  ,
, 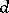 are integers; the real axis is invariant with respect to modular fractional-linear mappings. Non-elementary, non-Fuchsian groups of fractional-linear mappings — Kleinian groups (cf. Kleinian group) — are more complicated and have been studied to a lesser extent.
are integers; the real axis is invariant with respect to modular fractional-linear mappings. Non-elementary, non-Fuchsian groups of fractional-linear mappings — Kleinian groups (cf. Kleinian group) — are more complicated and have been studied to a lesser extent.
A fractional-linear mapping of the complex space 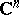 ,
, 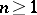 , is a non-degenerate mapping
, is a non-degenerate mapping
 |
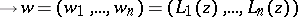 |
realizable by fractional-linear functions
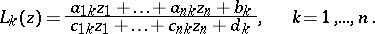 |
The most important fractional-linear mappings of 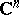 are those which extend to some compactification of
are those which extend to some compactification of 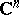 . Thus, all linear transformations involving a rearrangement of coordinates, as well as fractional-linear mappings of the type
. Thus, all linear transformations involving a rearrangement of coordinates, as well as fractional-linear mappings of the type
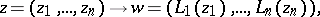 |
where 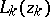 is a fractional-linear mapping of the type (1) in the plane
is a fractional-linear mapping of the type (1) in the plane 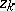 , extend to the function-theoretic space
, extend to the function-theoretic space  . The group of fractional-linear mappings generated by these mappings coincides with the group
. The group of fractional-linear mappings generated by these mappings coincides with the group 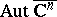 of all biholomorphic automorphisms of the compactification
of all biholomorphic automorphisms of the compactification 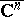 . The corresponding subgroup
. The corresponding subgroup 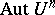 , with
, with
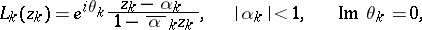 |
exhausts all the automorphisms of the unit polydisc  . Fractional-linear mappings in which
. Fractional-linear mappings in which
 | (4) |
extend to the projective closure 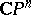 of the space
of the space 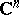 . This extension has the following form in homogeneous coordinates:
. This extension has the following form in homogeneous coordinates:
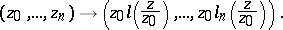 |
These mappings exhaust the group 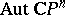 of all biholomorphic automorphisms of
of all biholomorphic automorphisms of 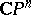 . The automorphisms of the unit ball
. The automorphisms of the unit ball 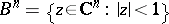 form the subgroup
form the subgroup 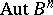 of the group
of the group 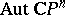 consisting of all fractional-linear mappings of the type (4) whose coefficients are subject to certain supplementary conditions (cf. [2], Vol. 2).
consisting of all fractional-linear mappings of the type (4) whose coefficients are subject to certain supplementary conditions (cf. [2], Vol. 2).
References
| [1] | I.I. [I.I. Privalov] Priwalow, "Einführung in die Funktionentheorie" , 1–3 , Teubner (1958–1959) (Translated from Russian) |
| [2] | B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1976) (In Russian) |
| [3] | S. Stoilow, "The theory of functions of a complex variable" , 1 , Moscow (1962) (In Russian; translated from Rumanian) |
| [4] | L.R. Ford, "Automorphic functions" , Chelsea, reprint (1951) |
Comments
A good reference for 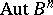 is [a1]. Fractional-linear mappings are also known as Möbius transformations.
is [a1]. Fractional-linear mappings are also known as Möbius transformations.
References
| [a1] | W. Rudin, "Function theory in the unit ball in 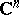 " , Springer (1980) " , Springer (1980) |
| [a2] | Z. Nehari, "Conformal mapping" , Dover, reprint (1975) |
| [a3] | H. Schwerdtfeger, "Geometry of complex numbers" , Dover, reprint (1979) |
| [a4] | A.I. Markushevich, "Theory of functions of a complex variable" , 1 , Chelsea (1977) (Translated from Russian) |
Fractional-linear mapping. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fractional-linear_mapping&oldid=17849