Flock

of a quadric set

In the projective space 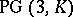, where 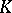is a field, there are three types of quadric sets: the elliptic and hyperbolic quadrics and the quadratic cone (cf. also Quadric). A flock is a covering of a quadric set except for possibly two points by mutually disjoint conics contained in planes (cf. also Conic). Thus, a flock may be either elliptic, hyperbolic or quadratic. A linear flock is one where the planes of the conics involved share a line. When is a finite field, it is possible to completely classify the elliptic and hyperbolic flocks. In particular, finite elliptic flocks are always linear (see, e.g., [a1]; however, for infinite fields, there are a great variety of non-linear flocks and there is no general classification [a2], p. 255, [a3]).

A partial flock is a set of mutually disjoint conics. A partial conical flock of deficiency one is a partial flock such that for each line of the cone there is exactly one point of the line which is not covered by the set of conics of the partial flock. A partial hyperbolic flock of deficiency one is a partial flock such that for each line of either ruling of the quadric there is exactly one point of the line which is not covered by the set of conics of the partial flock.

In the finite case, each partial conical flock of deficiency one may be uniquely extended to a flock (see [a11]), but there are proper partial hyperbolic flocks of deficiency one which may not be extended (see, e.g., [a10]).

Associated with flocks of quadric sets are certain types of affine (or projective) planes, called translation planes since they admit a collineation group of translations acting transitively on the points (carrying any one point onto any other). A regulus in 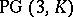is a set of lines which is completely covered by another set of lines called the opposite regulus. Each line of the opposite regulus intersects each line of the regulus in a unique point. The set of lines 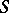incident with a particular point of the associated translation planes corresponds to a set 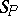of lines of 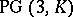, called the spread of the plane, with the following properties: An elliptic flock corresponds to a translation plane with spread a union of mutually disjoint reguli and at most two extra lines. A hyperbolic flock corresponds to a translation plane with spread a union of reguli that mutually share exactly two lines and a conical flock corresponds to a translation plane with spread a union of reguli that mutually share exactly one line.

In the finite case, hyperbolic flocks are completely classified (see [a4], [a5]). The associated translation planes are all near-field planes, which are translation planes for which the set of non-zero elements forms a group under multiplication in the associated ternary ring. However, in the infinite case, there are infinitely many mutually non-isomorphic hyperbolic flocks (see, e.g., [a6]).

Also associated with conical flocks in the finite case are certain generalized quadrangles, which are point-line geometries such that two points are incident with at most one line, two lines intersect in at most one point and given a point-line pair where 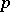is not incident with 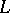, there is a unique point incident with such that 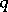and 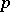are incident with a line 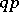(cf. also Quadrangle). However, in the infinite case this need not necessarily be the case (see, e.g., [a7], [a8]).

References

[a1]	J.A. Thas, "Flocks of egglike inversive planes" A. Barlotti (ed.) , Finite Geometric Structures and their Applications (1973) pp. 189–191.
[a2]	P. Dembowski, "Finite geometries" , Springer (l967.)
[a3]	M. Biliotti, N.L. Johnson, "Variations on a theme of Dembowski" , Proc. Amer. Math. Soc. Conf. Iowa City (l996)
[a4]	J.A. Thas, "Flocks, maximal exterior sets and inversive planes" Contemp. Math. , 111 (1990) pp. 187–218
[a5]	L. Bader, G. Lunardon, "On the flocks of 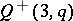" Geom. Dedicata , 29 (1989) pp. 177–183
[a6]	N.L. Johnson, "Flocks of infinite hyperbolic quadrics" J. Algebraic Combinatorics , 1 (1997) pp. 27–51
[a7]	J.A. Thas, "Generalized quadrangles and flocks of cones" Europ. J. Comb. , 8 (1987) pp. 441–452
[a8]	M. Biliotti, N.L. Johnson, "Bilinear flocks of quadratic cones" J. Geom. (to appear)
[a9]	V. Jha, N.L. Johnson, "Structure theory for point-Baer and line-Baer collineation groups in affine planes" , Proc. Amer. Math. Soc. Conf. Iowa City (l996)
[a10]	N.L. Johnson, "Flocks of hyperbolic quadrics and translation planes admitting affine homologies" J. Geom. , 34 (1989) pp. 50–73
[a11]	S.E. Payne, J.A. Thas, "Conical flocks, partial flocks, derivation and generalized quadrangles" Geom. Dedicata , 38 (1991) pp. 229–243