Completely-simple semi-group
One of the more important types of simple semi-groups. A semi-group  is called completely simple (completely
is called completely simple (completely  -simple) if it is simple (
-simple) if it is simple ( -simple) and contains a primitive idempotent, i.e. a non-zero idempotent that is not an identity for any non-zero idempotent of
-simple) and contains a primitive idempotent, i.e. a non-zero idempotent that is not an identity for any non-zero idempotent of 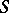 . If a zero is added to a completely-simple semi-group it becomes a completely
. If a zero is added to a completely-simple semi-group it becomes a completely  -simple semi-group; for this reason, many properties of completely-simple semi-groups may be deduced directly from the corresponding properties of completely
-simple semi-group; for this reason, many properties of completely-simple semi-groups may be deduced directly from the corresponding properties of completely  -simple semi-groups.
-simple semi-groups.
A semi-group 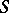 is completely
is completely  -simple if and only if it is
-simple if and only if it is  -simple and satisfies one of the following conditions: 1)
-simple and satisfies one of the following conditions: 1) 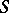 has minimal non-zero left and right ideals; or 2) some power of each element of
has minimal non-zero left and right ideals; or 2) some power of each element of 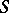 belongs to a subgroup of
belongs to a subgroup of 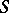 . In particular, any periodic (and, a fortiori, finite)
. In particular, any periodic (and, a fortiori, finite)  -simple semi-group will be a completely
-simple semi-group will be a completely  -simple semi-group. Any completely
-simple semi-group. Any completely  -simple semi-group is an O-bisimple regular semi-group and is the union of its
-simple semi-group is an O-bisimple regular semi-group and is the union of its  -minimal left (right) ideals. A semi-group
-minimal left (right) ideals. A semi-group 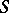 is a completely-simple semi-group if and only if it satisfies one of the following conditions: 1)
is a completely-simple semi-group if and only if it satisfies one of the following conditions: 1) 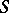 is a rectangular band of isomorphic groups (cf. Band of semi-groups); or 2)
is a rectangular band of isomorphic groups (cf. Band of semi-groups); or 2) 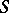 is regular and all its idempotents are primitive. A special kind of completely-simple semi-groups is the rectangular group which is the direct product of a group and a rectangular band (cf. Idempotents, semi-group of). A right group (left group) is in turn a special case of a rectangular semi-group. Rees' theorem gives an important representation of completely
is regular and all its idempotents are primitive. A special kind of completely-simple semi-groups is the rectangular group which is the direct product of a group and a rectangular band (cf. Idempotents, semi-group of). A right group (left group) is in turn a special case of a rectangular semi-group. Rees' theorem gives an important representation of completely  -simple semi-groups: A semi-group is a completely
-simple semi-groups: A semi-group is a completely  -simple semi-group if and only if it is isomorphic to a regular Rees semi-group of matrix type over a group with zero.
-simple semi-group if and only if it is isomorphic to a regular Rees semi-group of matrix type over a group with zero.
The study of finite completely-simple semi-groups formed the starting point of the development of the theory of semi-groups (cf. Semi-group). Completely  -simple and completely-simple semi-groups frequently appear in various theoretical investigations on semi-groups and are one of the most thoroughly studied types of semi-groups.
-simple and completely-simple semi-groups frequently appear in various theoretical investigations on semi-groups and are one of the most thoroughly studied types of semi-groups.
References
| [1] | A.H. Clifford, G.B. Preston, "Algebraic theory of semi-groups" , 1–2 , Amer. Math. Soc. (1961–1967) |
| [2] | E.S. Lyapin, "Semigroups" , Amer. Math. Soc. (1974) (Translated from Russian) |
| [3] | K. Kapp, H. Schneider, "Completely 0-simple semigroups: an abstract treatment of the lattice of congruences" , Benjamin (1969) |
Comments
A semi-group  is called simple (
is called simple ( -simple) if it has no proper ideals (respectively, if its only proper ideal is
-simple) if it has no proper ideals (respectively, if its only proper ideal is 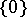 and
and 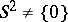 ) (cf. Simple semi-group). More accurately, a primitive idempotent is a non-zero idempotent
) (cf. Simple semi-group). More accurately, a primitive idempotent is a non-zero idempotent 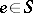 such that for any non-zero idempotent
such that for any non-zero idempotent  ,
, 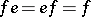 only if
only if 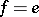 ( "e is not an identity for any f≠ e" ).
( "e is not an identity for any f≠ e" ).
A Rees semi-group of matrix type is often called a Rees matrix semi-group.
References
| [a1] | A.K. Suschkewitsch, "Ueber die endlichen Gruppen ohne das Gesetz der eindeutigen Umkehrbarkeit" Math. Ann. , 99 (1928) pp. 30–50 |
| [a2] | D. Rees, "On semi-groups" Proc. Cambridge Phil. Soc. , 36 (1940) pp. 387–400 |
Completely-simple semi-group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Completely-simple_semi-group&oldid=18594