Bredon cohomology
An ordinary equivariant cohomology for a finite group  , defined in [a1], on the category
, defined in [a1], on the category  -CW of
-CW of  -complexes (cf. Complex; CW-complex). The objects of
-complexes (cf. Complex; CW-complex). The objects of  -CW are the CW-complexes
-CW are the CW-complexes  with a cellular action of
with a cellular action of  , satisfying the condition that, for every subgroup
, satisfying the condition that, for every subgroup  of
of  , the fixed point set
, the fixed point set  is a subcomplex of
is a subcomplex of  . The morphisms are the cellular
. The morphisms are the cellular 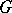 -mappings. Let
-mappings. Let  be the full subcategory of
be the full subcategory of  -CW whose objects are the
-CW whose objects are the  -orbits
-orbits 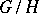 , where
, where  is a subgroup of
is a subgroup of  . For every contravariant functor
. For every contravariant functor  from
from  to the category
to the category 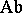 of Abelian groups, there exists a Bredon cohomology theory
of Abelian groups, there exists a Bredon cohomology theory  which, after restriction to
which, after restriction to 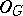 , vanishes for
, vanishes for  and is equal to
and is equal to  for
for  .
.
Let 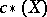 be the chain complex of functors from
be the chain complex of functors from  to
to 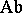 such that, for every subgroup
such that, for every subgroup  of
of  ,
,  is the ordinary cellular chain complex of
is the ordinary cellular chain complex of 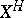 . Then
. Then
 |
where  denotes the set of natural transformations of functors. The functors
denotes the set of natural transformations of functors. The functors  are projective objects in the category of coefficient systems and there is a spectral sequence
are projective objects in the category of coefficient systems and there is a spectral sequence
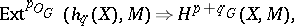 |
where  .
.
Let  be a
be a  -space with base point
-space with base point  (cf., e.g., Equivariant cohomology). Important examples of coefficient systems are the homotopy group functors
(cf., e.g., Equivariant cohomology). Important examples of coefficient systems are the homotopy group functors  defined by
defined by  . The obstruction theory for
. The obstruction theory for 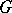 -mappings
-mappings  is formulated in terms of the cohomology groups
is formulated in terms of the cohomology groups  . For any coefficient system
. For any coefficient system  and natural number
and natural number  , there is a pointed Eilenberg–MacLane
, there is a pointed Eilenberg–MacLane  -complex
-complex  such that
such that 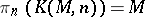 and
and  vanishes whenever
vanishes whenever  . For every
. For every  -complex
-complex 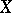 ,
,  , where
, where  denotes
denotes  -homotopy classes of
-homotopy classes of  -mappings.
-mappings.
If 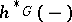 is an equivariant cohomology theory defined on the category
is an equivariant cohomology theory defined on the category  -CW, then there exists an Atiyah–Hirzebruch-type spectral sequence
-CW, then there exists an Atiyah–Hirzebruch-type spectral sequence
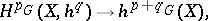 |
where 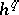 is the restriction of
is the restriction of  to
to  . Bredon cohomology for an arbitrary topological group is studied in [a4] and [a5]. Singular ordinary equivariant cohomology is defined in [a2] (the finite case) and in [a3]. If a coefficient system
. Bredon cohomology for an arbitrary topological group is studied in [a4] and [a5]. Singular ordinary equivariant cohomology is defined in [a2] (the finite case) and in [a3]. If a coefficient system  is a Mackey functor, then the Bredon cohomology
is a Mackey functor, then the Bredon cohomology  can be extended to an ordinary
can be extended to an ordinary  -graded cohomology [a6].
-graded cohomology [a6].
References
| [a1] | G.E. Bredon, "Equivariant cohomology theories" , Lecture Notes in Mathematics , 34 , Springer (1967) |
| [a2] | T. Bröcker, "Singuläre Definition der äquivarianten Bredon Homologie" Manuscr. Math. , 5 (1971) pp. 91–102 |
| [a3] | S. Illman, "Equivariant singular homology and cohomology" , Memoirs , 156 , Amer. Math. Soc. (1975) |
| [a4] | T. Matumoto, "Equivariant cohomology theories on 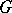 -CW-complexes" Osaka J. Math. , 10 (1973) pp. 51–68 -CW-complexes" Osaka J. Math. , 10 (1973) pp. 51–68 |
| [a5] | S.J. Wilson, "Equivariant homology theories on 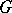 -complexes" Trans. Amer. Math. Soc. , 212 (1975) pp. 155–171 -complexes" Trans. Amer. Math. Soc. , 212 (1975) pp. 155–171 |
| [a6] | L.G. Lewis, J.P. May, J. McClure, "Ordinary RO(G)-graded cohomology" Bull. Amer. Math. Soc. , 4 (1981) pp. 208–212 |
Bredon cohomology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bredon_cohomology&oldid=16015