Boltzmann weight
According to statistical mechanics, the probability that a system in thermal equilibrium occupies a state with the energy 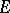 is proportional to
is proportional to 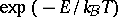 , where
, where 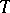 is the absolute temperature and
is the absolute temperature and 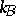 is the Boltzmann constant. Of course,
is the Boltzmann constant. Of course, 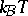 has a dimension of energy. The exponential
has a dimension of energy. The exponential 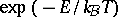 is called the Boltzmann weight.
is called the Boltzmann weight.
L. Boltzmann considered a gas of identical molecules which exchange energy upon colliding but otherwise are independent of each other. An individual molecule of such a gas does not have a constant velocity, so that no exact statement can be made concerning its state at a particular time. However, when the gas comes to equilibrium at some fixed temperature, one can make predictions about the average fraction of molecules which are in a given state. These average fractions are equivalent to probabilities and therefore the probability distribution for a molecule over its possible states can be introduced. Let the set of energies available to each molecule be denoted by 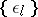 . The probability,
. The probability, 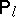 , of finding a molecule in the state
, of finding a molecule in the state 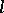 with the energy
with the energy  is
is
 | (a1) |
This is called the Boltzmann distribution.
J.W. Gibbs introduced the concept of an ensemble (cf. also Gibbs statistical aggregate), which is defined as a set of a very large number of systems, all dynamically identical with the system under consideration. The ensemble, also called the canonical ensemble, describes a system which is not isolated but which is in thermal contact with a heat reservoir. Since the system exchanges energy with the heat reservoir, the energy of the system is not constant and can be described by a probability distribution. Gibbs proved that the Boltzmann distribution holds not only for a molecule, but also for a system in thermal equilibrium. The probability  of finding a system in a given energy
of finding a system in a given energy 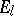 is
is
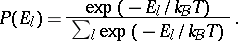 | (a2) |
With this extension, the Boltzmann distribution is extremely useful in investigating the equilibrium behaviour of a wide range of both classical and quantum systems [a1], [a2], [a3].
There are many examples of Boltzmann weights; some old and new ones are discussed below.
Free particle in thermal equilibrium.
Consider the momentum distribution for a free particle in thermal equilibrium. The particle has a (kinetic) energy 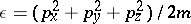 , with
, with 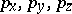 the Cartesian components of momentum. The probability
the Cartesian components of momentum. The probability 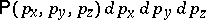 to find its momenta in the range between
to find its momenta in the range between 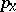 and
and 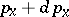 ,
, 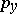 and
and 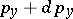 ,
, 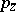 and
and 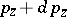 is
is
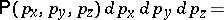 | (a3) |
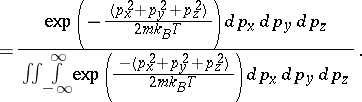 |
Equation (a3) is called the Maxwell distribution, after J.C. Maxwell, who obtained it before Boltzmann's more general derivation in 1871.
Models on a  -dimensional square lattice.
-dimensional square lattice.
There are two types of statistical-mechanics models, the vertex model and the  model (interaction round a face model) [a4].
model (interaction round a face model) [a4].
Vertex models.
State variables are located on the edges between two nearest neighbouring lattice points (vertices). One associates the Boltzmann weight with each vertex configuration. The configuration is defined by the state variables, say 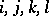 (placed anti-clockwise starting from the West), on the four edges joining together at the vertex. One denotes the energy and the Boltzmann weight of the vertex by
(placed anti-clockwise starting from the West), on the four edges joining together at the vertex. One denotes the energy and the Boltzmann weight of the vertex by 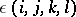 and
and 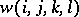 , respectively,
, respectively,
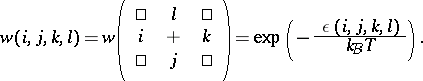 | (a4) |
 models.
models.
State variables are located on the lattice points. The Boltzmann weight is assigned to each unit face (plaquette) depending on the state variable configuration round the face. By  , one denotes the energy of a face with state variable configuration
, one denotes the energy of a face with state variable configuration 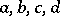 (placed anti-clockwise from the South-West). The corresponding Boltzmann weight is
(placed anti-clockwise from the South-West). The corresponding Boltzmann weight is
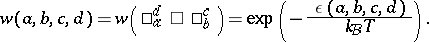 | (a5) |
General.
When one can evaluate thermodynamic quantities such as the free energy and the one-point function without any approximation, one says that the model is exactly solvable. The  -vertex model, the
-vertex model, the 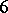 -vertex model, the (
-vertex model, the ( -dimensional) Ising model, and the hard-hexagon model are typical examples of exactly solvable models. For both vertex and IRF models, a sufficient condition for solvability is the Yang–Baxter relation, which ensures the existence of a commuting family of transfer matrices. The Yang–Baxter relation for the Boltzmann weights has been a source of recent developments in mathematical physics [a5]:
-dimensional) Ising model, and the hard-hexagon model are typical examples of exactly solvable models. For both vertex and IRF models, a sufficient condition for solvability is the Yang–Baxter relation, which ensures the existence of a commuting family of transfer matrices. The Yang–Baxter relation for the Boltzmann weights has been a source of recent developments in mathematical physics [a5]:
By solving the relation, one finds new solvable (vertex or  ) models. The models are solvable even at off-criticality and give complimentary information with conformal field theory which describes the phenomena just on criticality.
) models. The models are solvable even at off-criticality and give complimentary information with conformal field theory which describes the phenomena just on criticality.
The commuting family of transfer matrices gives a unified viewpoint on the solvable models in physics,  -dimensional field theory and
-dimensional field theory and  -dimensional statistical mechanics.
-dimensional statistical mechanics.
The Yang–Baxter relation leads to new mathematical objects, such as quantum groups [a6], [a7] and new link invariants [a8], [a9].
References
| [a1] | R.C. Tolman, "The principles of statistical mechanics" , Oxford Univ. Press (1938) (Reprint: 1980) |
| [a2] | F. Reif, "Statistical and thermal physics" , McGraw-Hill (1965) |
| [a3] | R. Kubo, et al., "Statistical physics" , 1–2 , Springer (1985) |
| [a4] | R.J. Baxter, "Exactly solved models in statistical mechanics" , Acad. Press (1992) |
| [a5] | "Yang–Baxter equation in integrable systems" M. Jimbo (ed.) , World Sci. (1990) |
| [a6] | M. Jimbo, "A 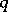 -difference analogue of -difference analogue of 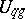 and the Yang–Baxter equation" Lett. Math. Phys. , 10 (1985) pp. 63–69 and the Yang–Baxter equation" Lett. Math. Phys. , 10 (1985) pp. 63–69 |
| [a7] | V.G. Drinfel'd, "Hopf algebras and the quantum Yang–Baxter equation" Soviet Math. Dokl. , 32 (1985) pp. 254–258 (In Russian) |
| [a8] | M. Wadati, T. Deguchi, Y Akutsu, "Exactly solvable models and knot theory" Physics Reports , 180 (1989) pp. 247–332 |
| [a9] | "Braid group, knot theory and statistical mechanics" C.N. Yang (ed.) M.L. Ge (ed.) , 1–2 , World Sci. (1989; 1994) |
Boltzmann weight. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Boltzmann_weight&oldid=16567