Infinite-dimensional space

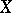A normal -space (cf. Normal space) such that for no 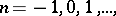the inequality 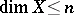is satisfied, i.e. 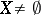and for any it is possible to find a finite open covering 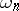of 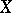such that every finite covering refining 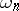has multiplicity 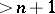. Examples of infinite-dimensional spaces are the Hilbert cube 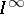and the Tikhonov cube 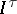. Most of the spaces encountered in functional analysis are also infinite-dimensional.

A normal 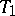-space 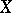is said to be infinite-dimensional in the sense of the large (small) inductive dimension if the inequality () is invalid for every 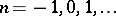. If 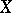is an infinite-dimensional space, it is infinite-dimensional in the sense of the large inductive dimension. If in addition is compact, it is also infinite-dimensional in the sense of the small inductive dimension. The infinite dimensionality of a metric space is equivalent with its infinite dimensionality in the sense of the large inductive dimension. There exist finite-dimensional compacta that are infinite-dimensional in the sense of the small (and hence also in the sense of the large) inductive dimension. It is not known (1986) whether or not a compactum (or a metric space) that is finite-dimensional in the sense of the small inductive dimension and infinite-dimensional in the sense of the large inductive dimension exists.

One of the most natural approaches to the study of infinite-dimensional spaces is to introduce the small transfinite dimension 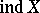and the large transfinite dimension 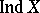. This approach consists in the extension of the definition of small and large inductive dimensions to infinite ordinal numbers. The transfinite dimensions and are not defined for all infinite-dimensional spaces. Thus, neither is defined for the Hilbert cube. The large transfinite dimension is not defined for the space 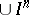, which is the discrete sum of the -dimensional cubes 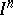, 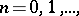but .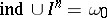

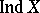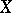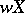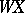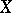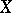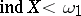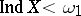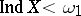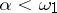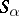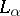If the transfinite dimension () is defined for a normal space , then it is equal to an ordinal number whose cardinality does not exceed the weight (respectively, the large weight ) of . In particular, if has a countable base, then , and if is compact, then as well. For metric spaces, too, . If , then there exist compacta and for which 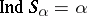, 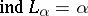. For any ordinal number 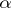there exists a metric space 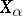with .

If the transfinite dimension 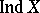is defined, the transfinite dimension 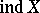is defined as well, and 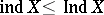. Metric compacta for which the transfinite dimension 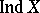is defined and for which 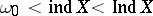, have also been constructed.

If the transfinite dimension (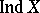) of a space 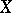is defined, then also the transfinite dimension 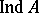(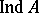) is defined for any (respectively, any closed) set 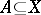, and the inequality (or 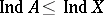) is valid.

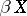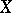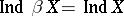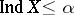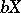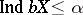For the maximal compactification of a normal space the equality is valid. A normal space of weight and of transfinite dimension has a compactification of weight and dimension . There exists a space with a countable base having dimension 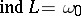for which no compactification 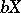with a countable base has dimension 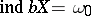. A metrizable space 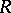of transfinite dimension 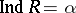has a metric such that the completion 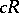with respect to it has dimension 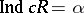. A metrizable space 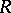of transfinite dimension 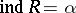with a countable base has a metric such that the completion 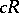with respect to it has dimension .

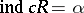The class of spaces for which a large or a small transfinite dimension is defined is closely connected with the class of metric countable-dimensional spaces; if a complete metric space is countable-dimensional, then the small transfinite dimension is defined for it; if the small transfinite dimension is defined for a metric space with a countable base, the space is countable-dimensional; if for a metric space the large transfinite dimension is defined (in particular if the space is finite-dimensional), then the space is countable-dimensional; the large transfinite dimension is defined for a countable-dimensional metric compactum. The space 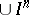is countable-dimensional and is infinite-dimensional. The Hilbert cube is not countable-dimensional.

Countable dimensionality of a metric space 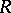is equivalent to any one of the following properties: a) there exists a finite-to-one (but, in general, not a 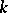-to-one for any 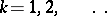) continuous closed mapping of a zero-dimensional metric space onto 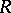; b) there exists a countable-to-one continuous closed mapping of a zero-dimensional metric space onto 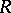; and c) 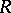is a countably zero-dimensional space.

Theorems about the representability of any -dimensional metric space as a sum of 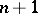zero-dimensional subsets or as the image of a zero-dimensional metric space under a continuous closed 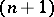-to-one mapping indicate that it is natural to consider the class of countable-dimensional (metric) spaces and that it is close to the class of finite-dimensional spaces. As in the finite-dimensional case, there exists a countable-dimensional space which is universal in the sense of homeomorphic imbedding in the class of countable-dimensional metric spaces of weight .

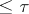If a normal space is represented as a finite or a countable sum of its countable-dimensional subspaces, then it is countable-dimensional. A subspace of a countable-dimensional perfectly-normal space is countable-dimensional.

The following theorem describes the relationships between countable and non-countable dimensional metric spaces: If a mapping 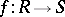between metric spaces and 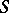is continuous and closed, if the space is countable-dimensional and the space is non-countable dimensional, then the set is also non-countable dimensional.

In addition to countable-dimensional spaces, a natural extension of the class of finite-dimensional spaces is the class of weakly countable-dimensional spaces. If one considers metrizable spaces only, weakly countable-dimensional spaces occupy a place which is intermediate between finite-dimensional and countable-dimensional spaces. There exist countable-dimensional metric compacta that are not weakly countable-dimensional, while the space 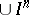is both weakly countable-dimensional and infinite-dimensional. A closed subspace of a weakly countable-dimensional space is weakly countable-dimensional. A normal space is weakly countable-dimensional if it is representable as a finite or a countable sum of its weakly countable-dimensional closed subsets.

In the classes of normal weakly countable-dimensional and metric weakly countable-dimensional spaces there exist universal (in the sense of homeomorphic imbedding) spaces. In the case of spaces with a countable base, an example is the subspace of the Hilbert cube which consists of all points with only a finite number of non-zero coordinates. The space 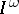has no weakly countable-dimensional compactifications.

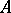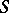All classes of infinite-dimensional spaces considered so far are "not very infinite-dimensional" as compared with, for example, the Hilbert cube. The problem of distinguishing "not very infinite-dimensional" from "very infinite-dimensional" spaces was solved by P.S. Aleksandrov and Yu.M. Smirnov, who introduced the classes of - and -weakly infinite-dimensional and of 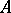- and 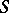-strongly infinite-dimensional normal spaces. Any finite-dimensional space is 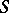-weakly infinite-dimensional, while any 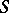-weakly infinite-dimensional space is also 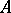-weakly infinite-dimensional. The space is 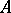-weakly infinite-dimensional, but 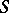-strongly infinite-dimensional.

In the case of compacta the definitions of 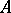- and 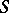-weak (strong) infinite dimensionality are equivalent, and for this reason 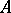-weakly (strongly) infinite-dimensional compacta are simply called strongly (weakly) infinite-dimensional. The Hilbert cube is strongly infinite-dimensional. There exist infinite-dimensional and weakly infinite-dimensional compacta.

A closed subspace of an - (-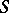) weakly infinite-dimensional space is - (-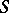) weakly infinite-dimensional. A normal space which is the sum of a finite number of its closed 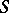-weakly infinite-dimensional sets, is itself -weakly infinite-dimensional. A paracompactum which is the sum of a finite or countable system of its closed -weakly infinite-dimensional sets is itself 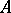-weakly infinite-dimensional. A hereditarily-normal space which is the sum of a finite or countable system of its -weakly infinite-dimensional sets is itself 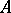-weakly infinite-dimensional.

A weakly countable-dimensional paracompactum is 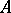-weakly infinite-dimensional. A hereditarily-normal countable-dimensional space is 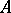-weakly infinite-dimensional. A weakly infinite-dimensional, not countable-dimensional metric compactum has been constructed by R. Pol [3].

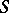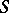The study of arbitrary -weakly infinite-dimensional metrizable spaces is reduced to the compact case by the following: A metrizable space is -weakly infinite-dimensional if and only if it can be represented as a sum of a weakly infinite-dimensional compactum and finite-dimensional open sets 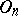, such that for any discrete sequence of points

there exists a set (depending on the sequence) containing all the points 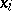, beginning with some such point.

The following theorems provide another way of studying infinite-dimensional compacta instead of arbitrary 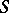-weakly infinite-dimensional spaces: The maximal compactification of an 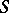-weakly infinite-dimensional space is weakly infinite-dimensional; any normal 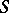-weakly infinite-dimensional space of weight has a weakly infinite-dimensional compactification of weight . All compactifications of the -weakly infinite-dimensional space 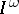are strongly infinite-dimensional.

A compactum is strongly infinite-dimensional if and only if there exists a continuous mapping such that for any set

(which is homeomorphic to an -dimensional cube) the restriction of the mapping 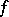to the inverse image is an essential mapping.

There exists an infinite-dimensional metric compactum any non-empty subspace of which is either zero-dimensional or infinite-dimensional. Moreover, any strongly infinite-dimensional metric compactum contains a subcompactum any non-empty subspace of which is either zero-dimensional or infinite-dimensional. Any strongly infinite-dimensional compactum contains an infinite-dimensional Cantor manifold.

All separable Banach spaces are mutually homeomorphic, 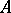-strongly infinite-dimensional and homeomorphic to the product of a countable system of straight lines.

References

[1]	P.S. Aleksandrov, B.A. Pasynkov, "Introduction to dimension theory" , Moscow (1973) (In Russian)
[2]	R. Engelking, "Transfinite dimension" G.M. Reed (ed.) , Surveys in general topology , Acad. Press (1980) pp. 131–161
[3]	R. Pol, "A weakly infinite-dimensional compactum which is not countable dimensional" Proc. Amer. Math. Soc. , 82 (1981) pp. 634–636

Comments

A space is called a countable-dimensional space if it can be written as the union of a countable family of finite-dimensional subsets, see also Countably zero-dimensional space.

References

[a1]	R. Engelking, "Dimension theory" , North-Holland & PWN (1978)
[a2]	R. Engelking, E. Pol, "Countable dimensional spaces: a survey" Diss. Math. , 216 (1983) pp. 1–41
[a3]	L.A. Ljuksemburg, "On compact metric spaces with non-coinciding transfinite dimensions" Pac. J. Math. , 93 (1981) pp. 339–386
[a4]	C. Bessaga, A. Pelczyński, "Selected topics in infinite-dimensional topology" , PWN (1975)