Additive category

A category in which for any two objects and an Abelian group structure is defined on the set of morphisms , such that the composition of morphisms

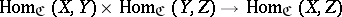is a bilinear mapping. Another necessary condition is that includes a null object (zero object, cf. Null object of a category) as well as the product of any two objects and .

In an additive category the direct sum 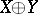of any two objects exists. It is isomorphic to their product 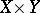. The dual category to an additive category is also an additive category.

A functor 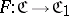from an additive category 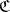into an additive category 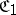is said to be additive if, for any objects 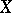and in 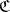, the mapping 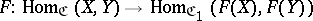is a homomorphism of Abelian groups. An additive category is said to be pre-Abelian if a kernel (cf. Kernel of a morphism in a category) and a cokernel exist for any morphism.

If for a morphism 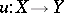in an additive category there exists an image 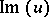and a co-image 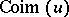, then there exists a unique morphism 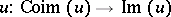such that the morphism splits as the composition

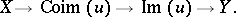An Abelian category is additive by definition. Examples of non-Abelian additive categories are the category of topological modules over a given topological ring with respect to the morphisms which are continuous linear mappings, and also the category of Abelian groups with a filtration with respect to the morphisms which are group homomorphisms preserving the filtration.

References

[1]	I. Bucur, A. Deleanu, "Introduction to the theory of categories and functors" , Wiley (1968)
[2]	A. Grothendieck, "Sur quelques points d'algèbrique homologique" Tohôku Math. J. , 9 (1957) pp. 119–221
[3]	L. Gruson, "Complétion abélienne" Bull. Sci. Math. (2) , 90 (1966) pp. 17–40

Comments

The requirement, in the definition of an additive category 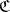above, that 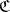possesses a null object as well as the product of any two objects in it, is not standard. Most authors drop this requirement, and take an additive category to mean a category satisfying only the requirement in the first sentence of the main text above.