Schottky theorem
If a function
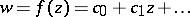 | (*) |
is regular and analytic in the disc  and does not take certain finite values
and does not take certain finite values 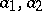 in
in 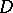 , then in any disc
, then in any disc  ,
,  , the modulus
, the modulus 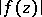 is bounded by a number
is bounded by a number 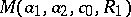 that depends only on
that depends only on 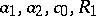 (see [1]). A more complete formulation can be obtained, by combining the generalized Schottky theorem and Landau's theorem, for an arbitrary number
(see [1]). A more complete formulation can be obtained, by combining the generalized Schottky theorem and Landau's theorem, for an arbitrary number  of exceptional values. Suppose that the function (*) does not take some finite values
of exceptional values. Suppose that the function (*) does not take some finite values  ,
, 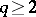 . Then for
. Then for 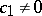 , the radius
, the radius  is bounded above by a number that depends only on
is bounded above by a number that depends only on 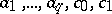 (Landau's theorem). Moreover, in the disc
(Landau's theorem). Moreover, in the disc  ,
,  , the modulus
, the modulus 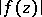 is bounded by a number
is bounded by a number 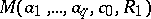 that depends only on
that depends only on  (Schottky's theorem).
(Schottky's theorem).
From the geometrical point of view Schottky's theorem means that the spherical distance (i.e. the distance on the Riemann sphere) of the image of the disc  from the points
from the points 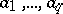 is no less than a number
is no less than a number 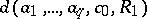 that depends only on
that depends only on 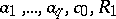 . Schottky's theorem is one of the classical results in the theory of functions of a complex variable of the type of distortion theorems.
. Schottky's theorem is one of the classical results in the theory of functions of a complex variable of the type of distortion theorems.
References
| [1] | F. Schottky, "Ueber den Picard'schen Satz und die Borel'schen Ungleichungen" Sitzungsber. Preuss. Akad. Wiss. , 2 (1904) pp. 1244–1262 |
| [2] | G.M. Goluzin, "Geometric theory of functions of a complex variable" , Transl. Math. Monogr. , 26 , Amer. Math. Soc. (1969) (Translated from Russian) |
| [3] | S. Stoilov, "The theory of functions of a complex variable" , 1–2 , Moscow (1962) (In Russian; translated from Rumanian) |
Comments
The theorems of Landau and Schottky are also related to the Picard theorem.
References
| [a1] | E. Landau, D. Gaier, "Darstellung und Begrundung einiger neuerer Ergebnisse der Funktionentheorie" , Springer, reprint (1986) |
| [a2] | J.B. Conway, "Functions of one complex variable" , Springer (1978) |
Schottky theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Schottky_theorem&oldid=18792