Hopf manifold

A complex Hopf manifold is a quotient of by the infinite cyclic group of holomorphic transformations generated by , , . It is usually denoted by 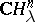. As a differentiable manifold, any is diffeomorphic to . Consequently, when the first Betti number ; hence, such provide examples of compact complex manifolds (cf. Complex manifold) not admitting any Kähler metric (note that, for , is a -dimensional complex torus). However, these manifolds do carry a particularly interesting class of Hermitian metrics (cf. Hermitian metric), namely locally conformal Kähler metrics (a Hermitian metric is locally conformal Kähler if its fundamental -form satisfies the integrability condition 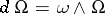with a closed 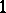-form , called the Lee form). An example of such a metric is the projection of the following metric on : , whose Lee form is . 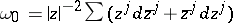It is parallel with respect to the Levi-Civita connection of . This originated the study of the more general class of generalized Hopf manifolds: locally conformal Kähler manifolds with parallel Lee form. Their geometry is closely related to Sasakian and Kählerian geometries. Generically, a compact generalized Hopf manifold arises as the total space of a flat, principal bundle over a compact Sasakian orbifold and, on the other hand, fibres into -dimensional complex tori over a Kähler orbifold.

I. Vaisman conjectured that a compact locally conformal Kähler manifold that is not globally conformal Kähler must have an odd Betti number. To date (1996), this has only been proved for generalized Hopf manifolds (see [a5]).

It is rather difficult to characterize the Hopf manifolds among the locally conformal Kähler manifolds. However, in complex dimension several such characterizations are available; for instance, the only compact complex surface with and admitting conformally flat Hermitian metrics is 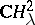(cf. [a4]; see also [a1]).

By analogy, "real Hopf manifoldreal Hopf manifolds" were defined as (compact) quotients of by an appropriate group of conformal transformations (see [a6] and [a2]). Similarly, "quaternion Hopf manifoldquaternion Hopf manifolds" are defined as quotients of (see [a3]).

References

[a1]	C.P. Boyer, "Conformal duality and compact complex surfaces" Math. Ann. , 274 (1986) pp. 517–526
[a2]	P. Gauduchon, "Structures de Weyl–Einstein, espaces de twisteurs et variétés de type 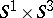" J. Reine Angew. Math. , 455 (1995) pp. 1–50
[a3]	L. Ornea, P. Piccinni, "Locally conformal Kähler structures in quaternionic geometry" Trans. Amer. Math. Soc. , 349 (1997) pp. 641–655
[a4]	M. Pontecorvo, "Uniformization of conformally flat Hermitian surfaces" Diff. Geom. Appl. , 3 (1992) pp. 295–305
[a5]	I. Vaisman, "Generalized Hopf manifolds" Geom. Dedicata , 13 (1982) pp. 231–255
[a6]	I. Vaisman, C. Reischer, "Local similarity manifolds" Ann. Mat. Pura Appl. , 35 (1983) pp. 279–292
[a7]	S. Dragomir, L. Ornea, "Locally conformal Kähler geometry" , Birkhäuser (1997)