Negative binomial distribution

A probability distribution of a random variable 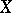which takes non-negative integer values 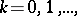in accordance with the formula

(*)

for any real values of the parameters and . The generating function and the characteristic function of a negative binomial distribution are defined by the formulas

and

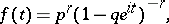respectively, where . The mathematical expectation and variance are equal, respectively, to 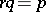and 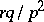. The distribution function of a negative binomial distribution for the values 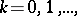is defined in terms of the values of the beta-distribution function at a point 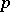by the following relation:

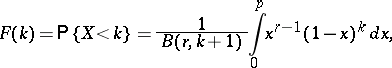where 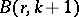is the beta-function.

The origin of the term "negative binomial distribution" is explained by the fact that this distribution is generated by a binomial with a negative exponent, i.e. the probabilities (*) are the coefficients of the expansion of 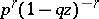in powers of .

Negative binomial distributions are encountered in many applications of probability theory. For an integer 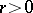, the negative binomial distribution is interpreted as the distribution of the number of failures before the -th "success" in a scheme of Bernoulli trials with probability of "success" 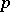; in this context it is usually called a Pascal distribution and is a discrete analogue of the gamma-distribution. When 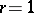, the negative binomial distribution coincides with the geometric distribution. The negative binomial distribution often appears in problems related to the randomization of the parameters of a distribution; for example, if 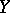is a random variable having, conditionally on , a Poisson distribution with random parameter 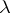, which in turn has a gamma-distribution with density

then the marginal distribution of 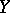will be a negative binomial distribution with parameters 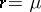and 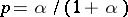. The negative binomial distribution serves as a limiting form of a Pólya distribution.

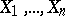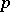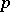The sum of independent random variables which have negative binomial distributions with parameters and , respectively, has a negative binomial distribution with parameters and 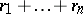. For large and small 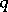, where , the negative binomial distribution is approximated by the Poisson distribution with parameter 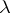. Many properties of a negative binomial distribution are determined by the fact that it is a generalized Poisson distribution.

References

[1]	W. Feller, "An introduction to probability theory and its applications" , 1–2 , Wiley (1950–1966)

Comments

See also Binomial distribution.

References

[a1]	N.L. Johnson, S. Kotz, "Distributions in statistics, discrete distributions" , Wiley (1969)