Picard group
A group of classes of invertible sheaves (or line bundles). More precisely, let 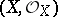 be a ringed space. A sheaf of
be a ringed space. A sheaf of 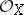 -modules
-modules 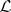 is called invertible if it is locally isomorphic to the structure sheaf
is called invertible if it is locally isomorphic to the structure sheaf 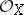 . The set of classes of isomorphic invertible sheaves on
. The set of classes of isomorphic invertible sheaves on 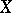 is denoted by
is denoted by  . The tensor product
. The tensor product 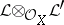 defines an operation on the set
defines an operation on the set 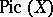 , making it an Abelian group called the Picard group of
, making it an Abelian group called the Picard group of 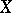 . The group
. The group  is naturally isomorphic to the cohomology group
is naturally isomorphic to the cohomology group 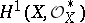 , where
, where  is the sheaf of invertible elements in
is the sheaf of invertible elements in 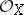 .
.
For a commutative ring 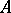 , the Picard group
, the Picard group 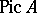 is the group of classes of invertible
is the group of classes of invertible 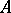 -modules;
-modules; 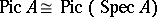 . For a Krull ring, the group
. For a Krull ring, the group  is closely related to the divisor class group for this ring.
is closely related to the divisor class group for this ring.
The Picard group of a complete normal algebraic variety 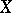 has a natural algebraic structure (see Picard scheme). The reduced connected component of the zero of
has a natural algebraic structure (see Picard scheme). The reduced connected component of the zero of  is denoted by
is denoted by  and is called the Picard variety for
and is called the Picard variety for  ; it is an algebraic group (an Abelian variety if
; it is an algebraic group (an Abelian variety if  is a complete non-singular variety). The quotient group
is a complete non-singular variety). The quotient group 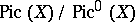 is called the Néron–Severi group and it has a finite number of generators; its rank is called the Picard number. In the complex case, where
is called the Néron–Severi group and it has a finite number of generators; its rank is called the Picard number. In the complex case, where 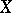 is a smooth projective variety over
is a smooth projective variety over  , the group
, the group 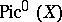 is isomorphic to the quotient group of the space
is isomorphic to the quotient group of the space 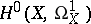 of holomorphic
of holomorphic 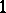 -forms on
-forms on 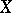 by the lattice
by the lattice 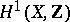 .
.
References
| [1] | D. Mumford, "Lectures on curves on an algebraic surface" , Princeton Univ. Press (1966) |
Comments
References
| [a1] | R. Hartshorne, "Algebraic geometry" , Springer (1977) pp. 91 |
Picard group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Picard_group&oldid=17920