Fourier coefficients
From Encyclopedia of Mathematics
The coefficients
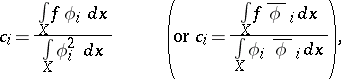 | (*) |
in the expansion of a function  defined on a space
defined on a space  with respect to an orthogonal system of real-valued (complex-valued) functions on
with respect to an orthogonal system of real-valued (complex-valued) functions on 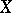 . If
. If 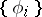 is an orthogonal system in a Hilbert (pre-Hilbert) space, then, given an element
is an orthogonal system in a Hilbert (pre-Hilbert) space, then, given an element 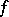 of this space, the numbers
of this space, the numbers 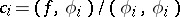 are also called the Fourier coefficients of
are also called the Fourier coefficients of 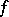 with respect to the system
with respect to the system 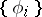 . J. Fourier first investigated trigonometric series with coefficients defined by (*).
. J. Fourier first investigated trigonometric series with coefficients defined by (*).
References
| [1] | S. Kaczmarz, H. Steinhaus, "Theorie der Orthogonalreihen" , Chelsea, reprint (1951) |
How to Cite This Entry:
Fourier coefficients. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fourier_coefficients&oldid=17816
Fourier coefficients. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fourier_coefficients&oldid=17816
This article was adapted from an original article by T.P. Lukashenko (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article