Almost-complex structure
A tensor field 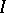 of linear transformations of the tangent spaces on a manifold
of linear transformations of the tangent spaces on a manifold 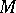 satisfying the condition
satisfying the condition
 |
i.e. a field of complex structures in the tangent spaces 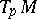 ,
, 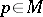 . An almost-complex structure
. An almost-complex structure 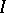 determines a decomposition
determines a decomposition 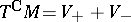 of the complexification
of the complexification 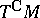 of the tangent bundle in a direct sum of two complex mutually-conjugate subbundles
of the tangent bundle in a direct sum of two complex mutually-conjugate subbundles 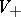 and
and 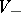 consisting of eigen vectors of the affinor
consisting of eigen vectors of the affinor 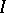 (extended by linearity on
(extended by linearity on  ) with eigen values
) with eigen values 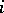 and
and  , respectively. Conversely, a decomposition of
, respectively. Conversely, a decomposition of 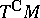 in a direct sum of mutually-conjugate vector subbundles
in a direct sum of mutually-conjugate vector subbundles 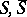 defines an almost-complex structure on
defines an almost-complex structure on 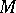 for which
for which 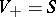 .
.
An almost-complex structure 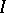 is called integrable if it is induced by a complex structure on
is called integrable if it is induced by a complex structure on 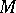 , i.e. if there exists an atlas of admissible charts of the manifold
, i.e. if there exists an atlas of admissible charts of the manifold 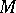 in which the field
in which the field 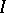 has constant coordinates
has constant coordinates  . A necessary and sufficient condition for the integrability of an almost-complex structure is that the subbundle
. A necessary and sufficient condition for the integrability of an almost-complex structure is that the subbundle 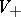 is involutive, i.e. that the space of its sections is closed with respect to commutation of (complex) vector fields. The condition for the subbundle
is involutive, i.e. that the space of its sections is closed with respect to commutation of (complex) vector fields. The condition for the subbundle  to be involutive is equivalent to the vanishing of the vector-valued
to be involutive is equivalent to the vanishing of the vector-valued  -form
-form 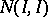 associated with
associated with  and given by the formula
and given by the formula
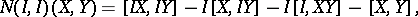 |
where  and
and 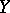 vector fields. This form is called the torsion tensor, or the Nijenhuis tensor, of the almost-complex structure. The torsion tensor
vector fields. This form is called the torsion tensor, or the Nijenhuis tensor, of the almost-complex structure. The torsion tensor 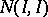 can be considered as first-order differentiation on the algebra of differential forms on
can be considered as first-order differentiation on the algebra of differential forms on 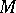 of the form
of the form
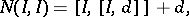 |
where 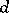 is the exterior differential and
is the exterior differential and 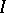 is considered as a differentiation of order zero.
is considered as a differentiation of order zero.
From the point of view of the theory of  -structures an almost-complex structure is a
-structures an almost-complex structure is a 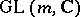 -structure, where
-structure, where 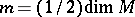 , and the torsion tensor
, and the torsion tensor  is the tensor defined by the first structure function of this structure. A
is the tensor defined by the first structure function of this structure. A  -structure is a structure of elliptic type, therefore the Lie algebra of infinitesimal automorphisms of an almost-complex structure satisfies a second-order system of elliptic differential equations [1]. In particular, the Lie algebra of infinitesimal automorphisms of an almost-complex structure on a compact manifold is finite-dimensional, and the group
-structure is a structure of elliptic type, therefore the Lie algebra of infinitesimal automorphisms of an almost-complex structure satisfies a second-order system of elliptic differential equations [1]. In particular, the Lie algebra of infinitesimal automorphisms of an almost-complex structure on a compact manifold is finite-dimensional, and the group  of all automorphisms of a compact manifold with an almost-complex structure is a Lie group. For non-compact manifolds this statement is, in general, not true.
of all automorphisms of a compact manifold with an almost-complex structure is a Lie group. For non-compact manifolds this statement is, in general, not true.
If the automorphism group 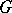 acts transitively on the manifold
acts transitively on the manifold  , then the almost-complex structure
, then the almost-complex structure 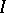 is uniquely defined by its value
is uniquely defined by its value 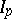 at a fixed point
at a fixed point 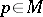 . This represents an invariant complex structure in the tangent space
. This represents an invariant complex structure in the tangent space 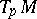 relative to the isotropic representation (see Invariant object on a homogeneous space). Methods of the theory of Lie groups allow one to construct a wide class of homogeneous spaces having an invariant almost-complex structure (both integrable and non-integrable) and to classify invariant almost-complex structures under different assumptions (see [2]). For instance, any quotient space
relative to the isotropic representation (see Invariant object on a homogeneous space). Methods of the theory of Lie groups allow one to construct a wide class of homogeneous spaces having an invariant almost-complex structure (both integrable and non-integrable) and to classify invariant almost-complex structures under different assumptions (see [2]). For instance, any quotient space  of a Lie group
of a Lie group 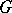 by the subgroup
by the subgroup 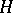 consisting of fixed points of an automorphism of even order of
consisting of fixed points of an automorphism of even order of 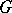 has an invariant almost-complex structure. An example is the
has an invariant almost-complex structure. An example is the 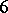 -dimensional sphere
-dimensional sphere 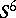 , considered as the homogeneous space
, considered as the homogeneous space 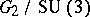 ; none of the invariant almost-complex structures on
; none of the invariant almost-complex structures on 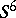 is integrable.
is integrable.
The existence of an almost-complex structure on a manifold imposes certain restrictions on its topology — it must be of even dimension, oriented, and in the compact case all its odd-dimensional Stiefel–Whitney classes must vanish. Among the spheres only the spheres of dimensions 2 and 6 admit an almost-complex structure.
References
| [1] | S. Kobayashi, "Transformation groups in differential geometry" , Springer (1972) |
| [2] | B.P. Komrakov, "Structure on manifolds and homogeneous spaces" , Minsk (1978) (In Russian) |
| [3] | A. Lichnerowicz, "Global theory of connections and holonomy groups" , Noordhoff (1976) (Translated from French) |
| [4] | R.O. Wells jr., "Differential analysis on complex manifolds" , Springer (1980) |
| [5] | L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973) |
Comments
The theorem that an almost-complex structure is integrable, i.e. comes from a complex structure, if and only if its Nijenhuis tensor vanishes, is due to A. Newlander and L. Nirenberg [a1].
References
| [a1] | A. Newlander, L. Nirenberg, "Complex analytic coordinates in almost complex manifolds" Ann. of Math. , 65 (1957) pp. 391–404 |
Almost-complex structure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Almost-complex_structure&oldid=17647