Poly-vector

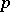-vector, over a vector space

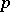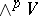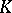An element of the -th exterior degree of the space over a field (see Exterior algebra). A -vector can be understood as a 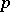-times skew-symmetrized contravariant tensor on 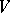. Any linearly independent system of vectors 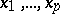from defines a non-zero -vector 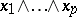; such a poly-vector is called factorable, decomposable, pure, or prime (often simply a poly-vector). Here two linearly independent systems 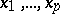and 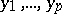generate the same subspace in 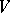if and only if 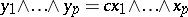, where 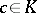. 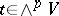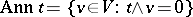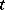For any non-zero poly-vector , its annihilator is a subspace of dimension , and the poly-vector is pure if and only if 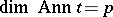. The pure 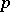-vectors of an -dimensional space form an algebraic variety in 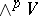; the corresponding projective algebraic variety is a Grassmann manifold. 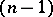Any non-zero -vector or -vector in an -dimensional space 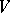is pure, but a bivector 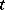is pure if and only if .

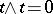If is a basis of 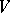and 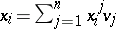, then the coordinates of the poly-vector 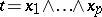in the basis 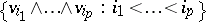of the space 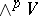are the minors 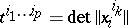, , 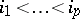of the matrix 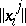. In particular, for ,

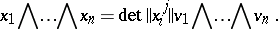If one specifies a non-zero -vector 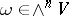, a duality between 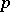-vectors and -vectors is obtained, i.e. a natural isomorphism

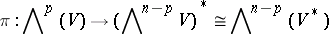such that 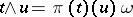for all and .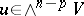

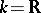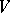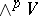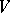Let and let an inner product be defined in , then in an inner product is induced with the following property: For any orthonormal basis in the basis 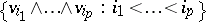in 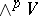is also orthonormal. The scalar square

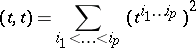of a pure poly-vector 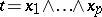coincides with the square of the volume of the parallelopipedon in 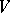constructed on the vectors 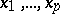. If one specifies an orientation in the -dimensional Euclidean space 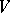(which is equivalent to choosing an -vector 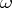for which 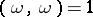), then the above duality leads to a natural isomorphism 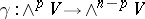. In particular, the 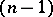-vector 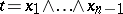corresponds to a vector , called the vector product of the vectors .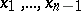

References

[1]	N. Bourbaki, "Elements of mathematics. Algebra: Algebraic structures. Linear algebra" , 1 , Addison-Wesley (1974) pp. Chapt.1;2 (Translated from French)
[2]	A.I. Kostrikin, Yu.I. Manin, "Linear algebra and geometry" , Gordon & Breach (1989) (Translated from Russian)
[3]	M.M. Postnikov, "Linear algebra and differential geometry" , Moscow (1979) (In Russian)