Feathering

of a space

A countable family 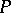of coverings of a space 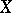by open sets in an ambient space 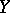such that

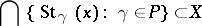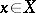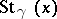for every point (here denotes the star of the point relative to 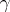, i.e. the union of all elements of 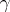containing the point ).

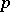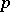The concept of a feathering forms the basis of the definition of the so-called -space (in the sense of A.V. Arkhangel'skii). A space is called a -space if it has a feathering in its Stone–Čech compactification or Wallman compactification. Every complete space (in the sense of Čech) is a -space. Every -space has pointwise countable type. In a -space, the addition theorem for weight holds and the net weight coincides with the weight. Paracompact 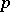-spaces are perfect pre-images of metric spaces. Paracompact 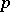-spaces with a pointwise countable base are metrizable, just as spaces of this type with a 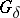-diagonal are also metrizable. The perfect image and the perfect pre-image of a paracompact -space are also paracompact 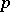-spaces.

Comments

The word "plumingpluming" is also used instead of feathering. A 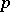-space is also called a feathered space.

References

[a1]	"Generalized metric spaces" K. Kunen (ed.) J.E. Vaughan (ed.) , Handbook of Set-Theoretic Topology , North-Holland (1984) pp. 423–501