Hyperbolic partial differential equation

at a given point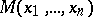

A partial differential equation for which the Cauchy problem is uniquely solvable for initial data specified in a neighbourhood of on any non-characteristic surface (cf. Characteristic surface). In particular, a partial differential equation for which the normal cone has no imaginary zones is a hyperbolic partial differential equation. The differential equation

(*)

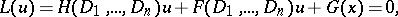where 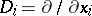(), 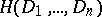is a homogeneous polynomial of degree , while the polynomial 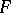is of lower degree than , is a hyperbolic partial differential equation if its characteristic equation

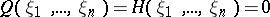has 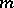different real solutions with respect to one of the variables 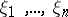, the remaining ones being fixed. Any equation (*) of the first order 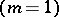with real coefficients is a hyperbolic partial differential equation. A second-order equation

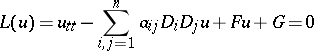is hyperbolic if the quadratic form

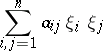is positive definite.

Comments

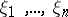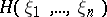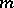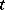The special variable among the such that has different real solutions for each set of fixed values of the other is often taken to be (time). One speaks then of a (strictly) hyperbolic equation or an equation of (strictly) hyperbolic type with respect to the 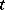-direction. More generally one considers hyperbolicity with respect to a vector 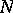[a1].

A polynomial of degree 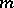with principal part 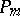is called hyperbolic with respect to the real vector 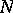if 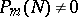and there exists a number 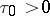such that

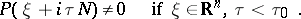If 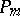is such that 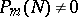and 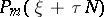has only simple real roots for every real 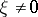, then 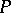is said to be strictly hyperbolic or hyperbolic in the sense of Petrovskii.

The Cauchy problem for a constant-coefficient differential operator 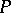with data on a non-characteristic plane is well posed for arbitrary lower-order terms if and only if 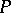is strictly hyperbolic. For a discussion of similar matters for polynomials with variable coefficients cf. [a2].

For a system of higher-order linear partial differential equations

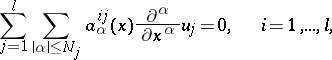where 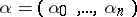, is a hyperbolic system of partial differential equations in the sense of Petrovskii if the determinant

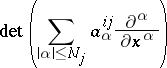calculated in the ring of differential operators is a hyperbolic polynomial in the sense of Petrovskii (as a polynomial of degree 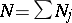). The Cauchy problem for a system that is hyperbolic in this sense is well posed [a3], [a4].

Instead of strictly hyperbolic one also finds the term strongly hyperbolic and instead of hyperbolic also weakly hyperbolic (which is therefore the case in which the lower-order terms of 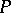do matter).

References

[a1]	L.V. Hörmander, "The analysis of linear partial differential operators" , 1 , Springer (1983) pp. Chapt. XII
[a2]	L.V. Hörmander, "The analysis of linear partial differential operators" , III , Springer (1985) pp. Chapt. XXIII
[a3]	I.G. Petrovskii, "Ueber das Cauchysche Problem für Systeme von partiellen Differentialgleichungen" Mat. Sb. (N.S.) , 2(44) (1937) pp. 815–870
[a4]	S. Mizohata, "The theory of partial differential equations" , Cambridge Univ. Press (1973) (Translated from Japanese)
[a5]	J. Chaillou, "Hyperbolic differential polynomials" , Reidel (1979)
[a6]	J. Chazarain, "Opérateurs hyperboliques à characteristique de multiplicité constante" Ann. Inst. Fourier , 24 (1974) pp. 173–202
[a7]	L. Gårding, "Linear hyperbolic equations with constant coefficients" Acta Math. , 85 (1951) pp. 1–62
[a8]	O.A. Oleinik, "On the Cauchy problem for weakly hyperbolic equations" Comm. Pure Appl. Math. , 23 (1970) pp. 569–586