Rank of an algebraic group

The dimension of a Cartan subgroup of it (this dimension does not depend on the choice of the Cartan subgroup). Along with the rank of an algebraic group 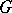one considers its semi-simple rank and reductive rank, which, by definition, are equal to the rank of the algebraic group 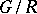and the rank of the algebraic group 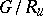respectively, where 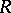is the radical of the algebraic group 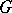and is its unipotent radical (cf. Radical of a group; Unipotent element). The reductive rank of an algebraic group 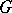is equal to the dimension of any of its maximal tori (cf. Maximal torus). The reductive 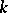-rank of a linear algebraic group 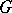defined over a field (and in the case when the group 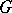is reductive (cf. Reductive group) — simply its 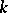-rank) is the dimension of a maximal 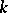-split torus of it (this dimension does not depend on the choice of a torus; see Split group). If the 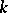-rank of a reductive linear algebraic group 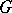over 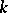is zero (is equal to the rank of 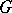), then the group 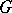is said to be anisotropic (or split, respectively) over 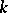(see also Anisotropic group).

Examples.

1) The rank of the algebraic group 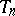of all non-singular upper-triangular square matrices of order is equal to its reductive rank and equal to ; the semi-simple rank of 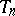is zero.

2) The rank of the algebraic group of all upper-triangular square matrices of order with 1 on the principal diagonal is equal to its dimension 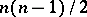, and the reductive and semi-simple ranks of 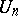are zero.

3) The rank of the algebraic group 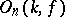of all -automorphisms of a definite quadratic form 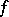on an -dimensional vector space over a field 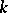is equal to 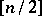, and the 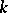-rank of the group 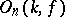is equal to the Witt index of the form .

If the characteristic of the ground field is 0, then the rank of the algebraic group coincides with the rank of its Lie algebra 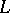(see Rank of a Lie algebra) and is equal to the minimum multiplicity of the eigen value 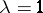of all possible adjoint operators 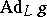(the minimum is taken over all 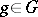). An element 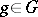for which this multiplicity is equal to the rank of the algebraic group is called regular. The set of regular elements of is open in the Zariski topology on .

References

[1]	C. Chevalley, "Théorie des groupes de Lie" , 2–3 , Hermann (1952–1955)
[2]	A. Borel, J. Tits, "Groupes réductifs" Publ. Math. IHES , 27 (1965) pp. 55–250
[3]	A. Borel, "Linear algebraic groups" , Benjamin (1969)
[4]	J.E. Humphreys, "Linear algebraic groups" , Springer (1975)