Logarithm of a number

to a base

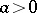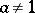The degree to which the number (the base of the logarithm) must be raised in order to obtain ; it is denoted by ; that is, means . To every positive number and given base , , there corresponds a unique real logarithm (logarithms of negative numbers are complex numbers). The main properties of the logarithm are:

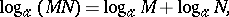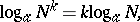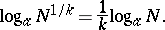These make it possible to reduce multiplication and division of numbers to the addition and subtraction of their logarithms, and the raising to powers and extraction of roots to the multiplication and division of the logarithm by the index of the power or root.

In accordance with the decimal system, the most commonly used are decimal logarithms 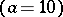, denoted by 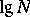. For rational numbers other than with 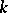an integer, the decimal logarithms are transcendental numbers (cf. Transcendental number), which can be approximately expressed by finite decimal fractions. The integer part of a decimal logarithm is called the characteristic, and the fractional part is called the mantissa. Since 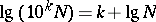, the decimal logarithms of numbers that differ by a multiple of 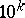have the same mantissa and differ only in the characteristics. This property is the basis for the construction of logarithm tables, which contain only the mantissa of the logarithms of integers.

The natural logarithms also have great significance; the base of these is the transcendental number ; they are denoted by . The transition from one base to another is carried out by the formula 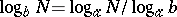; the factor 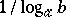is called the modulus of transition from the base to the base 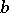. The formulas for the transition from natural logarithms to decimal logarithms and vice versa are:

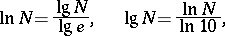See also Logarithmic function.

Comments

Western writers of post-calculus mathematics almost always use 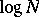instead of to denote the natural logarithm (base ) of 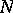. The number is given by 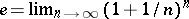and by 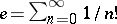(cf. (number)).

On the other hand, writers of calculus and pre-calculus books as well as manufacturers of calculators usually use for the decimal (common) logarithm and 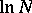for the natural logarithm. Cf. also Logarithmic function.