Chebyshev polynomials
of the first kind
Polynomials that are orthogonal on the interval  with the weight function
with the weight function
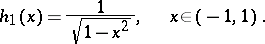 |
For the standardized Chebyshev polynomials one has the formula
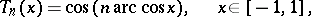 |
and the recurrence relation
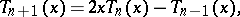 |
by which one can determine the sequence
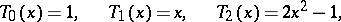 |
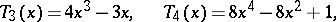 |
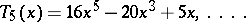 |
The orthonormalized Chebyshev polynomials are:
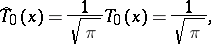 |
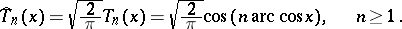 |
The leading coefficient of 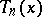 , for
, for 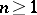 , is
, is 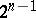 . Hence Chebyshev polynomials with leading coefficient 1 are defined by the formula
. Hence Chebyshev polynomials with leading coefficient 1 are defined by the formula
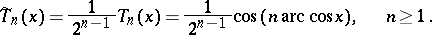 |
The zeros of 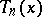 , given by
, given by
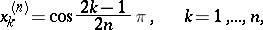 |
frequently occur as interpolation nodes in quadrature formulas. The polynomial 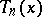 is a solution of the differential equation
is a solution of the differential equation
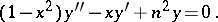 |
The polynomials 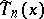 deviate as least as possible from zero on the interval
deviate as least as possible from zero on the interval  , that is, for any other polynomial
, that is, for any other polynomial 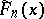 of degree
of degree  with leading coefficient 1 one has the following condition
with leading coefficient 1 one has the following condition
 |
On the other hand, for any polynomial 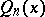 of degree
of degree  or less and satisfying
or less and satisfying
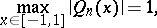 |
one has, for any 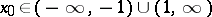 , the inequality
, the inequality
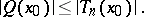 |
If a function 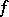 is continuous on the interval
is continuous on the interval 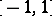 and if its modulus of continuity
and if its modulus of continuity  satisfies the Dini condition
satisfies the Dini condition
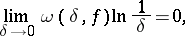 |
then this function can be expanded in a Fourier–Chebyshev series,
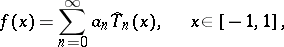 |
which converges uniformly on 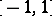 . The coefficients in this series are defined by the formula
. The coefficients in this series are defined by the formula
 |
If the function  is
is 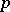 -times continuously differentiable on
-times continuously differentiable on 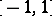 and if its
and if its 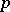 -th derivative
-th derivative 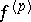 satisfies a Lipschitz condition of order
satisfies a Lipschitz condition of order 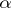 , i.e.
, i.e. 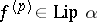 , then one has the inequality
, then one has the inequality
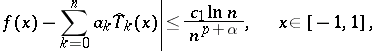 |
where the constant 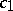 does not depend on
does not depend on  and
and  .
.
Chebyshev polynomials of the second kind are defined by
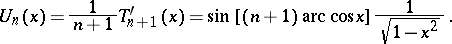 |
These polynomials are orthogonal on the interval 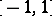 with weight function
with weight function
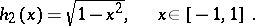 |
For any polynomial 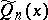 with leading coefficient 1 one has the inequality
with leading coefficient 1 one has the inequality
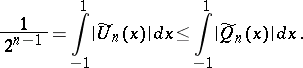 |
The Chebyshev polynomials were introduced in 1854 by P.L. Chebyshev (cf. [1]). Both systems of Chebyshev polynomials are special cases of ultraspherical polynomials and Jacobi polynomials.
References
| [1] | P.L. Chebyshev, , Collected works , 2 , Moscow-Leningrad (1947) pp. 23–51 (In Russian) |
| [2] | G. Szegö, "Orthogonal polynomials" , Amer. Math. Soc. (1975) |
Chebyshev polynomials. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Chebyshev_polynomials&oldid=16283