Weyl algebra
Let 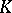 be a commutative field and
be a commutative field and  a positive integer. The ring of differential operators with coefficients in the polynomial ring
a positive integer. The ring of differential operators with coefficients in the polynomial ring 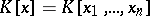 is denoted by
is denoted by  and called the Weyl algebra in
and called the Weyl algebra in  variables over
variables over 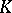 . Identifying
. Identifying  with the subring of zero-order differential operators, it follows that the ring
with the subring of zero-order differential operators, it follows that the ring 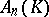 is generated by
is generated by 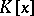 and the derivation operators
and the derivation operators  . The commutators
. The commutators 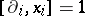 for every
for every 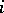 . So
. So 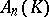 is a non-commutative ring. Every element has a unique representation
is a non-commutative ring. Every element has a unique representation
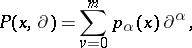 |
where  are monomials in the derivation operators. The largest integer
are monomials in the derivation operators. The largest integer 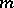 such that a polynomial coefficient
such that a polynomial coefficient 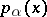 is non-zero with
is non-zero with  is the order of the differential operator
is the order of the differential operator 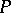 . The order yields a filtration (cf. Filtered module) and the associated graded ring (cf. Graded module)
. The order yields a filtration (cf. Filtered module) and the associated graded ring (cf. Graded module)
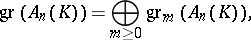 |
where  is the set of operators of order
is the set of operators of order 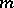 , divided by those of order
, divided by those of order 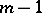 at most. It is well-known that the associated graded ring is isomorphic to the polynomial ring in
at most. It is well-known that the associated graded ring is isomorphic to the polynomial ring in 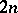 variables over
variables over  , where
, where 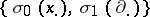 are the generators.
are the generators.
Ring-theoretic properties.
Here only the case when the field 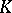 has characteristic zero is discussed. If
has characteristic zero is discussed. If  , the results below are no longer valid. For material when
, the results below are no longer valid. For material when 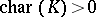 see [a30]. From now on
see [a30]. From now on 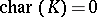 . Then
. Then  is a simple ring and since
is a simple ring and since  is Noetherian and commutative, it follows that
is Noetherian and commutative, it follows that  is both left and right Noetherian. By [a42], every left ideal of
is both left and right Noetherian. By [a42], every left ideal of 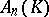 is generated by two elements. The global homological dimension of
is generated by two elements. The global homological dimension of 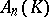 is equal to
is equal to  . This result was proved in [a37]. The case
. This result was proved in [a37]. The case 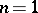 was settled before in [a35]. Another important result is the involutivity of characteristic ideals.
was settled before in [a35]. Another important result is the involutivity of characteristic ideals.
To explain this, one considers a finitely-generated left 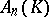 -module
-module  . A good filtration on
. A good filtration on 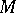 consists of an increasing sequence of
consists of an increasing sequence of 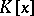 -submodules
-submodules 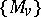 such that
such that 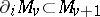 for all pairs
for all pairs 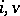 , and the associated graded module
, and the associated graded module  is finitely generated over
is finitely generated over 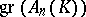 . A module can be equipped with different good filtrations. But there exists a unique graded ideal of
. A module can be equipped with different good filtrations. But there exists a unique graded ideal of 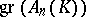 , given as the radical of the annihilating ideal of
, given as the radical of the annihilating ideal of 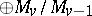 for any good filtration. It is denoted by
for any good filtration. It is denoted by 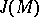 and called the characteristic ideal of
and called the characteristic ideal of 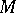 . On
. On 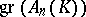 there exists a Poisson product such that
there exists a Poisson product such that 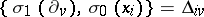 . The involutivity theorem asserts that
. The involutivity theorem asserts that
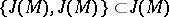 | (a1) |
for every finitely-generated left 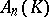 -module. In the special case when
-module. In the special case when 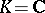 , the Nullstellen Satz (cf. Hilbert theorem) identifies
, the Nullstellen Satz (cf. Hilbert theorem) identifies 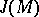 with an algebraic set in the symplectic cotangent space
with an algebraic set in the symplectic cotangent space 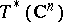 , denoted by
, denoted by  and called the characteristic variety of
and called the characteristic variety of 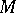 (cf. also Characteristic manifold). Then (a1) means that
(cf. also Characteristic manifold). Then (a1) means that  is involutive in the symplectic cotangent space.
is involutive in the symplectic cotangent space.
The involutivity implies that the dimension of 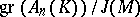 is at least
is at least  when
when 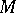 is a non-zero
is a non-zero 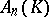 -module. This can be used to prove that
-module. This can be used to prove that  , using the fact that
, using the fact that 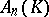 is a regular Auslander ring. See [a8] for a survey of this. The result (a1) was proved by micro-local analysis in [a40]. An algebraic proof was found later in [a14]. In [a26] characteristic ideals were used to show that if
is a regular Auslander ring. See [a8] for a survey of this. The result (a1) was proved by micro-local analysis in [a40]. An algebraic proof was found later in [a14]. In [a26] characteristic ideals were used to show that if 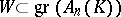 is a multiplicative set formed by homogeneous elements and
is a multiplicative set formed by homogeneous elements and 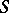 is the multiplicative set in
is the multiplicative set in  whose principal symbols belong to
whose principal symbols belong to 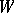 , then
, then 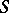 satisfies the two-sided Ore condition. So the universal
satisfies the two-sided Ore condition. So the universal 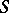 -inverting ring is the two-sided ring of Ore fractions
-inverting ring is the two-sided ring of Ore fractions 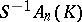 . In particular, this applies when
. In particular, this applies when 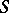 is the set of non-zero elements. The resulting division ring
is the set of non-zero elements. The resulting division ring 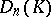 is related to division rings of certain enveloping algebras divided by primitive ideals.
is related to division rings of certain enveloping algebras divided by primitive ideals.
Holonomic modules.
The equality 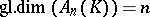 implies that its Krull dimension is at most
implies that its Krull dimension is at most  . The equality
. The equality 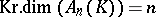 was actually proved before the discoveries above in [a15], using a trace formula which shows that every non-zero
was actually proved before the discoveries above in [a15], using a trace formula which shows that every non-zero 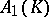 -module is an infinite-dimensional vector space over
-module is an infinite-dimensional vector space over 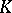 . Of special interest is the set of finitely-generated left or right
. Of special interest is the set of finitely-generated left or right 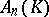 -modules
-modules 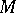 such that
such that 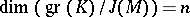 . Such modules are called holonomic and enjoy finiteness properties, e.g. every holonomic module is Artinian. The converse is not true, since [a43] gives examples of cyclic modules
. Such modules are called holonomic and enjoy finiteness properties, e.g. every holonomic module is Artinian. The converse is not true, since [a43] gives examples of cyclic modules 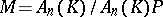 which are simple. Here
which are simple. Here  is any positive integer and
is any positive integer and  is a principal ideal, so
is a principal ideal, so 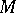 is non-holonomic when
is non-holonomic when  .
.
An important class of holonomic modules arise as follows: If 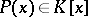 , then the subring of rational functions
, then the subring of rational functions  is a holonomic
is a holonomic  -module. This was proved by J. Bernstein in [a3], [a4], in which also a functional equation was found expressed by the equality:
-module. This was proved by J. Bernstein in [a3], [a4], in which also a functional equation was found expressed by the equality:
 | (a2) |
Here 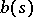 is a polynomial, chosen to have smallest possible degree and highest coefficient one. It is called the Bernstein–Sato polynomial of
is a polynomial, chosen to have smallest possible degree and highest coefficient one. It is called the Bernstein–Sato polynomial of 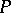 . The case when
. The case when  is of particular interest. It is proved in [a27] that the roots of
is of particular interest. It is proved in [a27] that the roots of  consist of strictly negative rational numbers. See also [a6] for this. The roots of
consist of strictly negative rational numbers. See also [a6] for this. The roots of 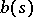 are related to the monodromy acting in
are related to the monodromy acting in  under the mapping
under the mapping 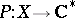 , where it is assumed that zero is the only critical value of
, where it is assumed that zero is the only critical value of 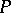 . In [a31] it is proved that the union
. In [a31] it is proved that the union 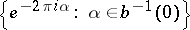 is equal to the union of eigenvalues of monodromy in every dimension in
is equal to the union of eigenvalues of monodromy in every dimension in  . Bernstein's functional equation gives meromorphic continuations of distributions. The meromorphic
. Bernstein's functional equation gives meromorphic continuations of distributions. The meromorphic 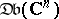 -valued function
-valued function 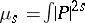 exists, with poles contained in the set
exists, with poles contained in the set  . The roots of the
. The roots of the 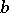 -function give an effective contribution to the set of poles. Namely, for any root
-function give an effective contribution to the set of poles. Namely, for any root 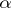 of
of 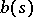 there exists some
there exists some 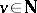 such that
such that 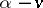 is a pole of
is a pole of 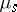 . This is proved in [a1].
. This is proved in [a1].
Fundamental solutions.
Let 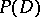 be a differential operator with constant coefficients. Using the Fourier transform, and replacing
be a differential operator with constant coefficients. Using the Fourier transform, and replacing 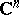 by
by 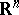 , the
, the 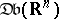 -valued function
-valued function 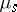 exists, acting on test-forms
exists, acting on test-forms  by
by
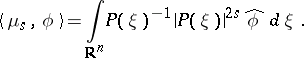 |
The constant term  at
at  gives a fundamental solution to
gives a fundamental solution to 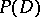 . Using results about regular holonomic modules it can be proved that the analytic wave front set of
. Using results about regular holonomic modules it can be proved that the analytic wave front set of 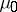 is equal to its
is equal to its 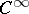 wave front set when the polynomial is homogeneous. For more results about Fourier transforms and
wave front set when the polynomial is homogeneous. For more results about Fourier transforms and 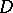 -modules with polynomial coefficients see [a32] (and also
-modules with polynomial coefficients see [a32] (and also 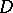 -module).
-module).
The Weyl algebra is a special case of rings of differential operators on non-singular algebraic varieties. See [a36] and [a20] for such constructions, which were made before the detailed study of Weyl algebras started.
But foremost 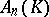 is fundamental in algebraic
is fundamental in algebraic  -module theory. The reason is that any quasi-projective manifold
-module theory. The reason is that any quasi-projective manifold 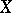 over
over 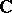 is covered in the Zariski topology by charts given by affine manifolds
is covered in the Zariski topology by charts given by affine manifolds 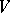 for which there exists an unramified covering mapping onto
for which there exists an unramified covering mapping onto 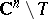 , where
, where 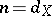 and
and 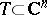 is an algebraic hypersurface. The ring of differential operators on the affine algebra
is an algebraic hypersurface. The ring of differential operators on the affine algebra  of regular functions on
of regular functions on 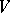 is equal to
is equal to 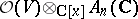 . For algebraic
. For algebraic 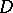 -module theory see [a9].
-module theory see [a9].
Fuchsian filtrations.
Above, the filtration on 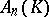 defined by the usual order of differential operators was considered. The Weyl algebra can be endowed with other filtrations, which no longer are positive. With
defined by the usual order of differential operators was considered. The Weyl algebra can be endowed with other filtrations, which no longer are positive. With  and coordinates
and coordinates 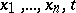 one takes the hyperplane
one takes the hyperplane  , and the filtration on
, and the filtration on 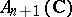 is such that
is such that 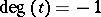 ,
, 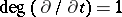 , while
, while 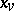 and
and 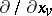 are of degree zero for every
are of degree zero for every  . The associated graded ring is
. The associated graded ring is 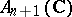 . The associated Rees ring of the Fuchsian filtered ring is Noetherian and its global homological dimension is
. The associated Rees ring of the Fuchsian filtered ring is Noetherian and its global homological dimension is 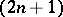 . This ring has interest in its own and appears in more extensive classes of rings, [a41]. The Fuchsian filtration is adapted to study nearby and vanishing cycles (cf. Vanishing cycle). If
. This ring has interest in its own and appears in more extensive classes of rings, [a41]. The Fuchsian filtration is adapted to study nearby and vanishing cycles (cf. Vanishing cycle). If 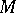 is a holonomic
is a holonomic  -module, then there exists a unique good filtration
-module, then there exists a unique good filtration 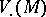 with respect to the Fuchsian filtration such that the minimal polynomial of the Euler mapping on
with respect to the Fuchsian filtration such that the minimal polynomial of the Euler mapping on 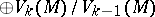 has roots in the lattice
has roots in the lattice  . One refers to this as the Kashiwara–Malgrange filtration. Every homogeneous quotient
. One refers to this as the Kashiwara–Malgrange filtration. Every homogeneous quotient  is a holonomic module over the
is a holonomic module over the  -dimensional Weyl algebra in the
-dimensional Weyl algebra in the  -variables. When
-variables. When 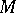 is regular holonomic and the Riemann–Hilbert correspondence is applied, one proves that the de Rham complex of
is regular holonomic and the Riemann–Hilbert correspondence is applied, one proves that the de Rham complex of 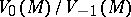 is the nearby cycle along
is the nearby cycle along 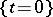 of the perverse sheaf complex defined by the de Rham complex of
of the perverse sheaf complex defined by the de Rham complex of 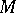 . For further details see [a16].
. For further details see [a16].
One should also mention the Bernstein filtration on the Weyl algebra, where both  and
and  have degree
have degree 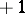 . For
. For  , [a44] contains a description of the graded ideals of
, [a44] contains a description of the graded ideals of 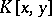 which are generated by principal symbols of elements in a left ideal of
which are generated by principal symbols of elements in a left ideal of 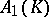 , using the Bernstein filtration to identify
, using the Bernstein filtration to identify 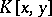 with
with 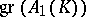 .
.
Weyl algebras with coefficients in a ring exist, i.e. for any ring  the ring
the ring  exists. New phenomena may occur when
exists. New phenomena may occur when 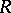 is non-commutative. The work [a19] shows that if
is non-commutative. The work [a19] shows that if 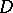 is the skew-field given by the quotient field
is the skew-field given by the quotient field 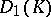 , then the ring
, then the ring 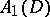 has global dimension
has global dimension  . For further results of Weyl algebras over division rings see [a18]. If
. For further results of Weyl algebras over division rings see [a18]. If 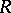 is a commutative Noetherian and regular
is a commutative Noetherian and regular 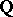 -algebra, one has
-algebra, one has
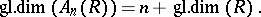 |
See [a6] and [a17] for this result and various extensions, where the global homological dimension is computed for other classes of rings of differential operators. If 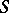 is a non-commutative
is a non-commutative 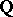 -algebra equipped with a Zariskian filtration such that
-algebra equipped with a Zariskian filtration such that 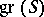 is a commutative regular Noetherian ring, one constructs
is a commutative regular Noetherian ring, one constructs 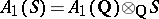 . It is an open problem if
. It is an open problem if 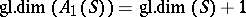 holds. The work [a13] contains results which predict the grade number of graded modules over
holds. The work [a13] contains results which predict the grade number of graded modules over 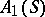 where the Fuchsian graded structure on
where the Fuchsian graded structure on 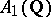 induces a graded ring structure on
induces a graded ring structure on 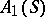 .
.
Invariant theory is another topic where the Weyl algebra appears. In [a29] it is proved that if 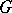 is a finite group of automorphisms on
is a finite group of automorphisms on 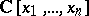 which does not contain any pseudo-reflection different from the identity, then the ring of differential operators on the
which does not contain any pseudo-reflection different from the identity, then the ring of differential operators on the 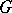 -invariant subring of
-invariant subring of 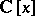 is equal to the
is equal to the 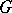 -invariant subring
-invariant subring  . Moreover, the ring
. Moreover, the ring 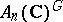 is an Auslander–Gorenstein ring whose injective dimension is
is an Auslander–Gorenstein ring whose injective dimension is  . This means that the bimodule given by the ring
. This means that the bimodule given by the ring 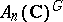 has an injective resolution of length
has an injective resolution of length  both as a left and a right module, and Auslander's condition holds:
both as a left and a right module, and Auslander's condition holds:
 |
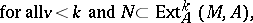 |
where 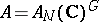 and
and 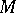 is any finitely-generated
is any finitely-generated 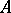 -module. This condition was originally verified for an extensive class of filtered rings, including the Weyl algebra in [a39]. See [a30] for more facts about rings of differential operators related to invariant theory.
-module. This condition was originally verified for an extensive class of filtered rings, including the Weyl algebra in [a39]. See [a30] for more facts about rings of differential operators related to invariant theory.
Noetherian operators.
The Weyl algebra is used in commutative algebra to describe primary ideals by equations with respect to its prime radical. Let  be a primary ideal and set
be a primary ideal and set 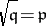 . Then there exists a finite set
. Then there exists a finite set 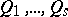 in
in 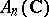 such that
such that 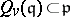 for every
for every  . Conversely, let
. Conversely, let 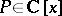 be such that
be such that 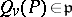 for every
for every  . Then
. Then 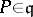 . So
. So  becomes a submodule of a direct sum of
becomes a submodule of a direct sum of  . This fact is used in the fundamental principle by Ehrenpreis to represent solutions to homogeneous systems of partial differential equations by absolutely convergent integrals over exponential solutions. See [a23], which also includes a construction of Noetherian operators.
. This fact is used in the fundamental principle by Ehrenpreis to represent solutions to homogeneous systems of partial differential equations by absolutely convergent integrals over exponential solutions. See [a23], which also includes a construction of Noetherian operators.
Enveloping algebras.
If 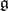 is a Heisenberg algebra, i.e. a nilpotent finite-dimensional Lie algebra with
is a Heisenberg algebra, i.e. a nilpotent finite-dimensional Lie algebra with 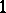 -dimensional centre
-dimensional centre  such that
such that 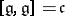 , then
, then 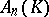 is a quotient ring of the enveloping algebra over
is a quotient ring of the enveloping algebra over  . Hence
. Hence 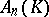 -modules yield representations of
-modules yield representations of 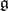 , which are infinite dimensional when
, which are infinite dimensional when 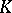 has characteristic zero. See [a11] for this. Quotients by primitive ideals of enveloping algebras of semi-simple Lie algebras lead to more involved results. The Weyl algebra
has characteristic zero. See [a11] for this. Quotients by primitive ideals of enveloping algebras of semi-simple Lie algebras lead to more involved results. The Weyl algebra 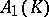 appears in the study of
appears in the study of 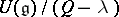 , where
, where  ,
, 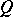 is the Casimir operator and
is the Casimir operator and  . This ring is a subring of
. This ring is a subring of  . See [a38], [a39]. Several problems concerned with semi-simple elements of [a11] were proposed in [a12]. For affirmative answers in some cases and related problems to Weyl algebras in many variables see [a24]. Finally one should mention the fundamental result in [a2], which is crucial for applications of algebraic
. See [a38], [a39]. Several problems concerned with semi-simple elements of [a11] were proposed in [a12]. For affirmative answers in some cases and related problems to Weyl algebras in many variables see [a24]. Finally one should mention the fundamental result in [a2], which is crucial for applications of algebraic  -module theory to representation theory of Lie algebras. See also [a25]. This gives special interest to the Weyl algebra and related rings, such as the ring of differential operators on the projective space
-module theory to representation theory of Lie algebras. See also [a25]. This gives special interest to the Weyl algebra and related rings, such as the ring of differential operators on the projective space 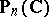 , which were determined in . See also [a21] for
, which were determined in . See also [a21] for 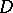 -module theory related to representations of Lie algebras.
-module theory related to representations of Lie algebras.
References
| [a1] | D. Barlet, "Monodromic et pôles de 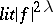 " Bull. Soc. Math. France , 114 (1986) pp. 247–269 " Bull. Soc. Math. France , 114 (1986) pp. 247–269 |
| [a2] | A.A. Beilinson, J. Bernstein, "Localisation des 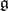 -modules" C.R. Acad. Sci. Paris , 292 (1981) pp. 15–18 -modules" C.R. Acad. Sci. Paris , 292 (1981) pp. 15–18 |
| [a3] | I.N. Bernstein, "Modules over a ring of differential operators. Study of the fundamental solutions to equations with constant coefficients" Funct. Anal. Appl. , 5 : 2 (1971) pp. 89–101 Funkts. Anal. i Prilozh. , 5 : 2 (1971) pp. 1–16 |
| [a4] | J. Bernstein, "The analytic continuation of generalized functions with respect to a parameter" Funct. Anal. Appl. , 6 : 4 (1972) pp. 273–285 Funkts. Anal. i Prilozh. , 6 : 4 (1972) pp. 3–25 |
| [a5] | F. Bien, "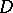 -modules and spherical representations of symmetric spaces" , Princeton Univ. Press (1986) -modules and spherical representations of symmetric spaces" , Princeton Univ. Press (1986) |
| [a6] | J.-E. Björk, "Rings of differential operators" , North-Holland (1979) |
| [a7] | J.-E. Björk, "The global homological dimension of some algebras of differential operators" Invent. Math. , 17 (1972) pp. 67–78 |
| [a8] | J.-E. Björk, "Non-commutative Noetherian rings and their use in homological algebra" J. Pure Appl. Algebra , 38 (1985) pp. 111–119 |
| [a9] | A. Borel, et al., "Algebraic 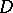 -modules" , Acad. Press (1987) -modules" , Acad. Press (1987) |
| [a10a] | W. Borho, J.-L Brylinski, "Differential operators on homogeneous spaces I" Invent. Math. , 69 (1982) pp. 437–476 |
| [a10b] | W. Borho, J.-L Brylinski, "Differential operators on homogeneous spaces II" Invent. Math. , 80 (1985) pp. 1–68 |
| [a11] | J. Dixmier, "Algèbres enveloppantes" , Gauthier-Villars (1974) |
| [a12] | J. Dixmier, "Sur les algèbres de Weyl II" Bull. Sci. Math. , 94 (1970) pp. 289–301 |
| [a13] | E.K. Ekström, "Homological properties of some Weyl algebra extensions" Compositio Math. , 75 (1989) pp. 231–246 |
| [a14] | O. Gabber, "The integrability of the characteristic variety" Amer. J. Math. , 103 (1981) pp. 445–468 |
| [a15] | P. Gabriel, R. Rentschler, "Sur la dimension des anneaux et ensembles ordonnes" C.R. Acad. Sci. Paris , 265 (1967) pp. A712-A715 |
| [a16] | V. Ginsburg, "Characteristic varieties and vanishing cycles" Inv. Math. , 84 (1986) pp. 327–403 |
| [a17] | K.R. Goodearl, R.B. Warfield Jr., "Krull dimension of differential operator rings" Proc. London Math. Soc. , 45 (1982) pp. 49–70 |
| [a18] | K.R. Goodearl, T.J. Hodges, T.H. Lenagan, "Krull and global dimensions of Weyl algebras over division rings" J. Algebra , 91 (1984) pp. 334–359 |
| [a19] | R. Hart, "A note on tensor products of algebras" J. Algebra , 21 (1972) pp. 422–427 |
| [a20] | G. Hochschild, B. Kostant, B. Rosenber, "Differential forms on regular affine algebras" Trans. Amer. Math. Soc. , 102 (1962) pp. 383–408 |
| [a21] | R. Hotta, M. Kashiwara, "The invariant system on a semi-simple Lie algebra" Inv. Math. , 75 (1984) pp. 327–358 |
| [a22] | L.V. Hörmander, "The analysis of linear partial differential operators" , 1 , Springer (1983) |
| [a23] | L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1990) |
| [a24] | A. Joseph, "The Weyl algebra - semisimple and nilpotent elements" Amer. J. Math. , 97 (1975) pp. 597–615 |
| [a25] | A. Joseph, "Primitive ideals in enveloping algebras" , Proc. Internat. Congress Mathematicians (Warszawa, 1983) , 1 , PWN & North-Holland (1984) pp. 403–414 |
| [a26] | M. Kashiwara, "A study of over-determined systems" , Kyoto University (1970) (Thesis) |
| [a27] | M. Kashiwara, "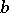 -functions and holonomic systems" Inv. Math. , 38 (1975) pp. 121–135 -functions and holonomic systems" Inv. Math. , 38 (1975) pp. 121–135 |
| [a28] | M. Kashiwara, "Regular holonomic 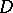 -modules and distributions on complex manifolds" T. Suwa (ed.) P. Wagreich (ed.) , Complex analytic singularities , Adv. Studies in Math. , 8 , Kinokuniya & North-Holland (1987) pp. 199–206 -modules and distributions on complex manifolds" T. Suwa (ed.) P. Wagreich (ed.) , Complex analytic singularities , Adv. Studies in Math. , 8 , Kinokuniya & North-Holland (1987) pp. 199–206 |
| [a29] | T. Levasseur, "Anneaux d'operateurs differentiels" M.P. Malliavin (ed.) , Sem. P. Dubreil et M.P. Malliavin , Lect. notes in math. , 867 , Springer (157–173) |
| [a30] | T. Levasseur, J.T. Stafford, "Rings of differential operators on classical rings of invariants" Memoirs Amer. Math. Soc. , 41 (1989) |
| [a31] | B. Malgrange, "Polynome de Bernstein–Sato et cohomologie evanescente" Asterisque , 101–102 (1983) pp. 243–267 |
| [a32] | B. Malgrange, "Equations différentiels à coefficients polynomiaux" , Birkhäuser (1991) |
| [a33] | J.C. McConnel, "Noncommutative Noetherian rings" , Wiley (1987) |
| [a34] | P. Revoy, "Algèbres de Weyl en caracteristique  " C.R. Acad. Sci. Paris , 276 (1973) pp. 225–228 " C.R. Acad. Sci. Paris , 276 (1973) pp. 225–228 |
| [a35] | G.S. Rinehart, "Note on the global dimension of a certain ring" Proc. Amer. Math. Soc. , 13 (1963) pp. 195–222 |
| [a36] | G.S. Rinehart, "Differential forms on general commutative algebras" Trans. Amer. Math. Soc. , 103 (1963) pp. 195–222 |
| [a37] | J.-E. Roos, "Determination de la dimension homologique globale des algèbres de Weyl" C.R. Acad. Sci. Paris , 274 (1972) pp. A23-A26 |
| [a38] | J.-E. Roos, "Properties homologiques des quotients primitifs des algèbres enveloppantes des algèbres de Lie semi-simples" C.R. Acad. Sci. Paris , 276 (1973) pp. 351–354 |
| [a39] | J.-E. Roos, "Complements" C.R. Acad. Sci. Paris , 276 (1973) pp. 447–450 |
| [a40] | M. Kashiwara, "Microfunctions and pseudo-differential equations" H. Komatsu (ed.) , Hyperfunctions and pseudodifferential equations. Proc. Conf. Katata, 1971 , Lect. notes in math. , 287 , Springer (1973) pp. 265–529 |
| [a41] | S.P. Smith, "Differential operators in commutative algebras" , Lect. notes in math. , 1197 , Springer (1986) pp. 165–177 |
| [a42] | J.L. Stafford, "Non-holonomic modules over Weyl algebras and enveloping algebras" Inv. Math. , 79 (1985) pp. 619–638 |
| [a43] | J.L. Stafford, "Module structure over Weyl algebras" Leeds Univ. preprint (1977) |
| [a44] | P. Strömbeck, "On left ideals in 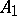 and their associated graded ideals" J. Algebra , 55 (1978) pp. 116–144 and their associated graded ideals" J. Algebra , 55 (1978) pp. 116–144 |
| [a45] | J.-E. Björk, "Analytic  -modules" , Kluwer (1993) -modules" , Kluwer (1993) |
Weyl algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Weyl_algebra&oldid=16166