Pseudo-group structure
on a manifold 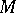
A maximal atlas 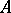 of smooth local diffeomorphisms (cf. Diffeomorphism) from
of smooth local diffeomorphisms (cf. Diffeomorphism) from 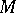 onto a fixed manifold
onto a fixed manifold 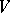 , all transition functions between them belonging to a given pseudo-group
, all transition functions between them belonging to a given pseudo-group 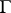 of local transformations of
of local transformations of 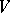 . The pseudo-group
. The pseudo-group 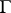 is called the defining pseudo-group, and
is called the defining pseudo-group, and 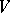 is called the model space. The pseudo-group structure with defining group
is called the model space. The pseudo-group structure with defining group 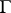 is also called a
is also called a 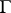 -structure. More precisely, a set
-structure. More precisely, a set 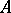 of
of 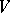 -valued charts of a manifold
-valued charts of a manifold  (i.e. of diffeomorphisms
(i.e. of diffeomorphisms 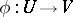 of open subsets
of open subsets 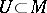 onto open subsets
onto open subsets  ) is called a pseudo-group structure if a) any point
) is called a pseudo-group structure if a) any point 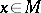 belongs to the domain of definition of a chart
belongs to the domain of definition of a chart 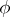 of
of  ; b) for any charts
; b) for any charts 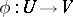 and
and 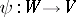 from
from 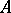 the transition function
the transition function  is a local transformation from the given pseudo-group
is a local transformation from the given pseudo-group  ; and c)
; and c)  is a maximal set of charts satisfying condition b).
is a maximal set of charts satisfying condition b).
Examples of pseudo-group structures.
1) A pseudo-group 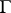 of transformations of a manifold
of transformations of a manifold 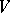 gives a pseudo-group structure
gives a pseudo-group structure 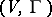 on
on 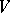 whose charts are the local transformations of
whose charts are the local transformations of  . It is called the standard flat
. It is called the standard flat 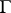 -structure.
-structure.
2) Let 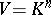 be an
be an  -dimensional vector space over
-dimensional vector space over 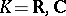 or a left module over the skew-field of quaternions
or a left module over the skew-field of quaternions  , and let
, and let 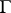 be the pseudo-group of local transformations of
be the pseudo-group of local transformations of 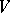 whose principal linear parts belong to the group
whose principal linear parts belong to the group 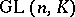 . The corresponding
. The corresponding 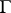 -structure on a manifold
-structure on a manifold 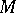 is the structure of a smooth manifold if
is the structure of a smooth manifold if 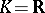 , of a complex-analytic manifold if
, of a complex-analytic manifold if 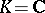 and of a special quaternionic manifold if
and of a special quaternionic manifold if 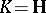 .
.
3) Let 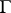 be the pseudo-group of local transformations of a vector space
be the pseudo-group of local transformations of a vector space 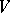 preserving a given tensor
preserving a given tensor 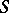 . Specifying a
. Specifying a 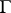 -structure is equivalent to specifying an integrable (global) tensor field of type
-structure is equivalent to specifying an integrable (global) tensor field of type 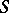 on a manifold
on a manifold 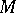 . E.g., if
. E.g., if 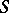 is a non-degenerate skew-symmetric
is a non-degenerate skew-symmetric  -form, then the
-form, then the 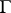 -structure is a symplectic structure.
-structure is a symplectic structure.
4) Let 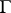 be the pseudo-group of local transformations of
be the pseudo-group of local transformations of 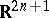 that preserve, up to a functional multiplier, the differential
that preserve, up to a functional multiplier, the differential  -form
-form
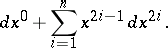 |
Then the  -structure is a contact structure.
-structure is a contact structure.
5) Let 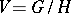 be a homogeneous space of a Lie group
be a homogeneous space of a Lie group 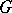 , and let
, and let  be the pseudo-group of local transformations of
be the pseudo-group of local transformations of 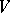 that can be lifted to transformations of
that can be lifted to transformations of 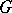 . Then the
. Then the  -structure is called the pseudo-group structure determined by the homogeneous space
-structure is called the pseudo-group structure determined by the homogeneous space 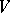 . Examples of such structures are the structure of a space of constant curvature (in particular, that of a locally Euclidean space), and conformally and projectively flat structures.
. Examples of such structures are the structure of a space of constant curvature (in particular, that of a locally Euclidean space), and conformally and projectively flat structures.
Let  be a transitive Lie pseudo-group of transformations of
be a transitive Lie pseudo-group of transformations of 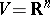 of order
of order 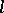 , see Pseudo-group. The
, see Pseudo-group. The 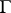 -structure
-structure 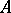 on a manifold
on a manifold  determines a principal subbundle
determines a principal subbundle 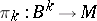 of the co-frame bundle of arbitrary order
of the co-frame bundle of arbitrary order 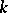 on
on 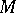 , consisting of the
, consisting of the 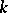 -jets of charts of
-jets of charts of 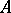 :
:
 |
The structure group of 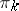 is the
is the 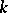 -th order isotropy group
-th order isotropy group  of
of 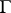 , which acts on
, which acts on 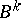 by the formula
by the formula
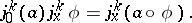 |
The bundle 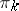 is called the
is called the 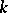 -th structure bundle, or
-th structure bundle, or 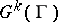 -structure, determined by the pseudo-group structure
-structure, determined by the pseudo-group structure 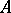 . The bundle
. The bundle 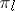 , with
, with 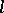 the order of
the order of 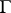 , in turn, uniquely determines the pseudo-group structure
, in turn, uniquely determines the pseudo-group structure 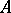 as the set of charts
as the set of charts 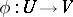 for which
for which
 |
The geometry of 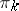 is characterized by the presence of a canonical
is characterized by the presence of a canonical  -equivariant
-equivariant 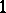 -form
-form 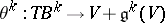 that is horizontal relative to the projection
that is horizontal relative to the projection 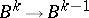 . Here
. Here 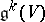 is the Lie algebra of the isotropy group
is the Lie algebra of the isotropy group 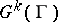 . The
. The 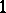 -form
-form 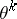 is given by
is given by
 |
where
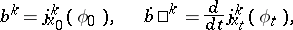 |
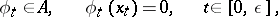 |
and satisfies a certain Maurer–Cartan structure equation (cf. also Maurer–Cartan form). The Lie algebra of infinitesimal automorphisms of the 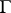 -structure can be characterized as the Lie algebra of projectable vector fields on
-structure can be characterized as the Lie algebra of projectable vector fields on 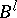 that preserve the canonical
that preserve the canonical 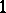 -form
-form 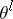 .
.
The basic problem in the theory of pseudo-group structures is the description of pseudo-group structures on manifolds with a defining pseudo-group 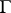 , up to equivalence. Two pseudo-group structures on a manifold are called equivalent if one of them can be reduced to the other by a diffeomorphism of the manifold.
, up to equivalence. Two pseudo-group structures on a manifold are called equivalent if one of them can be reduced to the other by a diffeomorphism of the manifold.
Let 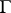 be a globalizing transitive pseudo-group of transformations of a simply-connected manifold
be a globalizing transitive pseudo-group of transformations of a simply-connected manifold 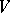 . Any simply-connected manifold with a
. Any simply-connected manifold with a 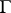 -structure
-structure  admits a mapping
admits a mapping 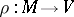 , called a Cartan development, that locally is an isomorphism of
, called a Cartan development, that locally is an isomorphism of  -structures. If
-structures. If 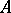 has some completeness property, then
has some completeness property, then 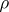 is an isomorphism of
is an isomorphism of 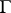 -structures and all
-structures and all  -structures of the type considered are forms of the standard
-structures of the type considered are forms of the standard 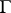 -structure
-structure 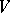 , i.e. are obtained from
, i.e. are obtained from  by factorization by a freely-acting discrete automorphism group
by factorization by a freely-acting discrete automorphism group  . This is the case, e.g. for (pseudo-)Riemannian structures of constant curvature and for conformally-flat structures on compact manifolds
. This is the case, e.g. for (pseudo-)Riemannian structures of constant curvature and for conformally-flat structures on compact manifolds 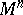 ,
,  .
.
The theory of deformations, originally developed for complex structures, occupies an important place in the theory of pseudo-group structures. In it one studies problems of the description of non-trivial deformations of a 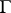 -structure
-structure 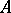 , i.e. a family
, i.e. a family  of
of  -structures containing the given
-structures containing the given 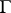 -structure and smoothly depending on a parameter
-structure and smoothly depending on a parameter 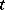 , modulo trivial deformations. The space of formal infinitesimal non-trivial deformations of a given
, modulo trivial deformations. The space of formal infinitesimal non-trivial deformations of a given 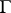 -structure is described by the one-dimensional cohomology space
-structure is described by the one-dimensional cohomology space  of
of  with coefficients in the sheaf
with coefficients in the sheaf  of germs of infinitesimal automorphisms of
of germs of infinitesimal automorphisms of 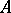 . The
. The 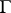 -structure is rigid if this space is trivial. If the two-dimensional cohomology space is trivial,
-structure is rigid if this space is trivial. If the two-dimensional cohomology space is trivial, 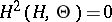 , one can prove, under certain assumptions, that there exist non-trivial deformations of the
, one can prove, under certain assumptions, that there exist non-trivial deformations of the  -structure, corresponding to given infinitesimal deformations from
-structure, corresponding to given infinitesimal deformations from  .
.
References
| [1] | E. Cartan, "La géométrie des éspaces Riemanniennes" , Mém. Sci. Math. , 9 , Gauthier-Villars (1925) |
| [2] | V. Guillemin, S. Sternberg, "Deformation theory of pseudogroup structures" , Mem. Amer. Math. Soc. , 64 , Amer. Math. Soc. (1966) |
| [3] | A.S. Pollack, "The integrability of pseudogroup structures" J. Diff. Geom. , 9 : 3 (1974) pp. 355–390 |
| [4a] | P.A. Griffiths, "Deformations of 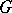 -structures. Part A: General theory of deformations" Math. Ann. , 155 : 4 (1964) pp. 292–315 -structures. Part A: General theory of deformations" Math. Ann. , 155 : 4 (1964) pp. 292–315 |
| [4b] | P.A. Griffiths, "Deformations of 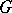 -structures. Part B: Deformations of geometric -structures. Part B: Deformations of geometric 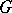 -structures" Math. Ann. , 158 : 5 (1965) pp. 326–351 -structures" Math. Ann. , 158 : 5 (1965) pp. 326–351 |
| [5] | J.F. Pommaret, "Théorie des déformations des structures" Ann. Inst. H. Poincaré Nouvelle Sér. , 18 (1973) pp. 285–352 (English abstract) |
| [6] | L. Berard Bergery, J.-P. Bourgignon, J. Lafontaine, "Déformations localement triviales des variétés Riemanniennes" , Differential geometry , Proc. Symp. Pure Math. , 27 , Amer. Math. Soc. (1975) pp. 3–32 |
| [7a] | D.C. Spencer, "Deformation of structures on manifolds defined by transitive, continuous pseudogroups I. Infinitesimal deformations of structure" Ann. of Math. , 76 : 2 (1962) pp. 306–398 |
| [7b] | D.C. Spencer, "Deformation of structures on manifolds defined by transitive, continuous pseudogroups II. Deformations of structure" Ann. of Math. , 76 : 3 (1962) pp. 399–445 |
Comments
For the topic of classifying spaces for  -structures cf. [a2].
-structures cf. [a2].
References
| [a1] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1 , Interscience (1963) pp. Chapt. 1 |
| [a2] | A. Haefliger, "Homotopy and integrability" J.N. Mordeson (ed.) et al. (ed.) , Structure of arbitrary purely inseparable extension fields , Lect. notes in math. , 173 , Springer (1971) pp. 133–163 |
| [a3] | J.F. Pommaret, "Systems of partial differential equations and Lie pseudogroups" , Gordon & Breach (1978) |
| [a4] | M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) |
Pseudo-group structure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pseudo-group_structure&oldid=16114