Integrable system

A differential system of dimension (cf. Involutive distribution) on an -dimensional differentiable manifold that has, in a neighbourhood of each point , an -parameter family of -dimensional integral manifolds (cf. Integral manifold). One often speaks of a totally-integrable system in this case; more precisely it is defined as follows. 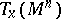Suppose that at each point a subspace of dimension in the tangent space has been distinguished, such that a differential system, or distribution, 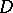of class 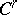, , 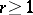of dimension 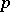is given on . The system is called totally integrable if for each point there is a coordinate system , , , such that for any constants 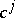, , the manifold is an integral submanifold, i.e. its tangent space at an arbitrary point coincides with . For analytic conditions that are necessary and sufficient for this, see Involutive distribution.

Comments

Cf. also Pfaffian equation. The phrase integrable system is also used to refer to a completely-integrable Hamiltonian system or equation, i.e. a Hamiltonian equation (system) on a 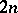-dimensional phase space which has (including the Hamiltonian itself) integrals in involution, cf. Hamiltonian system.