Inner automorphism

of a group

An automorphism such that

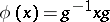for a certain fixed element . The set of all inner automorphisms of forms a normal subgroup in the group of all automorphisms of ; this subgroup is isomorphic to , where is the centre of (cf. Centre of a group). Automorphisms that are not inner are called outer automorphisms.

Other relevant concepts include those of an inner automorphism of a monoid (a semi-group with a unit element) and an inner automorphism of a ring (associative with a unit element), which are introduced in a similar way using invertible elements.

Comments

Let 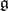be a Lie algebra and an element of for which 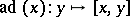is nilpotent. Then

defines an automorphism of 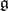. Such an automorphism is called an inner automorphism of 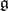. More generally, the elements in the group 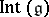generated by them are called inner automorphisms. It is a normal subgroup of .

If is a real or complex Lie group with semi-simple Lie algebra, then the inner automorphisms constitute precisely the identity component of the group 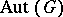of automorphisms of .

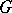References

[a1]	M. Hall jr., "The theory of groups" , Macmillan (1959)
[a2]	J.E. Humphreys, "Introduction to Lie algebras and representation theory" , Springer (1972) pp. §5.4
[a3]	J.-P. Serre, "Algèbres de Lie semi-simples complexes" , Benjamin (1966)