Discriminant curve
of an ordinary first-order differential equation 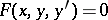
The set of points 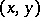 of the plane whose coordinates satisfy the equation
of the plane whose coordinates satisfy the equation 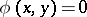 obtained by the elimination of
obtained by the elimination of  from the relations
from the relations  and
and 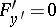 or by elimination of
or by elimination of 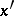 from the relations
from the relations 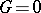 and
and 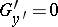 , where
, where 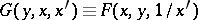 (on the assumption that
(on the assumption that 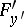 in fact exists). If the discriminant curve for the equation
in fact exists). If the discriminant curve for the equation 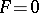 is a non-empty set and does not degenerate into individual points, the curve (or each one of its branches) may:
is a non-empty set and does not degenerate into individual points, the curve (or each one of its branches) may:
1) be a solution of the equation 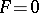 at each point of which uniqueness is violated; the discriminant curve will then form the envelope of a family of integral curves (e.g.
at each point of which uniqueness is violated; the discriminant curve will then form the envelope of a family of integral curves (e.g. 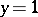 and
and 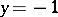 for the equation
for the equation 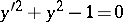 (Fig. a); or
(Fig. a); or  for the equation
for the equation  (Fig. b));
(Fig. b));
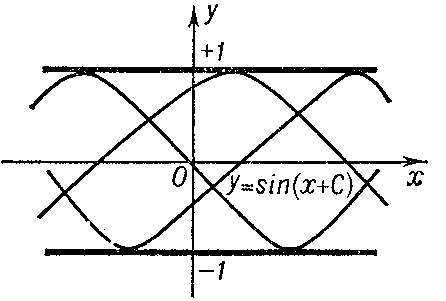
Figure: d033220a
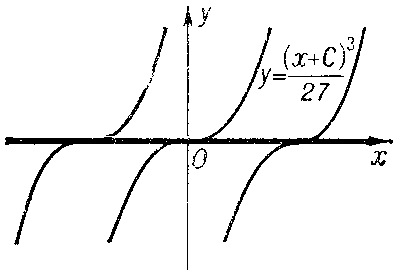
Figure: d033220b
2) be a solution of the equation 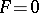 at each point of which there is uniqueness (e.g.
at each point of which there is uniqueness (e.g.  for the equation
for the equation 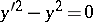 (Fig. c));
(Fig. c));
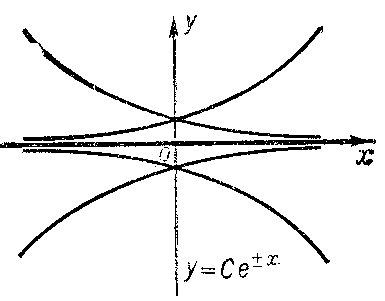
Figure: d033220c
3) not be a solution of the equation  . In this case the discriminant curve is either the set of cuspidal points of the integral curves (e.g.
. In this case the discriminant curve is either the set of cuspidal points of the integral curves (e.g. 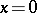 for the equation
for the equation 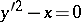 (Fig. d)) or the set of osculation points of different integral curves (e.g.
(Fig. d)) or the set of osculation points of different integral curves (e.g. 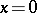 for the equation
for the equation 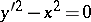 (Fig. e)).
(Fig. e)).
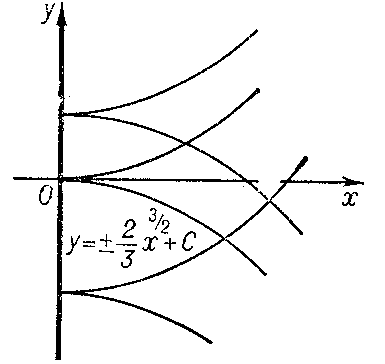
Figure: d033220d

Figure: d033220e
The equation 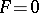 , when
, when  is a polynomial in
is a polynomial in 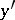 , is also studied in the complex domain [2].
, is also studied in the complex domain [2].
References
| [1] | G. Sansone, "Equazioni differenziali nel campo reale" , 2 , Zanichelli (1949) |
| [2] | V.V. Golubev, "Vorlesungen über Differentialgleichungen im Komplexen" , Deutsch. Verlag Wissenschaft. (1958) (Translated from Russian) |
Comments
References
| [a1] | E.L. Ince, "Ordinary differential equations" , Dover, reprint (1956) |
Discriminant curve. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Discriminant_curve&oldid=15781