Path-connected space

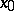A topological space in which any two points can be joined by a continuous image of a simple arc; that is, a space for any two points and 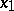of which there is a continuous mapping 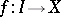of the unit interval 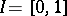such that 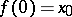and 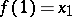. A path-connected Hausdorff space is a Hausdorff space in which any two points can be joined by a simple arc, or (what amounts to the same thing) a Hausdorff space into which any mapping of a zero-dimensional sphere is homotopic to a constant mapping. Every path-connected space is connected (cf. Connected space). A continuous image of a path-connected space is path-connected.

Path-connected spaces play an important role in homotopic topology. If a space 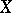is path-connected and , then the homotopy groups 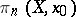and are isomorphic, and this isomorphism is uniquely determined up to the action of the group 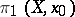. If 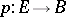is a fibration with path-connected base 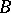, then any two fibres have the same homotopy type. If is a weak fibration (a Serre fibration) over a path-connected base 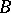, then any two fibres have the same weak homotopy type.

The multi-dimensional generalization of path connectedness is -connectedness (connectedness in dimension 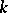). A space 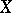is said to be connected in dimension if any mapping of an -dimensional sphere into 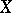, where 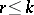, is homotopic to a constant mapping.

References

[1]	E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966)

Comments

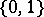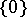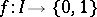A connected space is not necessarily path-connected. It is not true that in an arbitrary path-connected space any two points can be joined by a simple arc: consider the two-point space in which is open and is not. The mapping defined by

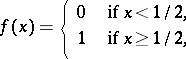is continuous and connects 0 and 1. A space in which any two points can be joined by a simple arc is called arcwise connected. Thus, path-connected Hausdorff spaces are arcwise connected.

References

[a1]	A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian)
[a2]	B. Gray, "Homotopy theory. An introduction to algebraic topology" , Acad. Press (1975) pp. 15ff, 130