Wandering point

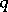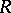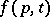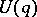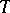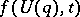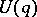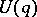A point in the phase space of a dynamical system with a neighbourhood for which there exists a moment in time such that has no common points with for all (all points of , from some moment on, leave the neighbourhood 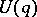). A point 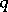without such a neighbourhood is said to be non-wandering. This property of a point — to be wandering or non-wandering — is two-sided: If has no common points with 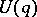, then 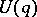has no common points with 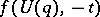. A wandering point may become non-wandering if the space 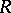is extended. For instance, if 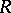is a circle with one rest point , all points of are wandering points. They become non-wandering if the points of some spiral without rest points, winding itself around this circle from the outside or from the inside, are added to .

Comments

A set 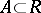is positively recursive with respect to a set 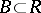if for all 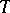there is a 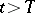such that 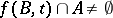. Negatively recursive is defined analogously. A point is then non-wandering if every neighbourhood of it is positively recursive with respect to itself (self-positively recursive). A point is positively Poisson stable (negatively Poisson stable) if every neighbourhood of it is positively recursive (negatively recursive) with respect to 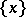. A point is Poisson stable if it is both positively and negatively Poisson stable. If 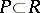is such that every 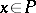is positively or negatively Poisson stable, then all points of 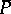are non-wandering. See also Wandering set.

References

[a1]	N.P. Bhatia, G.P. Szegö, "Stability theory of dynamical systems" , Springer (1970) pp. 30–36