Laplace-Stieltjes transform
From Encyclopedia of Mathematics
Let  be a function of bounded variation on
be a function of bounded variation on  , for all positive
, for all positive  . The integral
. The integral
 |
is known as a (formal) Laplace–Stieltjes integral.
If the integral converges for some complex number  , then it converges for all
, then it converges for all  with
with  , and the function
, and the function  is then the Laplace–Stieltjes transform of
is then the Laplace–Stieltjes transform of  . If
. If 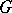 is of the form
is of the form 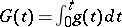 for a function
for a function 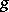 on
on 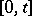 that is Lebesgue integrable for all
that is Lebesgue integrable for all 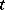 (see Lebesgue integral), then the Laplace–Stieltjes transform becomes the Laplace transform
(see Lebesgue integral), then the Laplace–Stieltjes transform becomes the Laplace transform 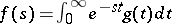 of
of 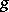 .
.
There is also a corresponding two-sided Laplace–Stieltjes transform (or bilateral Laplace–Stieltjes transform) for suitable functions 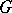 .
.
See Laplace transform for additional references.
References
| [a1] | D.V. Widder, "An introduction to transform theory" , Acad. Press (1971) |
How to Cite This Entry:
Laplace-Stieltjes transform. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Laplace-Stieltjes_transform&oldid=15100
Laplace-Stieltjes transform. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Laplace-Stieltjes_transform&oldid=15100
This article was adapted from an original article by M. Hazewinkel (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article