Free product

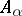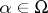in a class of universal algebras , , from

An algebra 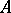from that contains all the as subalgebras and is such that any family of homomorphisms of the 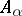into any other algebra from 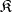can be uniquely extended to a homomorphism of into . A free product automatically exists if 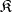is a variety of universal algebras. Every free algebra is the free product of free algebras generated by a singleton. The free product in the class of Abelian groups coincides with the direct sum. In certain cases there is a description of the subalgebras of a free product, for example, in groups (see Free product of groups), in non-associative algebras, and in Lie algebras.

A free product in categories of universal algebras coincides with the coproduct in these categories.

Comments

Free products do not always exists in a variety of algebras: for example, the ring of integers modulo and the ring of integers modulo have no free product in the variety of rings with 1. However, coproducts (which differ from free products in not requiring the canonical homomorphisms 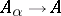to be injective) always exist in a variety of algebras [a1].

References

[a1]	F.E.J. Linton, "Coequalizors in categories of algebras" , Seminar on Triples and Categorical Homology Theory , Lect. notes in math. , 80 , Springer (1969) pp. 75–90