Arbitration scheme
A rule by which each arbitration game (cf. Cooperative game) is put into correspondence with a unique outcome of the game is called an arbitration solution. The first arbitration scheme was considered by J. Nash [1] for the case of a two-person game. Let 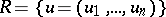 be the set of outcomes, let
be the set of outcomes, let 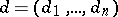 be the status quo point, i.e. the point corresponding to the situation in which no cooperative outcome is realized, let
be the status quo point, i.e. the point corresponding to the situation in which no cooperative outcome is realized, let  be the corresponding arbitration game and let
be the corresponding arbitration game and let  be an arbitration solution of it. An outcome
be an arbitration solution of it. An outcome 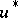 is called a Nash solution if
is called a Nash solution if
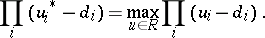 |
Only a Nash solution satisfies the following axioms: 1) if 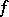 is a linear non-decreasing mapping then
is a linear non-decreasing mapping then 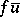 is an arbitration solution of the game
is an arbitration solution of the game 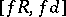 (invariance with respect to utility transformations); 2)
(invariance with respect to utility transformations); 2) 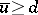 ,
, 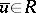 and there is no
and there is no 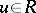 such that
such that 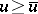 (Pareto optimality); 3) if
(Pareto optimality); 3) if 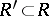 ,
,  ,
,  , then
, then  (independence of irrelevant alternatives); and 4) if
(independence of irrelevant alternatives); and 4) if 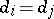 ,
, 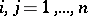 , and
, and 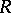 is symmetric, then
is symmetric, then 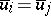 ,
, 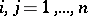 (symmetry).
(symmetry).
Another arbitration scheme for an  -person game with characteristic function
-person game with characteristic function  and player set
and player set  was given by L.S. Shapley [2]. The Shapley solution
was given by L.S. Shapley [2]. The Shapley solution 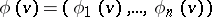 , where
, where
 |
 and
and  is the number of elements of the set
is the number of elements of the set 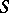 , also satisfies the axiom of symmetry, but, moreover,
, also satisfies the axiom of symmetry, but, moreover, 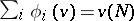 , and for any two games
, and for any two games  and
and  the equality
the equality  holds. Arbitration schemes with interpersonal utility comparisons have also been considered [3].
holds. Arbitration schemes with interpersonal utility comparisons have also been considered [3].
The arbitration schemes of Nash and Shapley were generalized by J.C. Harsanyi [4]. A Harsanyi solution satisfies, apart from the four axioms of Nash, the two axioms: 1) the solution depends monotonically on the initial demands of the players; and 2) if 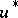 and
and  are two solutions, then
are two solutions, then  , defined by
, defined by
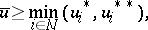 |
is also a solution if and only if  belongs to the boundary of the set
belongs to the boundary of the set 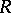 .
.
Under suitable conditions an arbitration scheme depends continuously on the parameters of the game.
References
| [1] | J. Nash, "The bargaining problem" Econometrica , 18 : 2 (1950) pp. 155–162 |
| [2] | L.S. Shapley, "A value for  -person games" H.W. Kuhn (ed.) A.W. Tucker (ed.) M. Dresher (ed.) , Contributions to the theory of games , 2 , Princeton Univ. Press (1953) pp. 307–317 -person games" H.W. Kuhn (ed.) A.W. Tucker (ed.) M. Dresher (ed.) , Contributions to the theory of games , 2 , Princeton Univ. Press (1953) pp. 307–317 |
| [3] | H. Raiffa, "Arbitration schemes for generalized two-person games" H.W. Kuhn (ed.) A.W. Tucker (ed.) M. Dresher (ed.) , Contributions to the theory of games , 2 , Princeton Univ. Press (1953) pp. 361–387 |
| [4] | J.C. Harsanyi, "A bargaining model for the cooperative  -person game" H.W. Kuhn (ed.) A.W. Tucker (ed.) M. Dresher (ed.) , Contributions to the theory of games , 4 , Princeton Univ. Press (1959) pp. 325–355 -person game" H.W. Kuhn (ed.) A.W. Tucker (ed.) M. Dresher (ed.) , Contributions to the theory of games , 4 , Princeton Univ. Press (1959) pp. 325–355 |
Comments
Arbitration schemes are also called bargaining schemes and a Nash solution is also called a bargaining solution. The Shapley solution vector 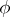 is also called the Shapley value. For other, more recent, bargaining schemes, such as the Kalai–Smorodinsky solution and Szidarovsky's generalization of the concept of a Nash solution, the reader is referred to [a1], [a2], respectively [a6]. For further developments concerning Harsanyi solutions, cf. [a3]. Some authors distinguish between bargaining schemes and arbitration schemes. Then the Nash scheme is a bargaining scheme and the Shapley one an arbitration scheme, [a5].
is also called the Shapley value. For other, more recent, bargaining schemes, such as the Kalai–Smorodinsky solution and Szidarovsky's generalization of the concept of a Nash solution, the reader is referred to [a1], [a2], respectively [a6]. For further developments concerning Harsanyi solutions, cf. [a3]. Some authors distinguish between bargaining schemes and arbitration schemes. Then the Nash scheme is a bargaining scheme and the Shapley one an arbitration scheme, [a5].
References
| [a1] | E. Kalai, M. Smorodinsky, "Other solutions to Nash's bargaining problems" Econometrica , 43 (1975) pp. 513–518 |
| [a2] | A.E. Roth, "Axiomatic models of bargaining" , Lect. notes econom. and math. systems , 170 , Springer (1979) |
| [a3] | J.C. Harsanyi, "Papers in game theory" , Reidel (1982) |
| [a4] | R.J. Aumann, L.S. Shapley, "Values of non-atomic games" , Princeton Univ. Press (1974) |
| [a5] | A. Rapoport, " -person game theory: Concepts and applications" , Univ. Michigan Press (1970) pp. 168 -person game theory: Concepts and applications" , Univ. Michigan Press (1970) pp. 168 |
| [a6] | J. Szép, F. Forgó, "Introduction to the theory of games" , Reidel (1985) pp. 171; 199 |
| [a7] | N.N. Vorob'ev, "Game theory. Lectures for economists and system scientists" , Springer (1977) (Translated from Russian) |
Arbitration scheme. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Arbitration_scheme&oldid=14856