Pfaffian problem

The problem of describing the integral manifolds of maximal dimension for a Pfaffian system of Pfaffian equations

(*)

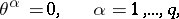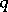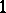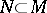given by a collection of differential -forms which are linearly independent at each point in a certain domain (or on a certain manifold). A submanifold is called an integral manifold of the system (*) if the restrictions of the forms 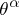to 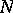are identically zero. The problem was posed by J. Pfaff (1814).

From a geometric point of view the system (*) determines an 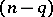-dimensional distribution (a Pfaffian structure) on 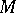, that is, a field

of -dimensional subspaces, and the Pfaffian problem consists of describing the submanifolds of maximum possible dimension tangent to this field. The importance of the Pfaffian problem lies in the fact that the integration of an arbitrary partial differential equation can be reduced to a Pfaffian problem. For example, the integration of a first-order equation

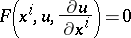reduces to the Pfaffian problem for the Pfaffian equation 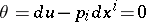on the submanifold (generally speaking with singularities) of the space 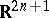defined by the equation

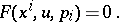A completely-integrable Pfaffian system (and also a single Pfaffian equation of constant class) can be locally reduced to a simple canonical form. In these cases the solution of the Pfaffian problem reduces to the solution of ordinary differential equations. In the general case (in the class of smooth functions) the Pfaffian problem has not yet been solved (1989). The Pfaffian problem was solved by E. Cartan in the analytic case in his theory of systems in involution (cf. Involutional system). The formulation of the basic theorem of Cartan is based on the concept of a regular integral element. A -dimensional subspace 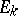of the tangent space 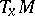is called a -dimensional integral element of the system (*) if

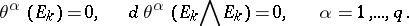The subspace 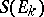of the cotangent space 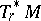generated by the -forms 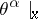, , 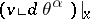where and is the operation of interior multiplication (contraction), is called the polar system of the integral element 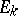. The integral element is regular if there exists a flag 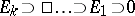for which

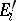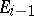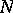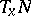where the maximum is taken over all -dimensional integral elements containing . Cartan's theorem asserts the following: Let be a -dimensional integral manifold of a Pfaffian system with analytic coefficients and let, for a certain , the tangent space be a regular integral element. Then for any integral element 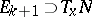of dimension 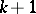there exists in a certain neighbourhood of the point an integral manifold 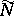, locally containing 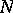, for which 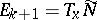. Cartan's theorem has been generalized to arbitrary differential systems given by ideals in the algebra of differential forms on a manifold (the Cartan–Kähler theorem).

References

[1]	E. Cartan, "Sur la théorie des systèmes en involution et ses applications à la relativité" Bull. Soc. Math. France , 59 (1931) pp. 88–118
[2]	E. Cartan, "Leçons sur les invariants intégraux" , Hermann (1922)
[3]	P.K. Rashevskii, "Geometric theory of partial differential equations" , Moscow-Leningrad (1947) (In Russian)
[4]	S. Sternberg, "Lectures on differential geometry" , Prentice-Hall (1964)
[5]	P.A. Griffiths, "Exterior differential systems and the calculus of variations" , Birkhäuser (1983)

Pfaffian problems and partial differential equations.

Let

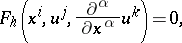(a1)

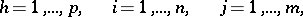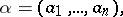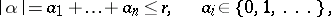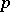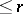be a system partial differential equations for functions in variables of order . Introduce the variables

Replacing the equations (a1) with the equations

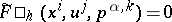(a2)

and adding to this the Pfaffian system

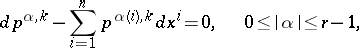(a3)

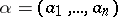where and if for 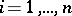, one finds a system (a2)–(a3) of equations which are equivalent to equations (a1) in a suitable sense. Thus, if (locally) (a2) defines a subvariety 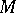in 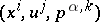-space, then a solution of the Pfaffian problem (a3) on 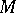defines a solution of (a1) in the sense that the projection onto 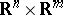(or 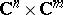as the case may be) gives the graph of a solution of (a1).

For instance, in the case of a single second-order equation

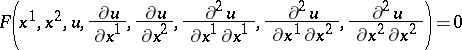one has for (a2) and (a3), respectively,

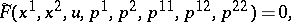(a2prm)

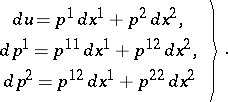(a3prm)

The main equations are (a2); the remaining equations (a3) express that the solutions of (a2) of interest are -jets (cf. Jet and Partial differential equations on a manifold) of functions 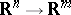. This leads to the idea of a system of partial differential equations on a manifold of order as being determined by a set of functions on the -th jet bundle; cf. Partial differential equations on a manifold for more details.

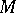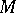In the setting of equations like (a2), (a3) the following generalization of Frobenius' theorem on complete integrability is of interest. Let be a set of differential forms on a manifold and a set of functions on . Let 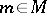be such that 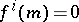, 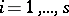. Suppose that

i) 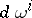and 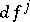are in the ideal of differential forms generated by ;

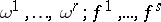ii) the 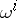are linearly independent at .

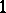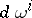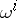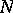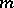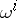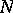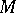(Recall that the linearly independent -forms form an involutive system if is in the ideal generated by the , cf. Involutive distribution.) Then there is a unique germ of a submanifold at of dimension , , such that the differential forms and functions restricted to are zero. Further if are functions on near 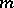such that 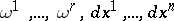are linearly independent at 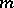, then the give a coordinate chart of 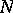near .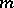

Cartan–Kähler theorem for differential systems defined by ideals.

Let , , be a Pfaffian system on 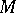and let be an integral manifold of this system. Then obviously the 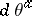and 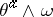, where 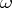is any differential form on 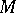, are also zero on 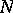. Thus all the elements of the differential ideal generated by 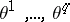in the differential algebra of exterior differential forms 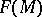(cf. Differential form; Differential ring) are zero on 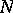. This leads to the idea of a differential system (of equations) on 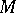as being defined by such an ideal. From now on let 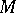be a real analytic manifold. Let 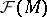be the associated sheaf to 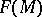, i.e. is the sheaf of germs of rings of differential forms on 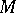. 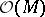Let be the sheaf of analytic functions on and let 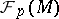be the -module of 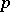-forms on 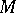. A differential system on 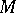is a graded differential subsheaf 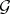of ideals of 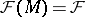, i.e. 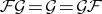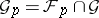(the ideal property), is generated by the (the graded property) and 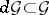(the differential property). A 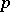-dimensional integral manifold for 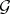is a submanifold 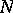of 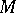on which 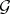is zero. 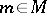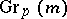For each let be the Grassmann manifold of 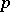-dimensional subspaces of the tangent space 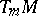. The union of the 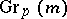for 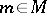has a natural structure of a real-analytic manifold and the projection 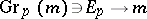then defines a locally trivial fibre bundle 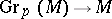. An element 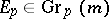is called a contact element at 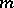. Such an element is an integral element o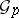f if 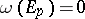for all 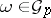; it is an integral element of a differential system 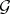if for all 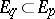, , 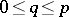is an integral element of 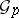. An integral element of dimension zero (i.e. a point of 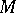) is an integral point (which is simply a solution of the equations 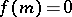for the functions 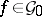). The polar element of an integral element for 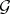is the element 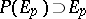consisting of all vectors such that the span of 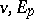is an integral element of 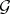. 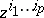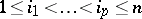Let , , be the Grassmann coordinates of 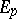(cf. Exterior algebra; these are only defined up to a common scalar multiple). Now associate to 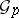the shea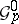f of -modules in 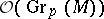consisting of all the functions for all 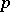-forms 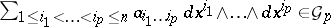. Let be the set of integral elements of 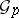(so that is a certain subset of the Grassmann bundle 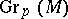). 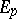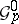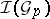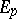The element is called a regular integral element if is a regular local equation for at and 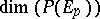is constant near on 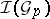. Recall that a subsheaf 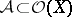, where 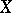is a manifold, is a regular local equation for (its set of zeros) 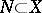at 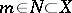if locally around 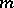there exist sections 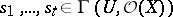such that the 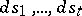are linearly independent on 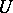and 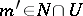if and only if .

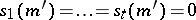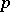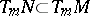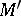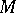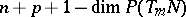The first Cartan–Kähler existence theorem is now as follows. Let be a -dimensional integral manifold of which defines a regular element at . Suppose that there is a submanifold of containing and of dimension such that 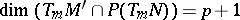. Then locally around 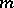there exists a unique integral manifold 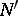of dimension 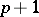contained in .

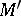If 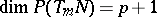, the only possible choice (locally) for 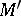is 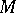itself, and there is a unique integral manifold of dimension 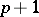extending 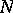. If 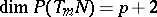there is "one arbitrary function worth" freedom in choosing 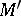and one re-encounters the phenomenon that the solution of a partial differential equation may depend on arbitrary functions (such as 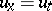with as solutions any function of the form 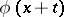). The second Cartan–Kähler existence theorem, which is obtained by repeated application of the first, details the dependence on initial conditions and arbitrary functions.

An immediate corollary of the first Cartan–Kähler existence theorem is as follows. Suppose one is given an integral element of dimension 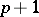of the differential system 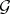at 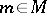which contains a regular integral element 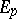. Then there exists (locally) an integral manifold of dimension such that .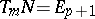

References

[a1]	P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) pp. Chapt. V, Appendix 3 (Translated from French)
[a2]	E. Cartan, "Les systèmes différentielles extérieurs et leur applications géométriques" , Hermann (1945)
[a3]	E. Cartan, "Sur l'intégration des systèmes d'équations aux différentielles totales" Ann. Sci. Ec. Norm. Sup. , 18 (1901) pp. 241–311
[a4]	E. Kähler, "Einführung in die Theorie der Systeme von Differentialgleichungen" , Teubner (1934)
[a5]	M. Kuranishi, "Lectures on exterior differential systems" , Tata Inst. (1962)
[a6]	J. Dieudonné, "Eléments d'analyse" , 4 , Gauthier-Villars (1977) pp. Chapt. XVIII, Sect. 13