Endomorphism ring
The associative ring  consisting of all morphisms of
consisting of all morphisms of  into itself, where
into itself, where  is an object in some additive category. The multiplication in
is an object in some additive category. The multiplication in  is composition of morphisms, and addition is the addition of morphisms defined by the axioms of the additive category. The identity morphism
is composition of morphisms, and addition is the addition of morphisms defined by the axioms of the additive category. The identity morphism  is the unit element of the ring
is the unit element of the ring  . An element
. An element  in
in  is invertible if and only if
is invertible if and only if  is an automorphism of the object
is an automorphism of the object  . If
. If 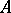 and
and 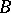 are objects of an additive category
are objects of an additive category 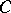 , then the group
, then the group  has the natural structure of a right module over
has the natural structure of a right module over  and of a left module over
and of a left module over  . Let
. Let  be a covariant (or contravariant) additive functor from an additive category
be a covariant (or contravariant) additive functor from an additive category  into an additive category
into an additive category 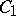 . Then for any object
. Then for any object  in
in 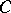 the functor
the functor  induces a natural homomorphism (or anti-homomorphism)
induces a natural homomorphism (or anti-homomorphism)  .
.
Let 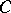 be the category of modules over a ring
be the category of modules over a ring 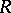 . For an
. For an  -module
-module  the ring
the ring 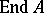 consists of all endomorphisms of the Abelian group
consists of all endomorphisms of the Abelian group  that commute with multiplication by elements of
that commute with multiplication by elements of  . The sum of two endomorphism
. The sum of two endomorphism 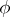 and
and  is defined by the formula
is defined by the formula
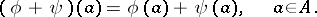 |
If  is commutative, then
is commutative, then  has the natural structure of an
has the natural structure of an  -algebra. Many properties of the module
-algebra. Many properties of the module  can be characterized in terms of
can be characterized in terms of  . For example,
. For example,  is an irreducible module if and only if
is an irreducible module if and only if  is a skew-field.
is a skew-field.
An arbitrary homomorphism 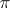 of an associative ring
of an associative ring  into
into  is called a representation of the ring
is called a representation of the ring  (by endomorphisms of the object
(by endomorphisms of the object  ). If
). If  has a unit element, then one imposes the additional condition
has a unit element, then one imposes the additional condition  . Any associative ring
. Any associative ring 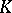 has a faithful representation in the endomorphism ring of a certain Abelian group
has a faithful representation in the endomorphism ring of a certain Abelian group  . If
. If  , moreover, has a unit element, then
, moreover, has a unit element, then  can be chosen as the additive group of
can be chosen as the additive group of  on which the elements of
on which the elements of 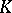 act by left multiplication. If
act by left multiplication. If  has no unit element and
has no unit element and  is obtained from
is obtained from 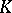 by adjoining a unit to
by adjoining a unit to  externally, then
externally, then  can be taken to be the additive group of
can be taken to be the additive group of  .
.
In the case of an Abelian variety  one considers, apart from the ring
one considers, apart from the ring 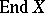 , which is a finitely-generated
, which is a finitely-generated  -module, the algebra of endomorphisms (algebra of complex multiplications)
-module, the algebra of endomorphisms (algebra of complex multiplications)  .
.
References
| [1] | C. Faith, "Algebra: rings, modules, and categories" , 1–2 , Springer (1973–1976) |
| [2] | D. Mumford, "Abelian varieties" , Oxford Univ. Press (1974) |
| [3] | V.T. Markov, A.V. Mikhalev, L.A. Skornyakov, A.A. Tugaubaev, "Endomorphism rings of modules and lattices of submodules" J. Soviet Math. , 31 : 3 (1985) pp. 3005–3051 Itogi Nauk. i Tekhn. Algebra. Topol. Geom. , 21 (1983) pp. 183–254 |
Endomorphism ring. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Endomorphism_ring&oldid=14709