Kummer criterion
From Encyclopedia of Mathematics
A general convergence criterion for series with positive terms, proposed by E. Kummer. Given a series
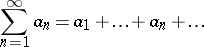 | (*) |
and an arbitrary sequence 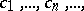 of positive numbers such that the series
of positive numbers such that the series 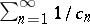 is divergent. If there exists an
is divergent. If there exists an 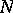 such that for
such that for 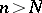 ,
,
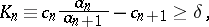 |
where 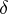 is a constant positive number, then the series (*) is convergent. If
is a constant positive number, then the series (*) is convergent. If 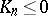 for
for 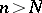 , the series (*) is divergent.
, the series (*) is divergent.
In terms of limits Kummer's criterion may be stated as follows. Let
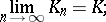 |
then the series (*) is convergent if 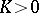 and divergent if
and divergent if 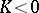 .
.
References
| [1] | G.M. Fichtenholz, "Differential und Integralrechnung" , 2 , Deutsch. Verlag Wissenschaft. (1964) |
Comments
References
| [a1] | E.D. Rainville, "Infinite series" , Macmillan (1967) |
How to Cite This Entry:
Kummer criterion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kummer_criterion&oldid=14698
Kummer criterion. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Kummer_criterion&oldid=14698
This article was adapted from an original article by E.G. Sobolevskaya (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article