Rational variety

An algebraic variety 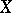, defined over an algebraically closed field 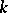, whose field of rational functions 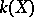is isomorphic to a purely transcendental extension of 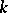of finite degree. In other words, a rational variety is an algebraic variety 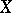that is birationally isomorphic to a projective space .

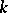A complete smooth rational variety possesses the following birational invariants. The dimensions of all spaces of regular differential -forms on are equal to 0. In addition, the multiple genus

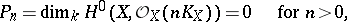where is the canonical divisor of the algebraic variety 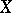, that is, the Kodaira dimension of the rational variety is equal to 0.

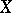In low dimension the above invariants uniquely distinguish the class of rational varieties among all algebraic varieties. Thus, if and the genus of is equal to 0, then is a rational curve. If 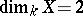, the arithmetic genus

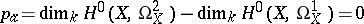and the multiple genus 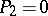, then 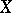is a rational surface. However, if 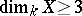, there is no good criterion of rationality, due to the negative solution of the Lüroth problem.

References

[1]	I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian)

References

[a1]	A. Beauville, J.-L. Colliot-Hélène, J.J. Sansuc, P. Swinnerton-Dyer, "Variétés stablement rationelles non-rationelles" Ann. of Math. , 121 (1985) pp. 283–318