Compatible distributions
projective system of probability measures, consistent system of probability measures, consistent system of distributions
A concept in probability theory and measure theory. For the most common and most important case of a product of spaces, see the article Measure. A more general construction is given below. Let 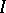 be an index set with a pre-order relation
be an index set with a pre-order relation  filtering to the right; suppose one is given a projective system of sets: For every
filtering to the right; suppose one is given a projective system of sets: For every 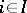 there is a set
there is a set 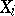 and for every pair of indices
and for every pair of indices 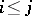 there is a mapping
there is a mapping 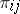 of
of 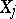 into
into 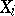 such that
such that 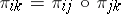 for
for 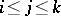 ; let
; let 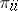 be the identity mapping on
be the identity mapping on 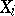 for every
for every 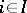 . It is further assumed that for each
. It is further assumed that for each 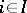 there is a
there is a  -algebra
-algebra 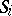 of subsets of
of subsets of 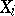 such that for
such that for  the mapping
the mapping 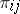 of
of 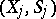 into
into 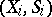 is measurable. Finally, let
is measurable. Finally, let 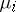 be a given distribution (or, more generally, a measure) on
be a given distribution (or, more generally, a measure) on 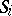 , for every
, for every 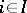 . The system of distributions (measures)
. The system of distributions (measures) 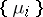 is called compatible (or consistent, or a projective system of distributions (measures)) if
is called compatible (or consistent, or a projective system of distributions (measures)) if  whenever
whenever 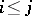 . Under certain additional conditions on the projective limit
. Under certain additional conditions on the projective limit  , there is a measure
, there is a measure 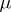 (the projective limit of the projective system
(the projective limit of the projective system  ) such that if
) such that if 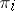 is the canonical projection of
is the canonical projection of 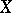 to
to  , then
, then  for all
for all 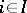 .
.
References
| [1] | A.N. Kolmogorov, "Foundations of the theory of probability" , Chelsea, reprint (1950) (Translated from Russian) |
| [2] | S. Bochner, "Harmonic analysis and the theory of probability" , Univ. California Press (1955) |
| [3] | M. Metivier, "Limites projectives de measures. Martingales. Applications" Ann. Mat. Pura Appl. , 63 (1963) pp. 225–352 |
| [4] | N. Bourbaki, "Elements of mathematics. Integration" , Addison-Wesley (1975) pp. Chapt.6;7;8 (Translated from French) |
Comments
A partial order or pre-order relation 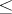 on
on 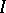 is said to filter to the right if for every
is said to filter to the right if for every  there is a
there is a 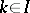 such that
such that 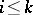 and
and 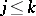 . The projective limit measure exists if, for instance, the
. The projective limit measure exists if, for instance, the 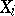 are all compact spaces, the
are all compact spaces, the 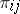 are all surjective and the family of norms
are all surjective and the family of norms 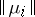 is bounded, where
is bounded, where 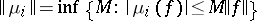 ,
,  ,
, 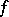 continuous of compact support. It also exists if the
continuous of compact support. It also exists if the 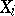 are compact,
are compact, 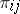 surjective, and the
surjective, and the 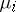 are positive measures; then
are positive measures; then 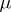 is positive and
is positive and 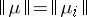 for all
for all  .
.
The concept of consistency (compatibility) of distributions (or measures) is of special importance in the construction of stochastic processes (cf. Stochastic process; Joint distribution).
Compatible distributions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Compatible_distributions&oldid=14474