Recursive relation
From Encyclopedia of Mathematics
A relation 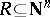 , where
, where 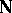 is the set of natural numbers, such that the function
is the set of natural numbers, such that the function 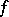 defined on
defined on 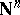 by the condition
by the condition
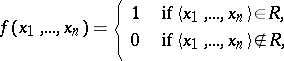 |
is a recursive function. In particular, for any  , the universal relation
, the universal relation 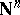 and the zero relation
and the zero relation 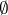 are recursive relations. If
are recursive relations. If 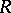 and
and 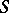 are
are  -place recursive relations, then the relations
-place recursive relations, then the relations 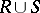 ,
, 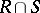 ,
, 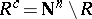 ,
, 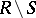 will also be recursive relations. With regard to the operations
will also be recursive relations. With regard to the operations  ,
, 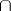 ,
,  , the system of all
, the system of all  -place recursive relations thus forms a Boolean algebra.
-place recursive relations thus forms a Boolean algebra.
How to Cite This Entry:
Recursive relation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Recursive_relation&oldid=14461
Recursive relation. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Recursive_relation&oldid=14461
This article was adapted from an original article by V.E. Plisko (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article