Diagonal product of mappings
 ,
, 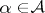
The mapping 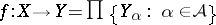 defined by the equation
defined by the equation 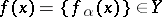 . The diagonal product of mappings
. The diagonal product of mappings  satisfies, for any
satisfies, for any 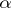 , the relation
, the relation  , where
, where 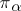 denotes the projection of the product
denotes the projection of the product 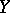 on the factor
on the factor  . The diagonal product of continuous mappings is continuous. A family of mappings
. The diagonal product of continuous mappings is continuous. A family of mappings 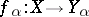 of topological spaces is said to be partitioning if for any point
of topological spaces is said to be partitioning if for any point  and neighbourhood
and neighbourhood 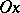 of
of  there exist an index
there exist an index 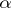 and an open subset
and an open subset  such that
such that  . If
. If 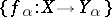 is a partitioning family of mappings and if
is a partitioning family of mappings and if  is the diagonal product of the mappings
is the diagonal product of the mappings 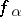 , then
, then 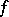 is an imbedding of
is an imbedding of 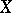 into the product
into the product 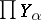 , i.e.
, i.e. 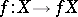 is a homeomorphism. The diagonal product of mappings was used by A.N. Tikhonov to imbed a completely-regular space of weight
is a homeomorphism. The diagonal product of mappings was used by A.N. Tikhonov to imbed a completely-regular space of weight  in the cube
in the cube 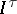 .
.
Comments
Instead of calling a family of mappings partitioning, one says that it separates points and closed sets.
In an arbitrary category with products, cf. Direct product, the diagonal product of mappings is given by the universal property defining the direct product. Indeed, categorically the product  is an object together with morphisms:
is an object together with morphisms: 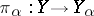 such that for every family of morphisms
such that for every family of morphisms 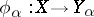 there is a unique morphism
there is a unique morphism 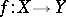 such that
such that  .
.
Tikhonov's imbedding result is in [a2]. E. Čech, inspired by Tikhonov's result, obtained the following imbedding theorem [a1]: Let  be the family of continuous mappings from a completely-regular space
be the family of continuous mappings from a completely-regular space  into the unit interval
into the unit interval 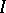 . Then the diagonal mapping
. Then the diagonal mapping  is an imbedding, and the closure of
is an imbedding, and the closure of 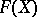 in
in  is equivalent to the Stone–Čech compactification of
is equivalent to the Stone–Čech compactification of 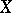 .
.
References
| [a1] | E. Čech, "On bicompact spaces" Ann. of Math. , 38 (1937) pp. 823–844 |
| [a2] | A.N. [A.N. Tikhonov] Tichonoff, "Ueber die topologische Erweiterung von Räumen" Math. Ann. , 102 (1929) pp. 544–561 |
Diagonal product of mappings. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Diagonal_product_of_mappings&oldid=14452