Dedekind completion

of a Riesz space

A Riesz space is called Dedekind complete if every non-empty subset that is bounded from below (respectively, above) has an infimum (respectively, supremum). A Dedekind-complete Riesz space is automatically Archimedean. Hence, so are its Riesz subspaces.

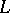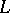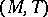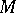Given an Archimedean Riesz space , a Dedekind completion of is a pair where is a Riesz space and is a mapping such that

1) 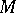is Dedekind complete;

2) 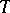is a Riesz isomorphism of 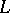onto a Riesz subspace of ;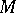

3) as a mapping 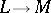, 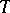is normal, i.e., it preserves arbitrary suprema and infima;

4) for all ,

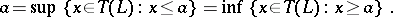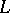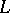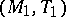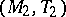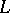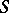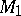Every Archimedean Riesz space has a Dedekind completion, whose underlying partially ordered set can be obtained from the MacNeille completion (cf. Completion, MacNeille (of a partially ordered set)) of by removing its largest and smallest elements. The Dedekind completion is unique in the following sense. If and are Dedekind completions of , then there exists a unique Riesz isomorphism of onto 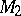with 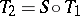. More generally, if 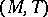is a Dedekind completion of , then every normal Riesz homomorphism of into any Dedekind-complete Riesz space 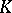can uniquely be extended to a normal Riesz homomorphism .

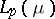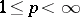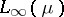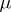The Riesz spaces () are Dedekind complete; so is if is 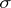-finite. The space 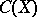( 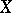a compact Hausdorff space) is Dedekind complete if and only if is extremally disconnected (cf. Extremally-disconnected space). There are few non-trivial instances of Riesz spaces whose Dedekind completions are to some extent "understood" . The Dedekind completion of the space of all converging sequences is 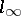. That of 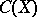is the quotient 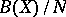, where 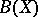is the space of all bounded Borel functions and 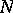is the ideal of all functions that vanish off meager sets (cf. Category of a set). (In either case, the mapping is obvious.)

References

[a1]	E. de Jonge, A. van Rooij, "Introduction to Riesz spaces" , Tracts , 8 , Math. Centre, Amsterdam (1977)
[a2]	W. Luxemburg, A.C. Zaanen, "Riesz spaces" , I , North-Holland (1974)
[a3]	B.Z. Vulikh, "Introduction to the theory of partially ordered spaces" , Wolters–Noordhoff (1967) (In Russian)